Serret-Frenet formulae
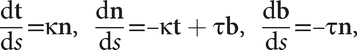
where τ denotes torsion. If the torsion of a curve is 0, then the curve is planar. κ(s) and τ(s) determine a curve up to isometry.
- tetrahedron
- TeX
- t-formulae
- Thales of Miletus
- theorem
- Theorema Egregium
- theorem proving
- there exists
- theta function
- Thom, René Frédéric
- Thomson, William
- three-body problem
- three-door problem
- thrust
- tie
- tiling
- time
- time dilation
- time series
- Tn
- Tonelli's theorem
- tonne
- topological data analysis
- topological group
- topologically distinguishable