inverse function
For a real function defined on an interval I, if f is strictly increasing on I or strictly decreasing on I, then f is one‐to‐one and so bijective onto its image.
When an inverse is required for a given function f, it may be necessary to restrict the domain and obtain instead the inverse function of this restriction of f. For example, suppose that f: R → R is defined by f(x) = x2−4x + 5. This function is not one‐to‐one but is strictly increasing for x≥2. Use f now to denote the function defined by f(x) = x2−4x + 5 with domain [2, ∞). The image is [1, ∞) and the function f [2,∞)→ [1,∞) has inverse f−1: [1,∞)→ [2,∞). A formula for f−1 can be found by setting y = x2−4x + 5 and, remembering that x∈[2,∞), obtaining 
So, with a change of notation, 
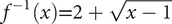for x≥1.
The graph of a function and its inverse
When the inverse function exists, the graphs y = f(x) and y = f−1(x) are reflections of each other in the line y = x. See inverse function theorem, inverse hyperbolic function, inverse trigonometric function, left inverse, right inverse.
- decimal representation
- decision analysis
- decision nodes
- decision problem
- decision theory
- decision tree
- decision variables
- deci‐
- declination
- decompose
- decomposition
- decreasing function
- decreasing sequence
- Dedekind cut
- Dedekind, (Julius Wilhelm) Richard
- deduction
- deficient number
- definite integral
- deformation
- degenerate
- degenerate conic
- degenerate quadric
- degree
- degree
- degree