| 释义 |
Pythagorean TheoremFor a Right Triangle with legs 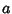 and and 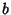 and Hypotenuse and Hypotenuse 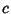 , ,
 | (1) |
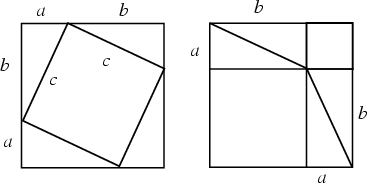
A clever proof by Dissection which reassembles two small squares into one larger one was given by the Arabianmathematician Thabit Ibn Qurra  (Ogilvy 1994, Frederickson 1997). (Ogilvy 1994, Frederickson 1997). Another proof by Dissection is due to Perigal (Pergial 1873, Dudeney 1970, Madachy 1979, Ball and Coxeter 1987). The Indian mathematician Bhaskara constructed a proof using the following figure. Another beautiful dissection proof is shown below.
 | (2) |
 | (3) |
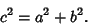 | (4) |
In the above figure, the Area of the large Square is four times the Area of one of theTriangles plus the Area of the interior Square. From the figure, 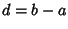 , so , so
Perhaps the most famous proof of all times is Euclid's  geometric proof. Euclid's proof used the figurebelow, which is sometimes known variously as the Bride's Chair, Peacock's Tail, or Windmill. geometric proof. Euclid's proof used the figurebelow, which is sometimes known variously as the Bride's Chair, Peacock's Tail, or Windmill. Let 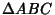 be a Right Triangle, be a Right Triangle,  , , 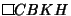 , and , and 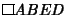 be squares, and be squares, and  . TheTriangles . TheTriangles 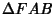 and and  are equivalent except for rotation, so are equivalent except for rotation, so
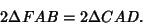 | (6) |
 | (7) |
 | (8) |
 | (9) |
 | (10) |
 proved that proved that  , ,  , and , and 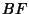 intersect in a point (Dunham 1990, pp. 48-53). intersect in a point (Dunham 1990, pp. 48-53).
Heron's Formula for the Area of the Triangle, contains the Pythagorean theorem implicitly. Using theform
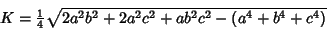 | (11) |
 | (12) |
 | (13) |
 | (14) |
 is the Area of a Triangle with sides is the Area of a Triangle with sides 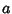 , , 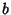 , and , and 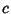 (Dunham 1990, pp. 128-129). (Dunham 1990, pp. 128-129).
A novel proof using a Trapezoid was discovered by James Garfield (1876), later president of the United States,while serving in the House of Representatives (Pappas 1989, pp. 200-201; Bogomolny).
Rearranging,
 | (16) |
 | (17) |
 | (18) |
An algebraic proof (which would not have been accepted by the Greeks) uses the Euler Formula. Let the sides of aTriangle be 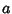 , , 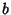 , and , and 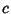 , and the Perpendicular legs of Right Triangle be aligned along the realand imaginary axes. Then , and the Perpendicular legs of Right Triangle be aligned along the realand imaginary axes. Then
 | (19) |
 | (20) |
 | (21) |
It is a property of Right Triangles, such as the one shown in the above left figure, that theRight Triangle with sides  , , 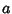 , and , and 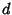 (small triangle in the left figure; reproduced in the right figure) issimilar to the Right Triangle with sides (small triangle in the left figure; reproduced in the right figure) issimilar to the Right Triangle with sides 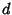 , , 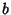 , and , and  (large triangle in the left figure; reproduced in the middlefigure), giving (large triangle in the left figure; reproduced in the middlefigure), giving
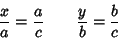 | (22) |
 | (23) |
 | (24) |
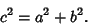 | (25) |

See also Bride's Chair, Cosines Law, Peacock's Tail, Pythagoras's Theorem, Windmill
References
Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, pp. 87-88, 1987.Bogomolny, A. ``Pythagorean Theorem.'' http://www.cut-the-knot.com/pythagoras/index.html. Dixon, R. ``The Theorem of Pythagoras.'' §4.1 in Mathographics. New York: Dover, pp. 92-95, 1991. Dudeney, H. E. Amusements in Mathematics. New York: Dover, p. 32, 1958. Dunham, W. ``Euclid's Proof of the Pythagorean Theorem.'' Ch. 2 in Journey Through Genius: The Great Theorems of Mathematics. New York: Wiley, 1990. Frederickson, G. Dissections: Plane and Fancy. New York: Cambridge University Press, pp. 28-29, 1997. Garfield, J. A. ``Pons Asinorum.'' New England J. Educ. 3, 161, 1876. Loomis, E. S. The Pythagorean Proposition: Its Demonstration Analyzed and Classified and Bibliography of Sources for Data of the Four Kinds of ``Proofs.'' Reston, VA: National Council of Teachers of Mathematics, 1968. Machover, M. ``Euler's Theorem Implies the Pythagorean Proposition.'' Amer. Math. Monthly 103, 351, 1996. Madachy, J. S. Madachy's Mathematical Recreations. New York: Dover, p. 17, 1979. Ogilvy, C. S. Excursions in Mathematics. New York: Dover, p. 52, 1994. Pappas, T. ``The Pythagorean Theorem,'' ``A Twist to the Pythagorean Theorem,'' and ``The Pythagorean Theorem and President Garfield.'' The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, pp. 4, 30, and 200-201, 1989. Perigal, H. ``On Geometric Dissections and Transformations.'' Messenger Math. 2, 103-106, 1873. Project Mathematics! The Theorem of Pythagoras. Videotape (22 minutes). California Institute of Technology. Available from the Math. Assoc. Amer. Shanks, D. Solved and Unsolved Problems in Number Theory, 4th ed. New York: Chelsea, pp. 123-127, 1993. Yancey, B. F. and Calderhead, J. A. ``New and Old Proofs of the Pythagorean Theorem.'' Amer. Math. Monthly 3, 65-67, 110-113, 169-171, and 299-300, 1896. Yancey, B. F. and Calderhead, J. A. ``New and Old Proofs of the Pythagorean Theorem.'' Amer. Math. Monthly 4, 11-12, 79-81, 168-170, 250-251, and 267-269, 1897. Yancey, B. F. and Calderhead, J. A. ``New and Old Proofs of the Pythagorean Theorem.'' Amer. Math. Monthly 5, 73-74, 1898. Yancey, B. F. and Calderhead, J. A. ``New and Old Proofs of the Pythagorean Theorem.'' Amer. Math. Monthly 6, 33-34 and 69-71, 1899.
|
![]() and
and ![]() and Hypotenuse
and Hypotenuse ![]() ,
,![]() (Ogilvy 1994, Frederickson 1997).
(Ogilvy 1994, Frederickson 1997).



![]() , so
, so![]() geometric proof. Euclid's proof used the figurebelow, which is sometimes known variously as the Bride's Chair, Peacock's Tail, or Windmill.
geometric proof. Euclid's proof used the figurebelow, which is sometimes known variously as the Bride's Chair, Peacock's Tail, or Windmill.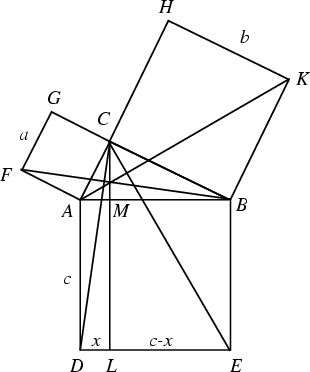
![]() be a Right Triangle,
be a Right Triangle, ![]() ,
, ![]() , and
, and ![]() be squares, and
be squares, and ![]() . TheTriangles
. TheTriangles ![]() and
and ![]() are equivalent except for rotation, so
are equivalent except for rotation, so
![]() ,
, ![]() , and
, and ![]() , and the Perpendicular legs of Right Triangle be aligned along the realand imaginary axes. Then
, and the Perpendicular legs of Right Triangle be aligned along the realand imaginary axes. Then
![]() ,
, ![]() , and
, and ![]() (small triangle in the left figure; reproduced in the right figure) issimilar to the Right Triangle with sides
(small triangle in the left figure; reproduced in the right figure) issimilar to the Right Triangle with sides ![]() ,
, ![]() , and
, and ![]() (large triangle in the left figure; reproduced in the middlefigure), giving
(large triangle in the left figure; reproduced in the middlefigure), giving