| 释义 |
Alexander PolynomialA Polynomial invariant of a Knot discovered in 1923 by J. W. Alexander (Alexander 1928). In technicallanguage, the Alexander polynomial arises from the Homology of the infinitely cyclic coverof a Knot's complement. Any generator of a Principal Alexander Ideal is called anAlexander polynomial (Rolfsen 1976). Because the Alexander Invariant of a Tame Knot in  has aSquare presentation Matrix, its Alexander Ideal is Principal and it has an Alexander polynomial denoted has aSquare presentation Matrix, its Alexander Ideal is Principal and it has an Alexander polynomial denoted  . .
Let 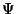 be the Matrix Product of Braid Words of a Knot, then be the Matrix Product of Braid Words of a Knot, then
 | (1) |
 is the Alexander polynomial and det is the Determinant. The Alexander polynomial of a TameKnot in is the Alexander polynomial and det is the Determinant. The Alexander polynomial of a TameKnot in  satisfies satisfies
 | (2) |
 is a Seifert Matrix, det is the Determinant, and is a Seifert Matrix, det is the Determinant, and  denotes the Matrix Transpose. The Alexander polynomial also satisfies denotes the Matrix Transpose. The Alexander polynomial also satisfies
 | (3) |
 Pretzel Knot. Pretzel Knot.
The Alexander polynomial remained the only known Knot Polynomial until the Jones Polynomial wasdiscovered in 1984. Unlike the Alexander polynomial, the more powerful Jones Polynomial does, in most cases,distinguish Handedness. A normalized form of the Alexander polynomial symmetric in 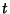 and and  andsatisfying andsatisfying
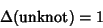 | (4) |
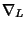 . The Notation . The Notation  is anabbreviation for the Conway-normalized Alexander polynomial of a Knot is anabbreviation for the Conway-normalized Alexander polynomial of a Knot
 | (5) |
for the Trefoil Knot, Figure-of-Eight Knot, and Solomon's Seal Knot, respectively. Multiplyingthrough to clear the Negative Powers gives the usual Alexander polynomial, where the finalSign is determined by convention.
Let an Alexander polynomial be denoted 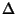 , then there exists a Skein Relationship(discovered by J. H. Conway) , then there exists a Skein Relationship(discovered by J. H. Conway)
 | (9) |
 | (10) |
For a Knot,
 | (11) |
 is a Knot and is a Knot and
 | (12) |
 cannot be represented as a closed 3-Braid. Also, if cannot be represented as a closed 3-Braid. Also, if
 | (13) |
 cannot be represented as a closed 4-braid (Jones 1985). cannot be represented as a closed 4-braid (Jones 1985).
The HOMFLY Polynomial 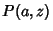 generalizes the Alexander polynomial (as well at the Jones Polynomial)with generalizes the Alexander polynomial (as well at the Jones Polynomial)with
 | (14) |
Rolfsen (1976) gives a tabulation of Alexander polynomials for Knots up to 10Crossings and Links up to 9 Crossings. See also Braid Group, Jones Polynomial, Knot, Knot Determinant,Link, Skein Relationship
ReferencesAdams, C. C. The Knot Book: An Elementary Introduction to the Mathematical Theory of Knots. New York: W. H. Freeman, pp. 165-169, 1994.Alexander, J. W. ``Topological Invariants of Knots and Links.'' Trans. Amer. Math. Soc. 30, 275-306, 1928. Alexander, J. W. ``A Lemma on a System of Knotted Curves." Proc. Nat. Acad. Sci. USA 9, 93-95, 1923. Doll, H. and Hoste, J. ``A Tabulation of Oriented Links.'' Math. Comput. 57, 747-761, 1991. Jones, V. ``A Polynomial Invariant for Knots via von Neumann Algebras.'' Bull. Amer. Math. Soc. 12, 103-111, 1985. Rolfsen, D. ``Table of Knots and Links.'' Appendix C in Knots and Links. Wilmington, DE: Publish or Perish Press, pp. 280-287, 1976. Stoimenow, A. ``Alexander Polynomials.'' http://www.informatik.hu-berlin.de/~stoimeno/ptab/a10.html. Stoimenow, A. ``Conway Polynomials.'' http://www.informatik.hu-berlin.de/~stoimeno/ptab/c10.html. |
![]() has aSquare presentation Matrix, its Alexander Ideal is Principal and it has an Alexander polynomial denoted
has aSquare presentation Matrix, its Alexander Ideal is Principal and it has an Alexander polynomial denoted ![]() .
.![]() be the Matrix Product of Braid Words of a Knot, then
be the Matrix Product of Braid Words of a Knot, then![]() and
and ![]() andsatisfying
andsatisfying
![]() , then there exists a Skein Relationship(discovered by J. H. Conway)
, then there exists a Skein Relationship(discovered by J. H. Conway)![]() generalizes the Alexander polynomial (as well at the Jones Polynomial)with
generalizes the Alexander polynomial (as well at the Jones Polynomial)with