Aliquot Sequence
Let
where
is the Divisor Function and 
is the Restricted Divisor Function. Then theSequence of numbers
is called an aliquot sequence. If the Sequence for a given
is bounded, it either ends at 
or becomesperiodic.- 1. If the Sequence reaches a constant, the constant is known as a Perfect Number.
- 2. If the Sequence reaches an alternating pair, it is called an Amicable Pair.
- 3. If, after
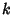iterations, the Sequence yields a cycle of minimum length 
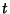of the form 
,
, ..., 
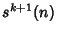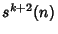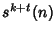, then these numbers form a group of Sociable Numbers of order .
(Guy 1994).See also 196-Algorithm, Additive Persistence, Amicable Numbers, Multiamicable Numbers,Multiperfect Number, Multiplicative Persistence, Perfect Number, Sociable Numbers, UnitaryAliquot Sequence
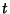References
Guy, R. K. ``Aliquot Sequences.'' §B6 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 60-62, 1994. Guy, R. K. and Selfridge, J. L. ``What Drives Aliquot Sequences.'' Math. Comput. 29, 101-107, 1975. Sloane, N. J. A. SequenceA003023/M0062in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and extended entry inSloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
- Genus (Curve)
- Genus (Knot)
- Genus (Surface)
- Genus Theorem
- Geocentric Latitude
- Geodesic
- Geodesic Curvature
- Geodesic Dome
- Geodesic Equation
- Geodesic Flow
- Geodesic Triangle
- Geodetic Latitude
- Geographic Latitude
- Geometric Construction
- Geometric Distribution
- Geometric Mean
- Geometric Mean Index
- Geometric Probability Constants
- Geometric Problems of Antiquity
- Geometric Progression
- Geometric Sequence
- Geometric Series
- Geometrization Conjecture
- Geometrography
- Geometry