| 释义 |
Bessel Function of the First KindThe Bessel functions of the first kind  are defined as the solutions to the Bessel Differential Equation are defined as the solutions to the Bessel Differential Equation
 | (1) |
 for for 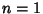 , 2, ..., 5. , 2, ..., 5.
To solve the differential equation, apply Frobenius Method using a series solution of the form
 | (2) |
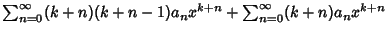 | |  | (4) |
The Indicial Equation, obtained by setting  , is , is
 | (5) |
 is defined as the first Nonzero term, is defined as the first Nonzero term,  , so , so  . Now, if . Now, if  , ,
 | (6) |
 | (7) |
 | (8) |
 | (9) |
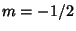 , then (9) becomes , then (9) becomes
 | (10) |
 | (11) |
 , where , where 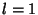 , 2, .... , 2, ....
which, using the identity  , gives , gives
 | (13) |

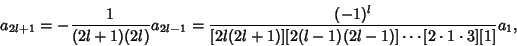 | (14) |
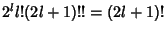 , gives , gives
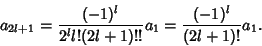 | (15) |
 gives gives
The Bessel Functions of order  are therefore defined as are therefore defined as
so the general solution for  is is
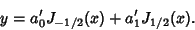 | (19) |
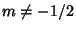 . Equation (9) requires . Equation (9) requires
 | (20) |
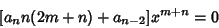 | (21) |
 , 3, ..., so , 3, ..., so
for  , 3, .... Let , 3, .... Let  , where , where 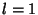 , 2, ..., then , 2, ..., then
where  is the function of is the function of  and and  obtained by iterating the recursion relationship down to obtained by iterating the recursion relationship down to 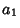 . Now let . Now let  , where , where 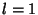 , 2, ..., so , 2, ..., so
Plugging back into (9),
Now define
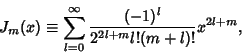 | (27) |
 . Theabove equation then becomes . Theabove equation then becomes
 | (28) |
 , ,
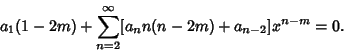 | (29) |
 is arbitrary, so the solutions must be the same for is arbitrary, so the solutions must be the same for  and and  . We are therefore free toreplace . We are therefore free toreplace  with with  , so , so
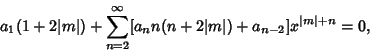 | (30) |
 replaced by replaced by  . .
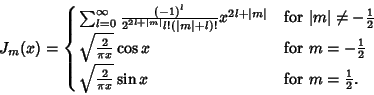 | (31) |
 and and 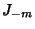 (when (when  is an Integer) by writing is an Integer) by writing
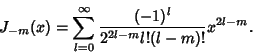 | (32) |
 . Then . Then
But  for for  , so the Denominator is infinite and the terms on the right are zero. Wetherefore have , so the Denominator is infinite and the terms on the right are zero. Wetherefore have
 | (34) |
Note that the Bessel Differential Equation is second-order, so there must be two linearly independent solutions.We have found both only for  . For a general nonintegral order, the independent solutions are . For a general nonintegral order, the independent solutions are  and and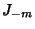 . When . When  is an Integer, the general (real) solution is of the form is an Integer, the general (real) solution is of the form
 | (35) |
 is a Bessel function of the first kind, is a Bessel function of the first kind,  (a.k.a. (a.k.a.  ) is the Bessel Function of the Second Kind(a.k.a. Neumann Function or Weber Function), and ) is the Bessel Function of the Second Kind(a.k.a. Neumann Function or Weber Function), and 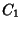 and and  are constants. Complexsolutions are given by the Hankel Functions (a.k.a. Bessel Functions of the ThirdKind). are constants. Complexsolutions are given by the Hankel Functions (a.k.a. Bessel Functions of the ThirdKind).
The Bessel functions are Orthogonal in 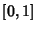 with respect to the weight factor with respect to the weight factor  . Exceptwhen . Exceptwhen  is a Negative Integer, is a Negative Integer,
 | (36) |
 is the Gamma Function and is the Gamma Function and  is a Whittaker Function. is a Whittaker Function.
In terms of a Confluent Hypergeometric Function of the First Kind, the Bessel function is written
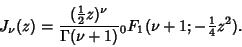 | (37) |
 is is
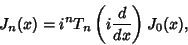 | (38) |
 is a Chebyshev Polynomial of the First Kind. Asymptotic forms for the Bessel functions are is a Chebyshev Polynomial of the First Kind. Asymptotic forms for the Bessel functions are
 | (39) |
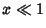 and and
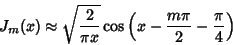 | (40) |
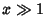 . A derivative identity is . A derivative identity is
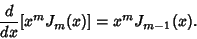 | (41) |
 | (42) |
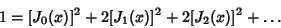 | (43) |
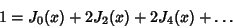 | (44) |
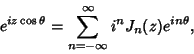 | (45) |
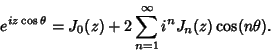 | (46) |
 | (47) |
Roots of the Function  are given in the following table. are given in the following table. | zero |  |  | 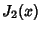 |  |  |  | | 1 | 2.4048 | 3.8317 | 5.1336 | 6.3802 | 7.5883 | 8.7715 | | 2 | 5.5201 | 7.0156 | 8.4172 | 9.7610 | 11.0647 | 12.3386 | | 3 | 8.6537 | 10.1735 | 11.6198 | 13.0152 | 14.3725 | 15.7002 | | 4 | 11.7915 | 13.3237 | 14.7960 | 16.2235 | 17.6160 | 18.9801 | | 5 | 14.9309 | 16.4706 | 17.9598 | 19.4094 | 20.8269 | 22.2178 |
Let  be the be the 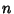 th Root of the Bessel function th Root of the Bessel function  , then , then
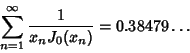 | (48) |
The Roots of its Derivatives are given in the following table. | zero | 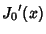 | 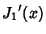 |  |  | 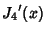 | 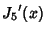 | | 1 | 3.8317 | 1.8412 | 3.0542 | 4.2012 | 5.3175 | 6.4156 | | 2 | 7.0156 | 5.3314 | 6.7061 | 8.0152 | 9.2824 | 10.5199 | | 3 | 10.1735 | 8.5363 | 9.9695 | 11.3459 | 12.6819 | 13.9872 | | 4 | 13.3237 | 11.7060 | 13.1704 | 14.5858 | 15.9641 | 17.3128 | | 5 | 16.4706 | 14.8636 | 16.3475 | 17.7887 | 19.1960 | 20.5755 |
Various integrals can be expressed in terms of Bessel functions
which is Bessel's First Integral,
for 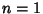 , 2, ..., , 2, ...,
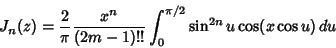 | (53) |
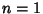 , 2, ..., , 2, ...,
for  . Integrals involving . Integrals involving  include include
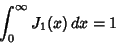 | (55) |
 | (56) |
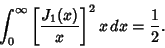 | (57) |
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Bessel Functions  and and 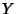 .'' §9.1 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 358-364, 1972. .'' §9.1 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 358-364, 1972.Arfken, G. ``Bessel Functions of the First Kind,  '' and ``Orthogonality.'' §11.1 and 11.2 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 573-591 and 591-596, 1985. '' and ``Orthogonality.'' §11.1 and 11.2 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 573-591 and 591-596, 1985. Lehmer, D. H. ``Arithmetical Periodicities of Bessel Functions.'' Ann. Math. 33, 143-150, 1932. Le Lionnais, F. Les nombres remarquables. Paris: Hermann, p. 25, 1983. Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 619-622, 1953. Spanier, J. and Oldham, K. B. ``The Bessel Coefficients  and and  '' and ``The Bessel Function '' and ``The Bessel Function  .'' Chs. 52-53 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 509-520 and 521-532, 1987. .'' Chs. 52-53 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 509-520 and 521-532, 1987.
|

![]() are defined as the solutions to the Bessel Differential Equation
are defined as the solutions to the Bessel Differential Equation 






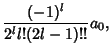




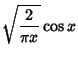
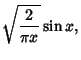

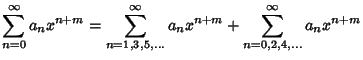

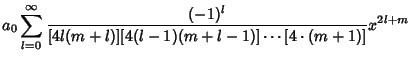


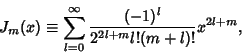
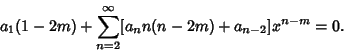
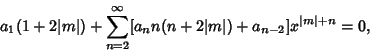
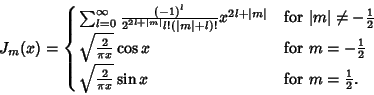
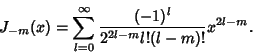

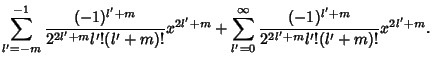

![]() . For a general nonintegral order, the independent solutions are
. For a general nonintegral order, the independent solutions are ![]() and
and![]() . When
. When ![]() is an Integer, the general (real) solution is of the form
is an Integer, the general (real) solution is of the form![]() with respect to the weight factor
with respect to the weight factor ![]() . Exceptwhen
. Exceptwhen ![]() is a Negative Integer,
is a Negative Integer,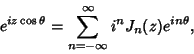
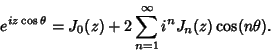

![]() are given in the following table.
are given in the following table.![]() be the
be the ![]() th Root of the Bessel function
th Root of the Bessel function ![]() , then
, then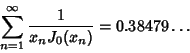
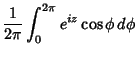




![]() and
and ![]() .'' §9.1 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 358-364, 1972.
.'' §9.1 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 358-364, 1972.![]() '' and ``Orthogonality.'' §11.1 and 11.2 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 573-591 and 591-596, 1985.
'' and ``Orthogonality.'' §11.1 and 11.2 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 573-591 and 591-596, 1985.![]() and
and ![]() '' and ``The Bessel Function
'' and ``The Bessel Function ![]() .'' Chs. 52-53 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 509-520 and 521-532, 1987.
.'' Chs. 52-53 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 509-520 and 521-532, 1987.