| 释义 |
Binomial DistributionThe probability of 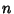 successes in successes in  Bernoulli Trials is Bernoulli Trials is
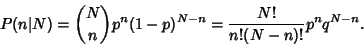 | (1) |
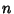 observed is observed is
 | (2) |
 | (3) |
 is the Beta Function, and is the Beta Function, and 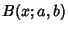 is the incomplete Beta Function. The CharacteristicFunction is is the incomplete Beta Function. The CharacteristicFunction is
 | (4) |
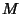 for the distribution is for the distribution is
The Mean is
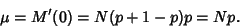 | (8) |
so the Moments about the Mean are
The Skewness and Kurtosis are
An approximation to the Bernoulli distribution for large  can be obtained by expanding about the value can be obtained by expanding about the value  where where is a maximum, i.e., where is a maximum, i.e., where  . Since the Logarithm function is Monotonic, we can instead choose to expand the Logarithm. Let . Since the Logarithm function is Monotonic, we can instead choose to expand the Logarithm. Let  , then , then
 | (18) |
 | (19) |
 | (20) |
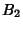 is negative, so we can write is negative, so we can write 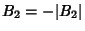 . Now, taking the Logarithm of (1) gives . Now, taking the Logarithm of (1) gives
 | (21) |
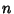 and and  we can use Stirling's Approximation we can use Stirling's Approximation
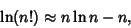 | (22) |
and
 | (25) |
 , set this expression to 0 and solve for , set this expression to 0 and solve for 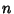 , ,
 | (26) |
 | (27) |
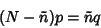 | (28) |
 | (29) |
 . We can now find the terms in the expansion . We can now find the terms in the expansion
Now, treating the distribution as continuous,
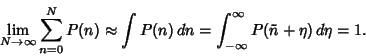 | (33) |
 smaller than the previous, we can ignore terms higher than smaller than the previous, we can ignore terms higher than 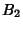 , so , so
 | (34) |
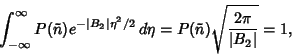 | (35) |
Defining  , ,
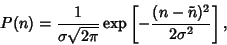 | (37) |
 , a different approximation procedure shows that the binomialdistribution approaches the Poisson Distribution. The first Cumulant is , a different approximation procedure shows that the binomialdistribution approaches the Poisson Distribution. The first Cumulant is
 | (38) |
 | (39) |
Let  and and  be independent binomial Random Variables characterized by parameters be independent binomial Random Variables characterized by parameters  and and  . The Conditional Probability of . The Conditional Probability of  given that given that 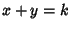 is is  | | 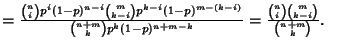 | (40) |
Note that this is a Hypergeometric Distribution!See also de Moivre-Laplace Theorem, Hypergeometric Distribution, Negative Binomial Distribution
References
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 531, 1987.Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. ``Incomplete Beta Function, Student's Distribution, F-Distribution, Cumulative Binomial Distribution.'' §6.2 in Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 219-223, 1992. Spiegel, M. R. Theory and Problems of Probability and Statistics. New York: McGraw-Hill, p. 108-109, 1992.
|

![]() successes in
successes in ![]() Bernoulli Trials is
Bernoulli Trials is 
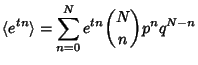


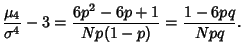

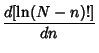

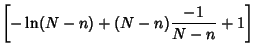

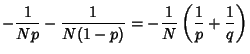



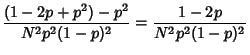

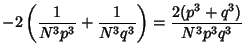



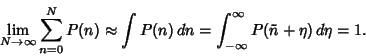
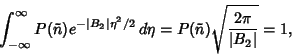
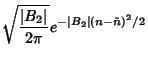

![]() and
and ![]() be independent binomial Random Variables characterized by parameters
be independent binomial Random Variables characterized by parameters ![]() and
and ![]() . The Conditional Probability of
. The Conditional Probability of ![]() given that
given that ![]() is
is