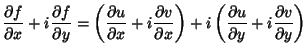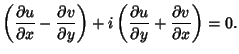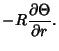| 释义 |
Cauchy-Riemann EquationsLet
 | (1) |
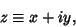 | (2) |
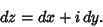 | (3) |
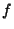 with respect to with respect to  may then be computed as follows. may then be computed as follows.
so
and
 | (8) |
 and and 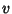 , (8) becomes , (8) becomes
Along the real, or x-Axis,  , so , so
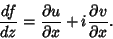 | (10) |
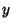 -axis, -axis,  , so , so
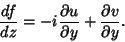 | (11) |
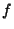 is Complex Differentiable, then the value of the derivative must be the same for a given is Complex Differentiable, then the value of the derivative must be the same for a given  , regardless of itsorientation. Therefore, (10) must equal (11), which requires that , regardless of itsorientation. Therefore, (10) must equal (11), which requires that
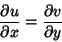 | (12) |
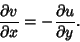 | (13) |
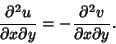 | (14) |
In Polar Coordinates,
 | (16) |
If  and and 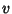 satisfy the Cauchy-Riemann equations, they also satisfyLaplace's Equation in 2-D, since satisfy the Cauchy-Riemann equations, they also satisfyLaplace's Equation in 2-D, since
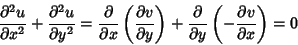 | (19) |
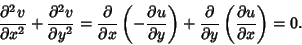 | (20) |
 , solutions can be found which automatically satisfy the Cauchy-Riemann equations andLaplace's Equation. This fact is used to find so-called Conformal Solutions tophysical problems involving scalar potentials such as fluid flow and electrostatics.See also Cauchy Integral Theorem, Conformal Solution, Monogenic Function,Polygenic Function , solutions can be found which automatically satisfy the Cauchy-Riemann equations andLaplace's Equation. This fact is used to find so-called Conformal Solutions tophysical problems involving scalar potentials such as fluid flow and electrostatics.See also Cauchy Integral Theorem, Conformal Solution, Monogenic Function,Polygenic Function
References
Abramowitz, M. and Stegun, C. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 17, 1972.Arfken, G. ``Cauchy-Riemann Conditions.'' §6.2 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 3560-365, 1985.
|