Abelian Group
N.B. A detailed on-line essay by S. Finchwas the starting point for this entry.
A Group for which the elements Commute (i.e., ![]() for all elements
for all elements ![]() and
and ![]() ) iscalled an Abelian group. All Cyclic Groups are Abelian, but an Abelian group is not necessarilyCyclic. All Subgroups of anAbelian group are Normal. In an Abelian group, each element is in a Conjugacy Class byitself, and the Character Table involves Powers of a single element known as aGenerator.
) iscalled an Abelian group. All Cyclic Groups are Abelian, but an Abelian group is not necessarilyCyclic. All Subgroups of anAbelian group are Normal. In an Abelian group, each element is in a Conjugacy Class byitself, and the Character Table involves Powers of a single element known as aGenerator.
No general formula is known for giving the number of nonisomorphic Finite Groups of a givenOrder. However, the number of nonisomorphic Abelian Finite Groups ![]() ofany given Order
ofany given Order ![]() is given by writing
is given by writing ![]() as
as
| (1) |
| (2) |
The Kronecker Decomposition Theorem states that every Finite Abelian group can be written as a DirectProduct of Cyclic Groups of Prime Power Orders. If the Orders of a Finite Group is a Prime ![]() , then there exists a singleAbelian group of order
, then there exists a singleAbelian group of order ![]() (denoted
(denoted ![]() ) and no non-Abelian groups. If the Order is a prime squared
) and no non-Abelian groups. If the Order is a prime squared![]() , then there are two Abelian groups (denoted
, then there are two Abelian groups (denoted ![]() and
and ![]() . If the Order is aprime cubed
. If the Order is aprime cubed ![]() , then there are three Abelian groups (denoted
, then there are three Abelian groups (denoted ![]() ,
, ![]() , and
, and![]() ), and five groups total. If the Order is a Product of two primes
), and five groups total. If the Order is a Product of two primes ![]() and
and ![]() , thenthere exists exactly one Abelian group of order
, thenthere exists exactly one Abelian group of order ![]() (denoted
(denoted ![]() ).
).
Another interesting result is that if ![]() denotes the number of nonisomorphic Abelian groups of Order
denotes the number of nonisomorphic Abelian groups of Order ![]() , then
, then
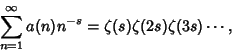 | (3) |
 | (4) |
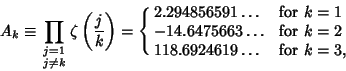 | (5) |
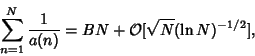 | (6) |
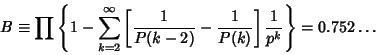 | (7) |
References
DeKoninck, J.-M. and Ivic, A. Topics in Arithmetical Functions: Asymptotic Formulae for Sums of Reciprocals of Arithmetical Functions and Related Fields. Amsterdam, Netherlands: North-Holland, 1980. Erdös, P. and Szekeres, G. ``Über die Anzahl abelscher Gruppen gegebener Ordnung und über ein verwandtes zahlentheoretisches Problem.'' Acta Sci. Math. (Szeged) 7, 95-102, 1935. Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/abel/abel.html Kendall, D. G. and Rankin, R. A. ``On the Number of Abelian Groups of a Given Order.'' Quart. J. Oxford 18, 197-208, 1947. Kolesnik, G. ``On the Number of Abelian Groups of a Given Order.'' J. Reine Angew. Math. 329, 164-175, 1981. Neumann, P. M. ``An Enumeration Theorem for Finite Groups.'' Quart. J. Math. Ser. 2 20, 395-401, 1969. Pyber, L. ``Enumerating Finite Groups of Given Order.'' Ann. Math. 137, 203-220, 1993. Richert, H.-E. ``Über die Anzahl abelscher Gruppen gegebener Ordnung I.'' Math. Zeitschr. 56, 21-32, 1952. Sloane, N. J. A. SequenceA000688/M0064in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995. Srinivasan, B. R. ``On the Number of Abelian Groups of a Given Order.'' Acta Arith. 23, 195-205, 1973.
- Area Principle
- Arf Invariant
- Argand Diagram
- Argand Plane
- Argoh's Conjecture
- Argument Addition Relation
- Argument (Complex Number)
- Argument (Elliptic Integral)
- Argument (Function)
- Argument Multiplication Relation
- Argument Principle
- Argument Variation
- Aristotle's Wheel Paradox
- Arithmetic
- Arithmetic-Geometric Mean
- Arithmetic Geometry
- Arithmetic-Harmonic Mean
- Arithmetic-Logarithmic-Geometric Mean Inequality
- Arithmetic Mean
- Arithmetic Progression
- Arithmetic Sequence
- Arithmetic Series
- Armstrong Number
- Arnold Diffusion
- Arnold's Cat Map