| 释义 |
Continued FractionA ``general'' continued fraction representation of a Real Number  is of the form is of the form
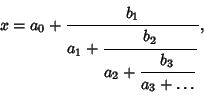 | (1) |
 | (2) |
 (which is usually what is meant when the term ``continuedfraction'' is used without qualification) of a number is given by (which is usually what is meant when the term ``continuedfraction'' is used without qualification) of a number is given by
 | (3) |
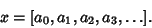 | (4) |
 | (5) |
 (where (where  is the Floor Function), is the Floor Function),
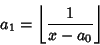 | (6) |
 , , 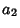 is the integral part of the reciprocal of theremainder, etc. The quantities is the integral part of the reciprocal of theremainder, etc. The quantities  are called Partial Quotients. An archaic word for acontinued fraction is Anthyphairetic Ratio. are called Partial Quotients. An archaic word for acontinued fraction is Anthyphairetic Ratio.
Continued fractions provide, in some sense, a series of ``best'' estimates for an Irrational Number. Functions canalso be written as continued fractions, providing a series of better and better rational approximations. Continued fractionshave also proved useful in the proof of certain properties of numbers such as e and  (Pi). Becauseirrationals which are square roots of Rational Numbers have periodic continued fractions, an exactrepresentation for a tabulated numerical value (i.e., 1.414... for Pythagoras's Constant, (Pi). Becauseirrationals which are square roots of Rational Numbers have periodic continued fractions, an exactrepresentation for a tabulated numerical value (i.e., 1.414... for Pythagoras's Constant,  ) cansometimes be found. ) cansometimes be found.
Continued fractions are also useful for finding near commensurabilities between events with different periods. For example,the Metonic cycle  used for calendrical purposes by the Greeks consists of 235 lunar monthswhich very nearly equal 19 solar years, and 235/19 is the sixth Convergent of the ratio of the lunar phase (synodic)period and solar period (365.2425/29.53059). Continued fractions can also be used to calculate gear ratios, and were usedfor this purpose by the ancient Greeks (Guy 1990). used for calendrical purposes by the Greeks consists of 235 lunar monthswhich very nearly equal 19 solar years, and 235/19 is the sixth Convergent of the ratio of the lunar phase (synodic)period and solar period (365.2425/29.53059). Continued fractions can also be used to calculate gear ratios, and were usedfor this purpose by the ancient Greeks (Guy 1990).
If only the first few terms of a continued fraction are kept, the result is called a Convergent. Let  beconvergents of a nonsimple continued fraction. Then beconvergents of a nonsimple continued fraction. Then
 | (7) |
 | (8) |
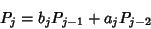 | (9) |
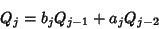 | (10) |
 , 2, ..., , 2, ...,  . It is also true that . It is also true that
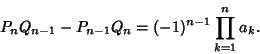 | (11) |
A finite simple continued fraction representation terminates after a finite number of terms. To ``round'' acontinued fraction, truncate the last term unless it is 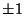 , in which case it should be added to the previous term(Beeler et al. 1972, Item 101A). To take one over a continued fraction, add (or possibly delete) an initial 0 term. Tonegate, take the Negative of all terms, optionally using the identity , in which case it should be added to the previous term(Beeler et al. 1972, Item 101A). To take one over a continued fraction, add (or possibly delete) an initial 0 term. Tonegate, take the Negative of all terms, optionally using the identity
 | (12) |
 | (13) |
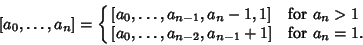 | (14) |
Consider the Convergents  of a simple continued fraction, and define of a simple continued fraction, and define
 | (15) |
 | (16) |
 | (17) |
 | (18) |
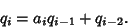 | (19) |
 | (20) |
 , ,
Furthermore,
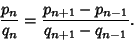 | (23) |
 , then , then
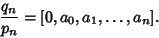 | (24) |
 , then , then
 | (25) |
 also satisfy also satisfy
The Even convergents 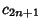 of an infinite simple continued fraction form an Increasing Sequence, and theOdd convergents of an infinite simple continued fraction form an Increasing Sequence, and theOdd convergents 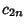 form a Decreasing Sequence (so any Even convergent is less than any Oddconvergent). Summarizing, form a Decreasing Sequence (so any Even convergent is less than any Oddconvergent). Summarizing,
 | (28) |
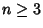 lies between the two preceding ones. Each convergent is nearer to the value ofthe infinite continued fraction than the previous one. In addition, for a number lies between the two preceding ones. Each convergent is nearer to the value ofthe infinite continued fraction than the previous one. In addition, for a number  , ,
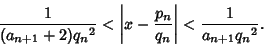 | (29) |
The Square Root of a Squarefree Integer has a periodic continued fraction of the form
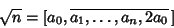 | (30) |
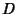 is not a Square Number, then the terms of the continued fraction of is not a Square Number, then the terms of the continued fraction of satisfy satisfy
 | (31) |
 | (36) |
The first follows from
Therefore,
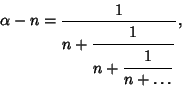 | (40) |
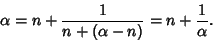 | (41) |
 | (42) |
 | (43) |
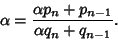 | (44) |
 |  |  |  | | 2 |  | 22 |  | | 3 | 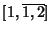 | 23 | 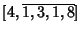 | | 5 | 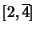 | 24 | 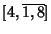 | | 6 | 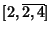 | 26 | 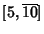 | | 7 | 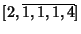 | 27 | 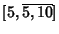 | | 8 |  | 28 | 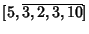 | | 10 | 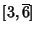 | 29 | 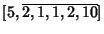 | | 11 | 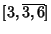 | 30 |  | | 12 |  | 31 | 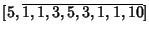 | | 13 | 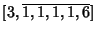 | 32 |  | | 14 |  | 33 |  | | 15 |  | 34 | 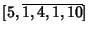 | | 17 | 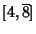 | 35 | 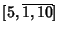 | | 18 |  | 37 | 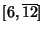 | | 19 |  | 38 | 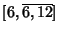 | | 20 |  | 39 |  | | 21 | 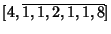 | 40 |  |
The periods of the continued fractions of the square roots of the first few nonsquare integers 2, 3, 5, 6, 7, 8, 10,11, 12, 13, ... (Sloane's A000037) are 1, 2, 1, 2, 4, 2, 1, 2, 2, 5, ... (Sloane's A013943; Williams 1981, Jacobson et al. 1995).An upper bound for the length is roughly  . .
An even stronger result is that a continued fraction is periodic Iff it is a Root of a quadraticPolynomial. Calling the portion of a number  remaining after a given convergent the ``tail,'' it must be true thatthe relationship between the number remaining after a given convergent the ``tail,'' it must be true thatthe relationship between the number  and terms in its tail is of the form and terms in its tail is of the form
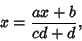 | (45) |
Logarithms 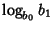 can be computed by defining can be computed by defining  , ...and the PositiveInteger , ...and the PositiveInteger 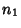 , ...such that , ...such that
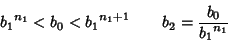 | (46) |
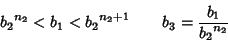 | (47) |
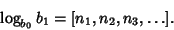 | (48) |
A geometric interpretation for a reduced Fraction  consists of a string through a Lattice of points withends at consists of a string through a Lattice of points withends at 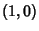 and and  (Klein 1907, 1932; Steinhaus 1983; Ball and Coxeter 1987, pp. 86-87; Davenport 1992). Thisinterpretation is closely related to a similar one for the Greatest Common Divisor. The pegs it presses against (Klein 1907, 1932; Steinhaus 1983; Ball and Coxeter 1987, pp. 86-87; Davenport 1992). Thisinterpretation is closely related to a similar one for the Greatest Common Divisor. The pegs it presses against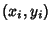 give alternate Convergents give alternate Convergents 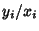 , while the other Convergentsare obtained from the pegs it presses against with the initial end at , while the other Convergentsare obtained from the pegs it presses against with the initial end at 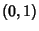 . The above plot is for . The above plot is for 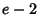 , which hasconvergents 0, 1, 2/3, 3/4, 5/7, .... , which hasconvergents 0, 1, 2/3, 3/4, 5/7, ....
Let the continued fraction for  be written be written 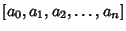 . Then the limiting value is almost alwaysKhintchine's Constant . Then the limiting value is almost alwaysKhintchine's Constant
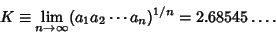 | (49) |
Continued fractions can be used to express the Positive Roots of any Polynomial equation. Continued fractionscan also be used to solve linear Diophantine Equations and the Pell Equation. Euler  showed that if a Convergent Series can be written in the form showed that if a Convergent Series can be written in the form
 | (50) |
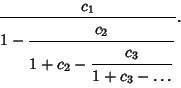 | (51) |
Gosper has invented an Algorithm for performing analytic Addition, Subtraction, Multiplication,and Division using continued fractions. It requires keeping track of eight Integers which areconceptually arranged at the Vertices of a Cube. The Algorithm has not,however, appeared in print (Gosper 1996).
An algorithm for computing the continued fraction for  from the continued fraction for from the continued fraction for  is given byBeeler et al. (1972, Item 101), Knuth (1981, Exercise 4.5.3.15, pp. 360 and 601), and Fowler (1991). (In line 9 of Knuth's solution, is given byBeeler et al. (1972, Item 101), Knuth (1981, Exercise 4.5.3.15, pp. 360 and 601), and Fowler (1991). (In line 9 of Knuth's solution, should be replaced by should be replaced by  .)Beeler et al. (1972) andKnuth (1981) also mention the bivariate case .)Beeler et al. (1972) andKnuth (1981) also mention the bivariate case  . . See also Gaussian Brackets, Hurwitz's Irrational Number Theorem, Khintchine's Constant, Lagrange'sContinued Fraction Theorem, Lamé's Theorem, Lévy Constant,Padé Approximant, Partial Quotient, Pi, Quadratic Irrational Number,Quotient-Difference Algorithm, Segre's Theorem
References
 Continued Fractions Continued FractionsAbramowitz, M. and Stegun, C. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 19, 1972. Acton, F. S. ``Power Series, Continued Fractions, and Rational Approximations.'' Ch. 11 in Numerical Methods That Work, 2nd printing. Washington, DC: Math. Assoc. Amer., 1990. Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, pp. 54-57 and 86-87, 1987. Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, pp. 36-44, Feb. 1972. Beskin, N. M. Fascinating Fractions. Moscow: Mir Publishers, 1980. Brezinski, C. History of Continued Fractions and Padé Approximants. New York: Springer-Verlag, 1980. Conway, J. H. and Guy, R. K. ``Continued Fractions.'' In The Book of Numbers. New York: Springer-Verlag, pp. 176-179, 1996. Courant, R. and Robbins, H. ``Continued Fractions. Diophantine Equations.'' §2.4 in Supplement to Ch. 1 in What is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 49-51, 1996. Davenport, H. §IV.12 in The Higher Arithmetic: An Introduction to the Theory of Numbers, 6th ed. New York: Cambridge University Press, 1992. Euler, L. Introduction to Analysis of the Infinite, Book I. New York: Springer-Verlag, 1980. Fowler, D. H. The Mathematics of Plato's Academy. Oxford, England: Oxford University Press, 1991. Guy, R. K. ``Continued Fractions'' §F20 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, p. 259, 1994. Jacobson, M. J. Jr.; Lukes, R. F.; and Williams, H. C. ``An Investigation of Bounds for the Regulator of Quadratic Fields.'' Experiment. Math. 4, 211-225, 1995. Khinchin, A. Ya. Continued Fractions. New York: Dover, 1997. Kimberling, C. ``Continued Fractions.'' http://cedar.evansville.edu/~ck6/integer/contfr.html. Klein, F. Ausgewählte Kapitel der Zahlentheorie. Germany: Teubner, 1907. Klein, F. Elementary Number Theory. New York, p. 44, 1932. Kline, M. Mathematical Thought from Ancient to Modern Times. New York: Oxford University Press, 1972. Knuth, D. E. The Art of Computer Programming, Vol. 2: Seminumerical Algorithms, 2nd ed. Reading, MA: Addison-Wesley, p. 316, 1981. Moore, C. D. An Introduction to Continued Fractions. Washington, DC: National Council of Teachers of Mathematics, 1964. Olds, C. D. Continued Fractions. New York: Random House, 1963. Pettofrezzo, A. J. and Bykrit, D. R. Elements of Number Theory. Englewood Cliffs, NJ: Prentice-Hall, 1970. Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. ``Evaluation of Continued Fractions.'' §5.2 in Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 163-167, 1992. Rose, H. E. A Course in Number Theory, 2nd ed. Oxford, England: Oxford University Press, 1994. Rosen, K. H. Elementary Number Theory and Its Applications. New York: Addison-Wesley, 1980. Sloane, N. J. A. SequencesA013943 andA000037/M0613in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995. Steinhaus, H. Mathematical Snapshots, 3rd American ed. New York: Oxford University Press, pp. 39-42, 1983. Van Tuyl, A. L. ``Continued Fractions.'' http://www.calvin.edu/academic/math/confrac/. Wagon, S. ``Continued Fractions.'' §8.5 in Mathematica in Action. New York: W. H. Freeman, pp. 263-271, 1991. Wall, H. S. Analytic Theory of Continued Fractions. New York: Chelsea, 1948. Williams, H. C. ``A Numerical Investigation into the Length of the Period of the Continued Fraction Expansion of  .'' Math. Comp. 36, 593-601, 1981. .'' Math. Comp. 36, 593-601, 1981.
|
![]() is of the form
is of the form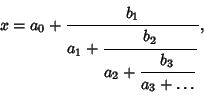

![]() (Pi). Becauseirrationals which are square roots of Rational Numbers have periodic continued fractions, an exactrepresentation for a tabulated numerical value (i.e., 1.414... for Pythagoras's Constant,
(Pi). Becauseirrationals which are square roots of Rational Numbers have periodic continued fractions, an exactrepresentation for a tabulated numerical value (i.e., 1.414... for Pythagoras's Constant, ![]() ) cansometimes be found.
) cansometimes be found.![]() used for calendrical purposes by the Greeks consists of 235 lunar monthswhich very nearly equal 19 solar years, and 235/19 is the sixth Convergent of the ratio of the lunar phase (synodic)period and solar period (365.2425/29.53059). Continued fractions can also be used to calculate gear ratios, and were usedfor this purpose by the ancient Greeks (Guy 1990).
used for calendrical purposes by the Greeks consists of 235 lunar monthswhich very nearly equal 19 solar years, and 235/19 is the sixth Convergent of the ratio of the lunar phase (synodic)period and solar period (365.2425/29.53059). Continued fractions can also be used to calculate gear ratios, and were usedfor this purpose by the ancient Greeks (Guy 1990).![]() beconvergents of a nonsimple continued fraction. Then
beconvergents of a nonsimple continued fraction. Then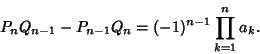
![]() , in which case it should be added to the previous term(Beeler et al. 1972, Item 101A). To take one over a continued fraction, add (or possibly delete) an initial 0 term. Tonegate, take the Negative of all terms, optionally using the identity
, in which case it should be added to the previous term(Beeler et al. 1972, Item 101A). To take one over a continued fraction, add (or possibly delete) an initial 0 term. Tonegate, take the Negative of all terms, optionally using the identity![]() of a simple continued fraction, and define
of a simple continued fraction, and define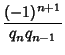
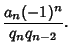
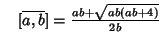
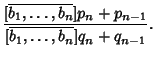


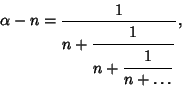
![]() .
.![]() remaining after a given convergent the ``tail,'' it must be true thatthe relationship between the number
remaining after a given convergent the ``tail,'' it must be true thatthe relationship between the number ![]() and terms in its tail is of the form
and terms in its tail is of the form![]() can be computed by defining
can be computed by defining ![]() , ...and the PositiveInteger
, ...and the PositiveInteger ![]() , ...such that
, ...such that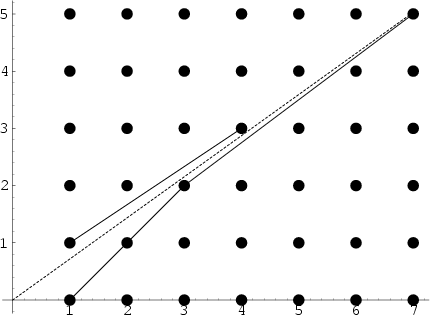
![]() consists of a string through a Lattice of points withends at
consists of a string through a Lattice of points withends at ![]() and
and ![]() (Klein 1907, 1932; Steinhaus 1983; Ball and Coxeter 1987, pp. 86-87; Davenport 1992). Thisinterpretation is closely related to a similar one for the Greatest Common Divisor. The pegs it presses against
(Klein 1907, 1932; Steinhaus 1983; Ball and Coxeter 1987, pp. 86-87; Davenport 1992). Thisinterpretation is closely related to a similar one for the Greatest Common Divisor. The pegs it presses against![]() give alternate Convergents
give alternate Convergents ![]() , while the other Convergentsare obtained from the pegs it presses against with the initial end at
, while the other Convergentsare obtained from the pegs it presses against with the initial end at ![]() . The above plot is for
. The above plot is for ![]() , which hasconvergents 0, 1, 2/3, 3/4, 5/7, ....
, which hasconvergents 0, 1, 2/3, 3/4, 5/7, ....![]() be written
be written ![]() . Then the limiting value is almost alwaysKhintchine's Constant
. Then the limiting value is almost alwaysKhintchine's Constant![]() showed that if a Convergent Series can be written in the form
showed that if a Convergent Series can be written in the form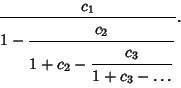
![]() from the continued fraction for
from the continued fraction for ![]() is given byBeeler et al. (1972, Item 101), Knuth (1981, Exercise 4.5.3.15, pp. 360 and 601), and Fowler (1991). (In line 9 of Knuth's solution,
is given byBeeler et al. (1972, Item 101), Knuth (1981, Exercise 4.5.3.15, pp. 360 and 601), and Fowler (1991). (In line 9 of Knuth's solution,![]() should be replaced by
should be replaced by ![]() .)Beeler et al. (1972) andKnuth (1981) also mention the bivariate case
.)Beeler et al. (1972) andKnuth (1981) also mention the bivariate case ![]() .
.![]() Continued Fractions
Continued Fractions![]() .'' Math. Comp. 36, 593-601, 1981.
.'' Math. Comp. 36, 593-601, 1981.