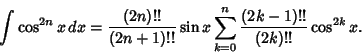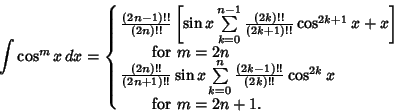| 释义 |
Cosine IntegralThere are (at least) three types of ``cosine integrals,'' denoted 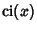 , , 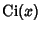 , and , and 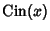 : :
Here, 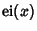 is the Exponential Integral, E is the Exponential Integral, E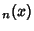 is the En-Function, and is the En-Function, and 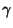 is the Euler-Mascheroni Constant. is the Euler-Mascheroni Constant. 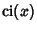 is the function returned by the Mathematica is the function returned by the Mathematica (WolframResearch, Champaign, IL) command CosIntegral[x] and displayed above. (WolframResearch, Champaign, IL) command CosIntegral[x] and displayed above.
To compute the integral of an Even power times a cosine,
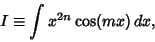 | (7) |
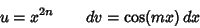 | (8) |
 | (9) |
 | (10) |
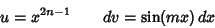 | (11) |
 | (12) |
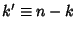 , ,
To find a closed form for an integral power of a cosine function,
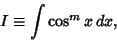 | (15) |
 | (16) |
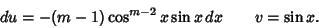 | (17) |
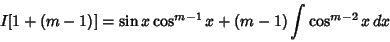 | (19) |
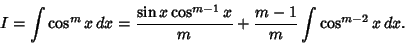 | (20) |
Now, if  is Even so is Even so  , then , then Now let 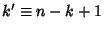 , so , so 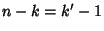 , , | | 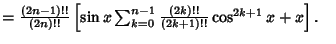 | (22) |
Now if  is Odd so is Odd so 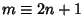 , then , then
Now let 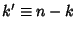 , ,
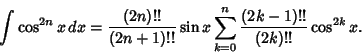 | (24) |
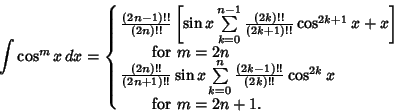 | (25) |
The infinite integral of a cosine times a Gaussian can also be done in closed form,
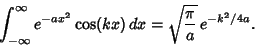 | (26) |
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Sine and Cosine Integrals.'' §5.2 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 231-233, 1972.Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 342-343, 1985. Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. ``Fresnel Integrals, Cosine and Sine Integrals.'' §6.79 in Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 248-252, 1992. Spanier, J. and Oldham, K. B. ``The Cosine and Sine Integrals.'' Ch. 38 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 361-372, 1987. |


![]() ,
, ![]() , and
, and ![]() :
: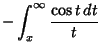
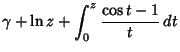

![]() is Even so
is Even so ![]() , then
, then![]() is Odd so
is Odd so ![]() , then
, then![]() ,
,