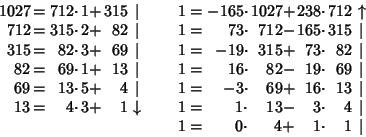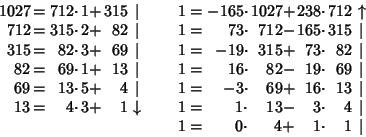| 释义 |
Diophantine Equation--LinearA linear Diophantine equation (in two variables) is an equation of the general form
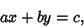 | (1) |
 , , 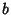 , and , and  Integers. Such equations can be solved completely, andthe first known solution was constructed by Brahmagupta. Consider the equation Integers. Such equations can be solved completely, andthe first known solution was constructed by Brahmagupta. Consider the equation
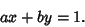 | (2) |
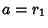 and and 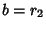
Starting from the bottom gives
so
Continue this procedure all the way back to the top.
Take as an example the equation
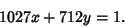 | (10) |
The solution is therefore 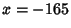 , , 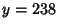 . The above procedure can be simplified by noting that the two left-mostcolumns are offset by one entry and alternate signs, as they must since . The above procedure can be simplified by noting that the two left-mostcolumns are offset by one entry and alternate signs, as they must since
so the Coefficients of 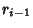 and and 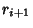 are the same and are the same and
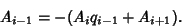 | (14) |
and we recover the above solution.
Call the solutions to
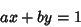 | (15) |
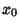 and and 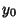 . If the signs in front of . If the signs in front of 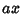 or or 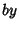 are Negative, then solve the above equation and take the signs ofthe solutions from the following table: are Negative, then solve the above equation and take the signs ofthe solutions from the following table:
In fact, the solution to the equation
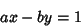 | (16) |
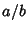 , with , with  and and 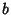 Relatively Prime (Olds 1963).If there are Relatively Prime (Olds 1963).If there are  terms in the fraction, take the terms in the fraction, take the 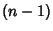 th convergent th convergent  . But . But
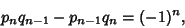 | (17) |
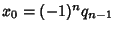 , , 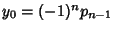 , with a general solution , with a general solution
with  an arbitrary Integer. The solution in terms of smallest Positive Integers is givenby choosing an appropriate an arbitrary Integer. The solution in terms of smallest Positive Integers is givenby choosing an appropriate  . .
Now consider the general first-order equation of the form
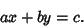 | (20) |
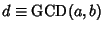 can be divided through yielding can be divided through yielding
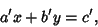 | (21) |
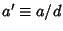 , , 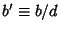 , and , and 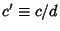 . If . If 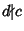 , then , then 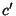 is not an Integer and theequation cannot have a solution in Integers. A necessary and sufficient condition for the generalfirst-order equation to have solutions in Integers is therefore that is not an Integer and theequation cannot have a solution in Integers. A necessary and sufficient condition for the generalfirst-order equation to have solutions in Integers is therefore that 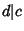 . If this is the case, thensolve . If this is the case, thensolve
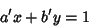 | (22) |
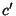 , since , since
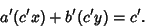 | (23) |
References
Courant, R. and Robbins, H. ``Continued Fractions. Diophantine Equations.'' §2.4 in Supplement to Ch. 1 in What is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 49-51, 1996.Dickson, L. E. ``Linear Diophantine Equations and Congruences.'' Ch. 2 in History of the Theory of Numbers, Vol. 2: Diophantine Analysis. New York: Chelsea, pp. 41-99, 1952. Olds, C. D. Ch. 2 in Continued Fractions. New York: Random House, 1963.
|