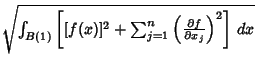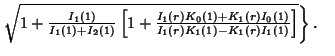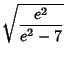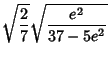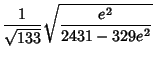| 释义 |
Whitney-Mikhlin Extension ConstantsN.B. A detailed on-line essay by S. Finchwas the starting point for this entry.
Let  be the be the 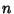 -D closed Ball of Radius -D closed Ball of Radius 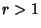 centered at the Origin. A function which isdefined on centered at the Origin. A function which isdefined on  is called an extension to is called an extension to  of a function of a function 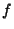 defined on defined on 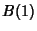 if if
 | (1) |
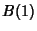 and and  , find the extension operator fromone to the other of minimal norm. Mikhlin (1986) found the best constants , find the extension operator fromone to the other of minimal norm. Mikhlin (1986) found the best constants  such that this condition, correspondingto the Sobolev such that this condition, correspondingto the Sobolev  integral norm, is satisfied, integral norm, is satisfied,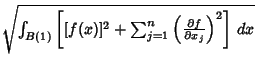 | |  | (2) |
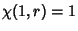 . Let . Let
 | (3) |
 , ,
 | (4) |
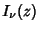 is a Modified Bessel Function of the First Kind and is a Modified Bessel Function of the First Kind and  is a Modified Bessel Functionof the Second Kind. For is a Modified Bessel Functionof the Second Kind. For  , , | | 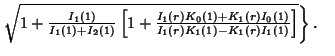 | (5) |
For  , ,
 | (6) |
 | (7) |
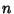 , the Recurrence Relations , the Recurrence Relations
with
where e is the constant 2.71828..., give
 | (14) |
Similar formulas can be given for even 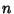 in terms of in terms of  , , 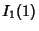 , ,  , , 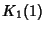 . .
References
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/mkhln/mkhln.htmlMikhlin, S. G. Constants in Some Inequalities of Analysis. New York: Wiley, 1986.
|
![]() be the
be the ![]() -D closed Ball of Radius
-D closed Ball of Radius ![]() centered at the Origin. A function which isdefined on
centered at the Origin. A function which isdefined on ![]() is called an extension to
is called an extension to ![]() of a function
of a function ![]() defined on
defined on ![]() if
if