“Euclid's Theorems”的概念、定义、习题解题方法及翻译-数学辞典

单词

Euclid's Theorems

释义

Euclid's Theorems

A theorem sometimes called ``Euclid's First Theorem'' or Euclid's Principle states that if is a Primeand , then or (where means Divides). A Corollary is that (Conway and Guy 1996). The Fundamental Theorem of Arithmetic is another Corollary (Hardyand Wright 1979).

Euclid's Second Theorem states that the number of Primes is Infinite. This theorem, also called theEuclid in Proposition IX.20 of the Elements. Ribenboim (1989) gives nine (and a half) proofs of this theorem. Euclid's elegant proof proceeds as follows. Given afinite sequence of consecutive Primes 2, 3, 5, ..., , the number

(1)

known as the

th Euclid Number when

is the

th Prime, is either a new Prime or the productof Primes. If

is a Prime, then it must be greater than the previous Primes, since one plus the product ofPrimes must be greater than each Prime composing the product. Now, if

is a product of Primes, then atleast one of the Primes must be greater than

. This can be shown as follows. If

is Composite and has no factorgreater than

, then one of its factors (say

) must be one of the Primes in the sequence, 2, 3, 5, ...,

. It therefore Divides the product

. However, since it is a factorof

, it also Divides

. But a number which Divides two numbers

and

also Divides their difference

, so

must also divide

(2)

However, in order to divide 1,

must be 1, which is contrary to the assumption that it is a Primein the sequence 2, 3, 5, .... It therefore follows that if

is composite, it has at least one factor greater than

. Since

is either a Prime greater than

or contains a prime factor greater than

, a Prime larger than thelargest in the finite sequence can always be found, so there are an infinite number of Hardy

(1967)remarks that this proof is ``as fresh and significant as when it was discovered'' so that ``two thousand years have notwritten a wrinkle'' on it.

A similar argument shows that and

(3)

must be either Prime or be divisible by a Prime

. Kummer

used a variation of this proof, which is also aproof by contradiction. It assumes that there exist only a finite number of Primes

, ...,

. Nowconsider

. It must be a product of Primes, so it has a Prime divisor

in common with

. Therefore,

which is nonsense, so we have proved the initial assumption is wrong by contradiction.

It is also true that there are runs of Composite Numbers which are arbitrarily long. This canbe seen by defining

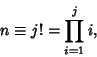(4)

where

is a Factorial. Then the

consecutive numbers

, ...,

are Composite, since

			(5)
			(6)
			(7)

Guy (1981, 1988) points out that while is not necessarily Prime, letting be the next Primeafter , the number is almost always a Prime, although it hasnot been proven that this must always be the case.

See also Divide, Euclid Number, Prime Number

References

Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, p. 60, 1987.

Conway, J. H. and Guy, R. K. ``There are Always New Primes!'' In The Book of Numbers. New York: Springer-Verlag, pp. 133-134, 1996.

Cosgrave, J. B. ``A Remark on Euclid's Proof of the Infinitude of Primes.'' Amer. Math. Monthly 96, 339-341, 1989.

Courant, R. and Robbins, H. What is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, p. 22, 1996.

Dunham, W. ``Great Theorem: The Infinitude of Primes.'' Journey Through Genius: The Great Theorems of Mathematics. New York: Wiley, pp. 73-75, 1990.

Guy, R. K. §A12 in Unsolved Problems in Number Theory. New York: Springer-Verlag, 1981.

Guy, R. K. ``The Strong Law of Small Numbers.'' Amer. Math. Monthly 95, 697-712, 1988.

Hardy, G. H. A Mathematician's Apology. Cambridge, England: Cambridge University Press, 1992.

Ribenboim, P. The Book of Prime Number Records, 2nd ed. New York: Springer-Verlag, pp. 3-12, 1989.

随便看

数学辞典收录了8975条数学词条，基本涵盖了常用数学知识及数学英语单词词组的翻译及用法，是数学学习的有利工具。