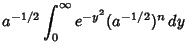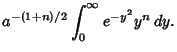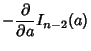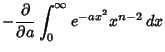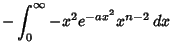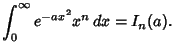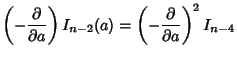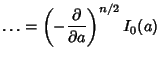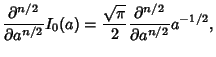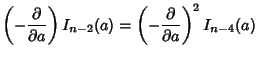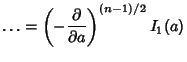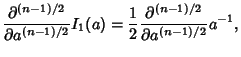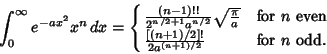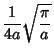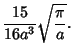| 释义 |
Gaussian IntegralThe Gaussian integral, also called the Probability Integral, is the integral of the 1-D Gaussian over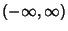 . It can be computed using the trick of combining two 1-D Gaussians . It can be computed using the trick of combining two 1-D Gaussians
and switching to Polar Coordinates,
However, a simple proof can also be given which does not require transformation to Polar Coordinates (Nicholas andYates 1950).
The integral from 0 to a finite upper limit  can be given by theContinued Fraction can be given by theContinued Fraction
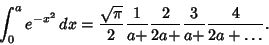 | (3) |
The general class of integrals of the form
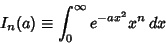 | (4) |
Then
For 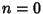 , this is just the usual Gaussian integral, so , this is just the usual Gaussian integral, so
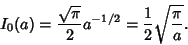 | (9) |
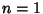 , the integrand is integrable by quadrature, , the integrand is integrable by quadrature,
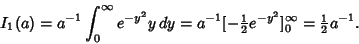 | (10) |
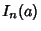 for for 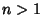 , use the identity , use the identity
For 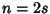 Even, Even,
so
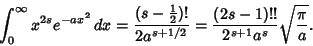 | (13) |
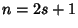 is Odd, then is Odd, then
so
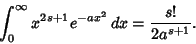 | (15) |
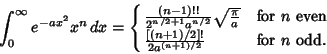 | (16) |
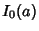 |  | 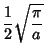 | (17) | 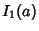 |  | 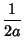 | (18) | 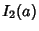 |  | 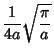 | (19) | 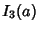 |  | 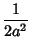 | (20) | 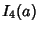 |  |  | (21) | 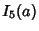 |  | 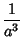 | (22) | 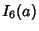 |  | 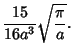 | (23) |
A related, often useful integral is
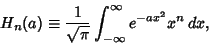 | (24) |
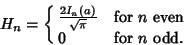 | (25) |
References
Nicholas, C. B. and Yates, R. C. ``The Probability Integral.'' Amer. Math. Monthly 57, 412-413, 1950. |
![]() . It can be computed using the trick of combining two 1-D Gaussians
. It can be computed using the trick of combining two 1-D Gaussians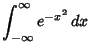
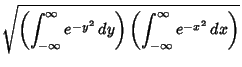
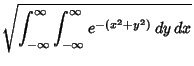
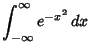
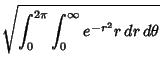
![]() can be given by theContinued Fraction
can be given by theContinued Fraction