Homoclinic Point
A point where a stable and an unstable separatrix (invariant manifold) from the same fixed point or same family intersect.Therefore, the limits
and
exist and are equal.
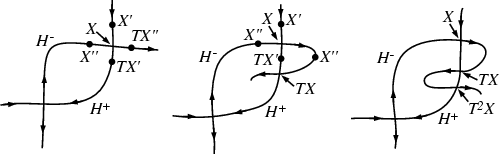
Refer to the above figure. Let 
be the point of intersection, with 
ahead of on one Manifold and 
ahead of of the other. The mapping of each of these points 
and 
must be ahead of the mapping of , 
.The only way this can happen is if the Manifold loops back and crosses itself at a new homoclinic point. Anotherloop must be formed, with 
another homoclinic point. Since is closer to the hyperbolic point than , thedistance between and is less than that between and . Area preservation requires the Area toremain the same, so each new curve (which is closer than the previous one) must extend further. In effect, the loopsbecome longer and thinner. The network of curves leading to a dense Area of homoclinic points is known as ahomoclinic tangle or tendril. Homoclinic points appear where Chaotic regions touch in a hyperbolicFixed Point.
A small Disk centered near a homoclinic point includes infinitely many periodic points of different periods.Poincaré 
showed that if there is a single homoclinic point, there are an infinite number. Morespecifically, there are infinitely many homoclinic points in each small disk (Nusse and Yorke 1996).
References
Nusse, H. E. and Yorke, J. A. ``Basins of Attraction.'' Science 271, 1376-1380, 1996. Tabor, M. Chaos and Integrability in Nonlinear Dynamics: An Introduction. New York: Wiley, p. 145, 1989.
- Pascal Distribution
- Pascal Line
- Pascal's Formula
- Pascal's Hexagrammum Mysticum
- Pascal's Limaçon
- Pascal's Rule
- Pascal's Theorem
- Pascal's Triangle
- Pascal's Wager
- Pasch's Axiom
- Pasch's Theorem
- Pass Equivalent
- Pass Move
- Patch
- Path
- Path Graph
- Path Integral
- Pathwise-Connected
- Patriarchal Cross
- Pauli Matrices
- Pauli Spin Matrices
- Payoff Matrix
- P-Circle
- Peacock's Tail
- Peano Arithmetic