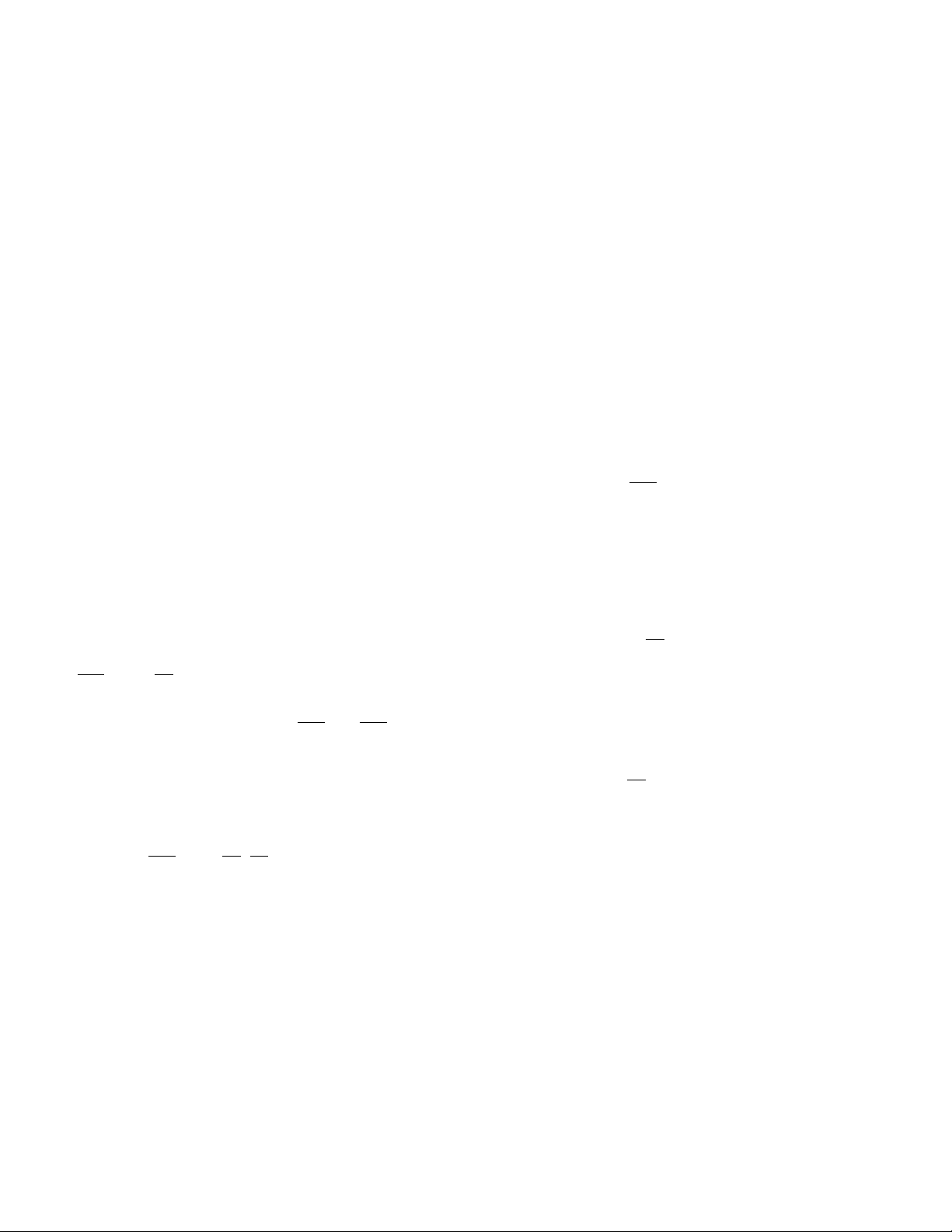
dy

–

–

dx

dy

–

–

dx

134 differential equation

dy

–

– = f(x)

dx

dP

–

– = kP

dt

DECREASING

;

MAXIMUM

/

MINIMUM

;

MEAN

-

VALUE THEO

-

REM

;

NUMERICAL DIFFERENTIATION

;

PARTIAL DERIVA

-

TIVE

; R

OLLE

’

S THEOREM

.

differential equation Any equation that contains

one or more derivatives is called a differential equation.

If such an equation involves just a single independent

variable xand a single dependent variable yand its

derivatives, then it is described as “ordinary.” For

example, the equation – 4x+ 3 = 0 is an ordinary

differential equation.

A solution of a differential equation is any func-

tion y = f(x) that satisfies the equation. For example,

y=2x2– 3xis a solution to the above equation. So too

is y=2x2– 3x+ 5. These solutions were found by

writing = 4x– 3 and integrating: y=∫4x– 3dx

=2x2– 3x+ C. If particular values of xand yare

known, say y= 0 when x= 1, then a value for Cis

determined, in this case C= 1, yielding the particular

solution: y=2x2– 3x+ 1.

The “order” of a differential equation is the order

of the highest derivative that appears in the equation,

and the “degree” of the equation is the power to which

the highest-order derivative is raised. Thus, for example,

is a third-order differential

equation of the first degree, and

is a third-order equation of degree 2.

An equation involving more than one independent

variable and

PARTIAL DERIVATIVE

s with respect to those

variables is called a partial-differential equation. For

example, is a second-order

partial differential equation of degree 3.

Differential equations arise in virtually every

branch of science, economics, and engineering. Any

theory that attempts to describe relationships between

the rates of change of continuously varying quantities

leads to a differential equation. For example, one

model of population growth describes the rate of

change of population size Pas proportional to the size

of the population. This leads to the equation ,

where kis some constant. (See

POPULATION MODELS

.)

Finding solutions to differential equations is an essen-

tial part of scientific investigation.

There are a number of standard techniques for

solving certain types of differential equations. All

involve rearranging terms or transforming the equation

into a form that can be readily integrated. We outline a

selection of some basic techniques.

Directly Integrable Equations: A first-order equation of

the form:

is directly integrable and has solution given by y=

∫f(x)dx. Similarly, a second-order equation of the form:

can be solved by integrating twice.

Separation of Variables: A first-order equation of the

form:

where fis a function of yonly and gis a function of x

only, can be solved by integrating both sides of the

equation with respect to x. This yields:

The method of

INTEGRATION BY SUBSTITUTION

shows

that the left integral can be interpreted simply as an

integral with respect to the variable y, and so we are

permitted to write:

∫f(y)dy = ∫g(x)dx

We can now evaluate these integrals and solve for y.

For example, to solve write, with an abuse

of notation, dy = dx Integration gives

∫

dy = ∫1dx,

yielding, , or .

1

y= ——

–

C– x

1

–

–

= x– C

y

1

–

y2

1

–

y2

dy

–

– = y2

dx