194 figurate numbers

Triangular and square numbers

Properties of figurate numbers

Canadian mathematician John Charles Fields

(1863–1932) later donated funds to support this idea,

and the awards were created and named in his honor. It

was agreed that two gold medals would be awarded

every four years—at each quadrennial meeting of the

International Congress of Mathematicians. The first

awards were given in 1936 and, following a wartime

hiatus, were resumed in 1950. In 1966, due to the sig-

nificant expansion of mathematical research, it was

agreed that up to four medals could be awarded at any

given meeting.

The award itself consists of a cash prize and a

medal made of gold. A picture of A

RCHIMEDES OF

S

YRACUSE

, along with the quotation, “Transire suum

pectus mundoque potiri” (Rise above oneself and take

hold of the world), appears on one side of the medal.

On the reverse side is the inscription, “Congregati ex

toto orbe mathematici ob scripta insignia tribuere” (the

mathematicians of the world assembled here pay trib-

ute for your outstanding work).

Following Fields’s wish, the awards are presented

in recognition of existing work completed by a mathe-

matician, as well as potential for future achievement.

For this reason, the awards are usually given to mathe-

maticians under the age of 40.

A board of trustees set up by the University of

Toronto administers the awards, and a committee of

mathematicians appointed by the International

Congress of Mathematicians presents the medals to

recipients.

Laurent Lafforgue of the Institut des Hautes

Études Scientifiques, Buressre-Yvette, France, and

Vladimir Voevodsky of the Institute of Advanced

Study, Princeton, New Jersey, were the 2002 recipients

of the award. Lafforgue made significant contributions

to the so-called Langlands program, a series of far-

reaching conjectures proposed by Robert Langlands in

1967 that, if true, would unite disparate branches of

mathematics. Voevodsky was awarded the prize for his

work in algebraic geometry, a field that unites number

theory and geometry.

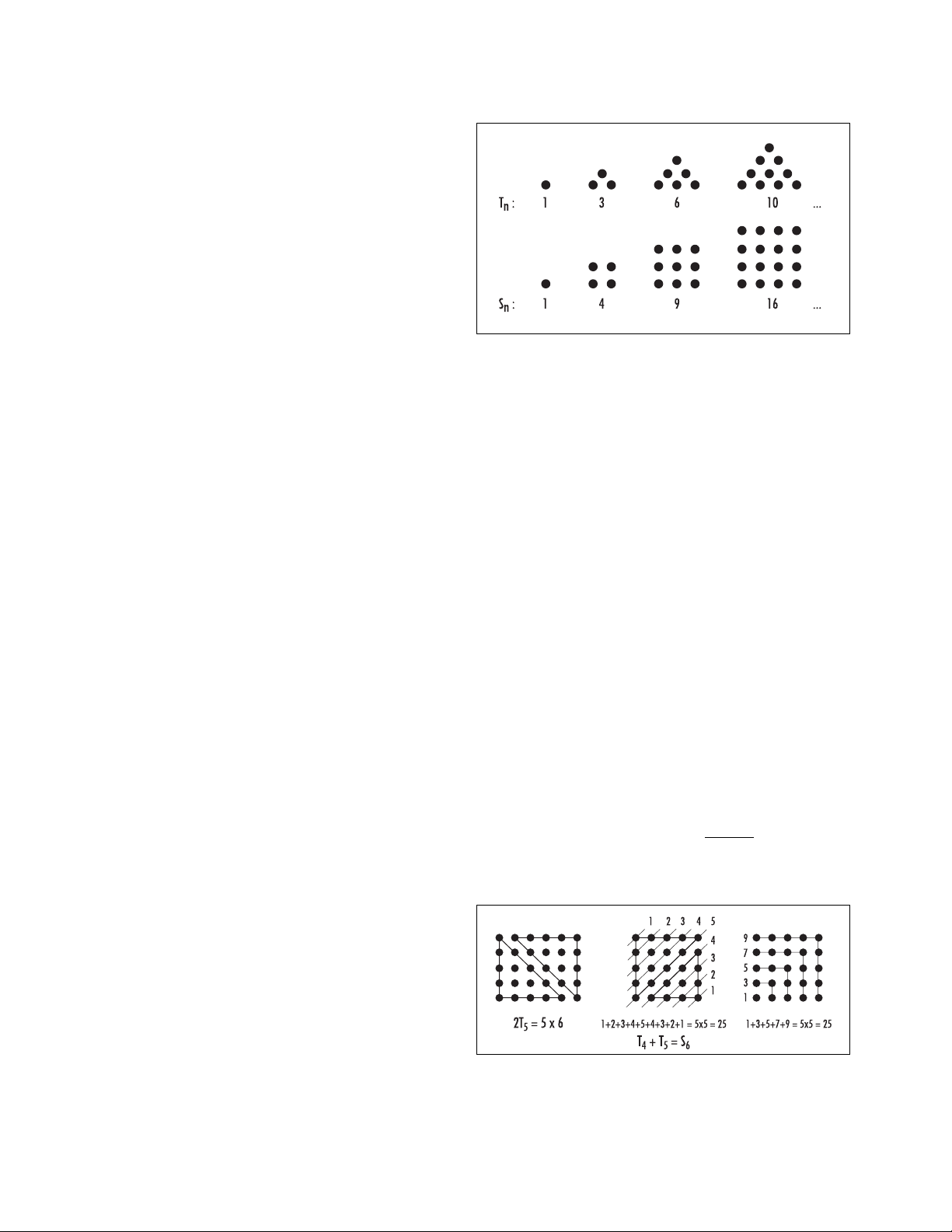
figurate numbers Arranging dots to create geomet-

ric figures leads to a class of numbers called figurate

numbers. For example, the triangular numbers are

those numbers arising from triangular arrangements of

dots, and the square numbers those from square arrays.

Other geometric shapes are possible, leading to other

sequences of figurate numbers.

Figurate numbers were of special importance to the

Pythagoreans of sixth century

B

.

C

.

E

. Believing that

everything in the universe could be explained by the

“harmony of number,” they imparted special impor-

tance, even personality, to the figurate numbers. For

example, 10, being the sum of the first four counting

numbers 1 + 2 + 3 + 4, in their belief united the four

elements—earth, water, fire, and air—and so was to be

held in the greatest of reverence. (They named this

number tetraktys, “the holy four.”)

Many arithmetic properties of sums can be read-

ily explained by the figurate numbers. For example,

the nth triangular number, Tn, is given by the sum: 1

+ 2 + … + n. As two triangular configurations placed

together produce an n×(n+ 1) array of dots, 2Tn, =

n×(n+ 1), we have: