of all values zthat lead to a bounded sequence is
called the “Julia set” for f. It is a region in the com-
plex plane that is almost always a fractal. Even simple
functions such as f(z) = z2+ c, with different values of
the constant c, can yield surprisingly varied and beau-
tiful structures.
Polish-born French mathematician Benoit Mandel-
brot considered, alternatively, all the complex values c
for which the repeated application of the function
f(z)= z2+ c, beginning with the point z= 0, yields a
bounded sequence. Plotting all such points cdescribes a
subset of the complex plane today called the Mandel-
brot set. It has an extremely complicated structure. In
particular, its boundary is a fractal.
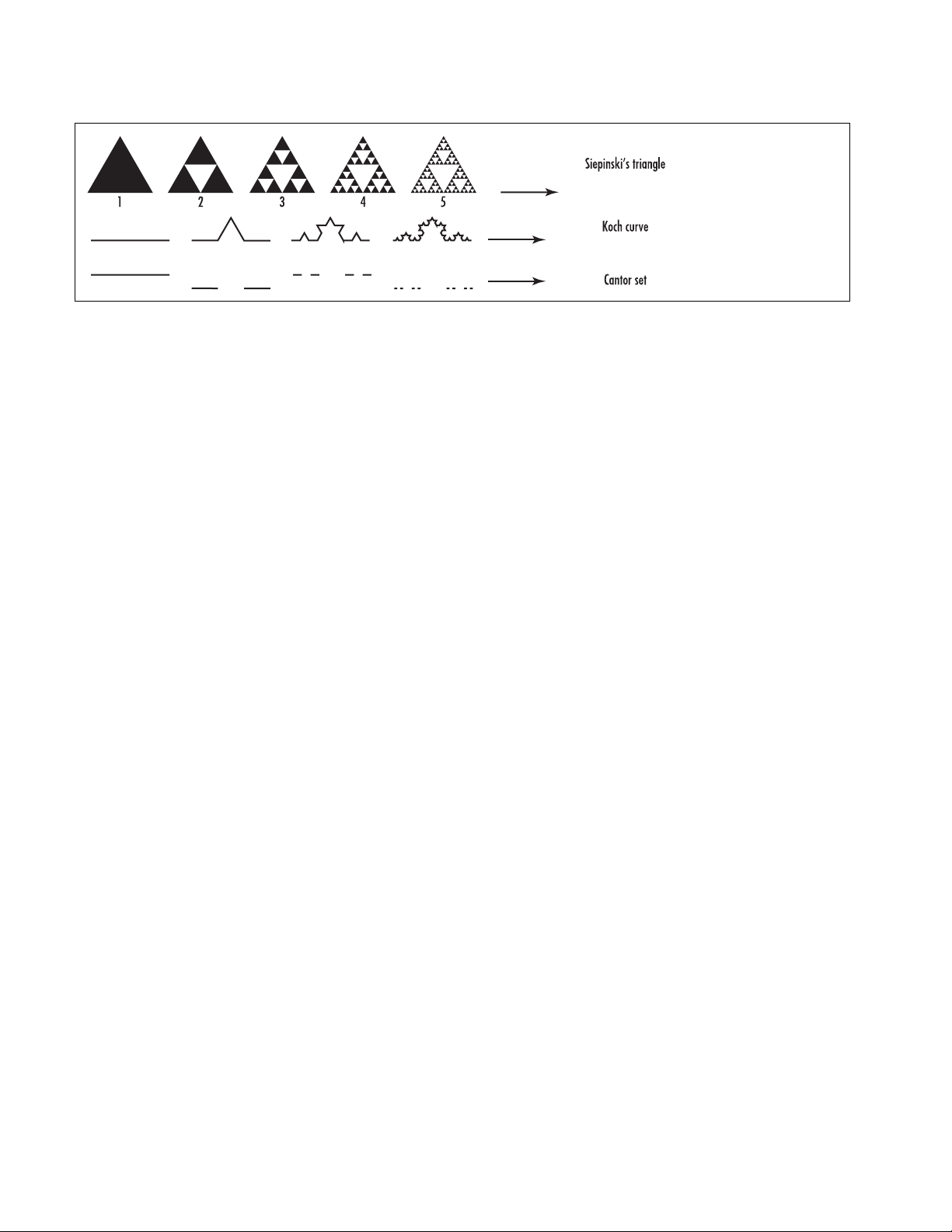
The Sierpinski triangle also arises from an iterative
procedure known as the “chaos game:”
Pick a point at random inside an equilateral tri-
angle (diagram (1) above) and then draw the
point halfway between it and one of the ver-
tices of the triangle picked at random. (This
point will lie somewhere in the shaded region
of diagram (2) above.) Now draw the point
halfway between this new point and another
vertex picked at random. (This point will lie
somewhere in the shaded region of diagram (3)
above.) Imagine we continue this process indef-
initely. Sierpinski’s triangle represents all possi-
ble “final” destinations of this point as this
game is played an infinite number of times.
Many objects in nature seem to possess the self-
similarity properties of fractals. For example, the
boundary shape of a cloud looks just as irregular under
magnification as it does when looked at directly. Scien-
tists have found it possible to assign fractal dimensions
to various objects in nature. The study of fractals has
since found applications to the study of crystal forma-
tion, fluid mechanics, urban growth, linguistics, eco-
nomics, and many other diverse areas.
Much of the work in fractal geometry was pio-
neered by Benoit Mandelbrot, who also coined the
term fractal.
fractal dimension See
FRACTAL
.
fraction Any number written as a
QUOTIENT
, that is,
as one number adivided by another b, is a fraction. We
write a/b and call the dividend athe numerator of the
fraction and the divisor bthe denominator of the frac-
tion. It is assumed that bis not zero.
It is appropriate to regard fractions simply as
answers to division problems. For example, if six pies
are shared equally among three boys, then each boy
receives two pies. We write: 6/3 = 2. Similarly, if one
pie is shared among two boys, then the amount of pie
each boy receives is written 1/2. We, appropriately, call
this quantity “half.” Clearly if apies are shared with
just one boy, then that boy receives all apies. This
yields the observation:
Unit Denominator Rule: = a
Every fractional property can be explained with this
simple pie-sharing model. For instance, we have:
Cancellation Law: For any nonzero value xand
fraction we have = .
For instance, if apies are shared among bboys, then
doubling the number of pies and doubling the number
a
–
b
xa
–
xb
a
–
b
a
–
1
204 fractal dimension
Fractals