nonzero-sum

GAME THEORY

. Nash’s 1949 doctoral dis-

sertation “Non-Cooperative Games” (only 45 pages

long) proved to have such a profound impact on the

development and study of economic theory that it sim-

ply redefined the nature of economic thinking during

the four decades that followed. For this, he was hon-

ored as a corecipient of the 1994 Nobel Prize in eco-

nomics. Nash also made extraordinary contributions to

the field of

TOPOLOGY

and solved a number of break-

through problems in the study of Riemannian geometry.

Nash entered the Carnegie Institute of Technology

(now Carnegie-Mellon University) in 1945 with the

intent to study chemical engineering, but soon discov-

ered a passion for mathematics. He graduated 3 years

later with both bachelor and master degrees in mathe-

matics and entered Princeton University in 1948 to

commence work on a doctorate. He graduated just 2

years later.

By 1954 Nash had published five extraordinary

papers on the topic of game theory, including “Equi-

librium Points in N-person Games” in 1950, “The

Bargaining Problem” in 1950, and “Non-Cooperative

Games” in 1951, and two truly significant works on

the topic of analytic geometry, “Real Algebraic Mani-

folds” in 1952, and “C1Isometric Imbeddings” in

1954. He had clearly established himself as a mathe-

matical genius and was recognized as such by the

mathematical community. He was a tenured professor

at the Massachusetts Institute of Technology at the

age of 29.

In the early 1960s, Nash began developing para-

noid schizophrenia and became virtually incapacitated

by the disease for the two decades that followed. He

lost his position at M.I.T, spent a number of years

roaming Europe and America, and returned to Prince-

ton, New Jersey, before receiving institutional help.

Nash, John 347

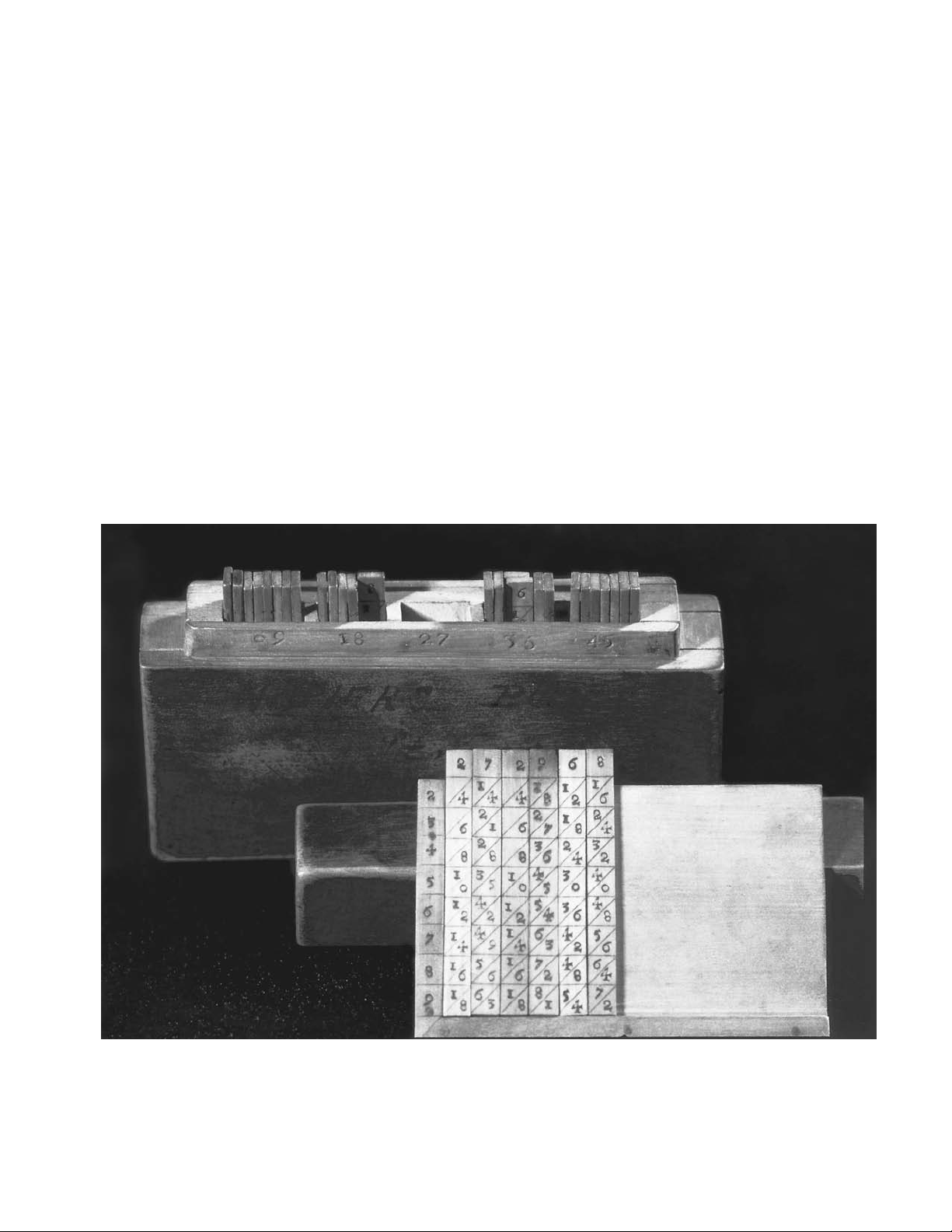
Napier’s bones, such as this set of rods dating from 1690, provided the means to simplify the process of long multiplication. (Photo

courtesy of the Science Museum, London/Topham-HIP/The Image Works)