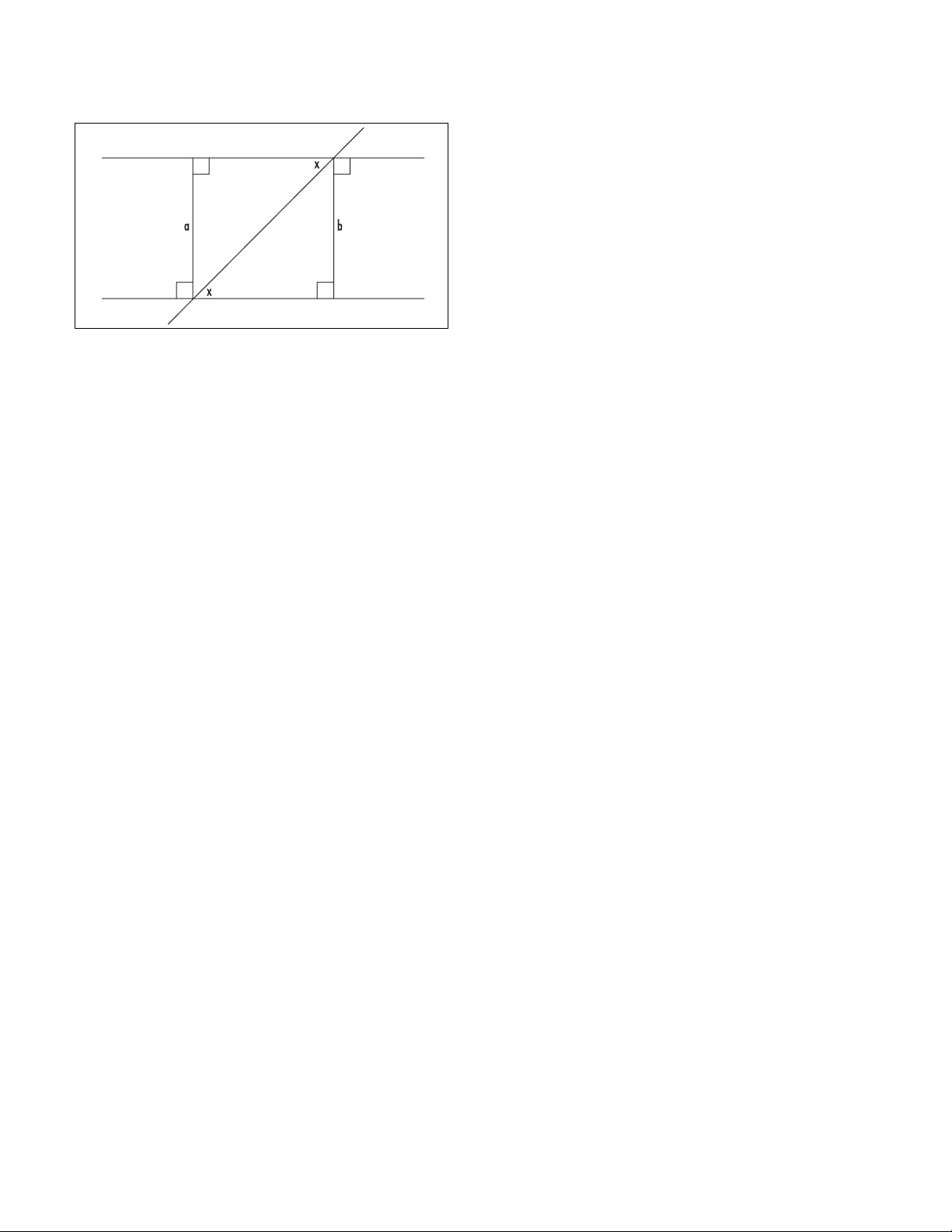
to a given line L, draw an arbitrary line L′through P
that intersects L. Measure the angle between Land L′,
and draw another line L′′ through P, making this angle
with L′so as to create a diagram with equal alternate
interior angles. By the result above, L′′ is a line through
Pparallel to L.
Scottish mathematician John Playfair (1748–1819)
proved that for any given point Pand line Lin the
plane, there is one, and only one, line through Pparal-
lel to L. This result is today known as P
LAYFAIR
’
S
AXIOM
. Playfair proved that one could take this axiom
as a starting point for geometry and establish the paral-
lel postulate from it.
See also
AAA
/
AAS
/
ASA
/
SAS
/
SSS
;
HISTORY OF GEOME
-
TRY
(essay);
PARALLELOGRAM
;
SIMILAR FIGURES
.
parallelepiped (parallelopiped) A solid figure with
six faces, each of which is a
PARALLELOGRAM
, is called
a parallelepiped. A cube with all six faces square is a
special example of a parallelepiped, as is a “rectangular
box” with six rectangular faces (also called a cuboid).
In these two examples, edges meet at the corners of the
solids at 90°angles, but this need not be the case for a
general parallelepiped.
The volume of a parallelepiped is the area of the
BASE
of the figure multiplied by the figure’s height. If
the three edges meeting at a corner are regarded as vec-
tors a, b, and c, then the volume of the parallelepiped is
also given by the absolute value of the
TRIPLE VECTOR
PRODUCT
a· b×c.
A parallelotope is a parallelepiped whose three
side-lengths are in the ratio 4:2:1.
See also
PRISM
.
parallelogram A
QUADRILATERAL
with opposite sides
parallel, and hence equal in length, is called a parallelo-
gram. The area of a parallelogram is “base times height.”
That is to say, if one pair of parallel sides, of length b, are
hunits apart, then the area of the figure is bh. (See
AREA
.)
An examination of alternate angles across parallel
lines quickly shows that opposite angles in a parallelo-
gram are equal and that adjacent angles are supplemen-
tary, that is, sum to 180°. The parallelogram is the only
quadrilateral with this property.
The
DIAGONAL
s of a parallelogram bisect one
another, and again the parallelogram is the only
quadrilateral that has this property. If the two different
side-lengths of a parallelogram are aand b, and the
length of its two diagonals are pand q, then
p2+ q2= 2a2+ 2b2
This follows from applying the
LAW OF COSINES
to the
four triangles defined by the diagonals (two triangles
on either side of each diagonal) and adding all four
equations. Interpreted as an equation of
VECTOR
lengths, this establishes the
PARALLELOGRAM LAW
in
vector-space theory.
A parallelogram with all four sides equal in length
is called a rhombus (or sometimes a rhomb, diamond,
or a lozenge). A rhomboid is a parallelogram with
adjacent sides unequal in length.
If all four angles of a parallelogram are equal, then
each angle equals 90°, and the figure is a
RECTANGLE
.
A parallelogram that is both equilateral and equiangu-
lar is a
SQUARE
.
See also
TRAPEZOID
/
TRAPEZIUM
.
parallelogram law (parallelogram rule) The fact
that
VECTOR
addition is commutative is sometimes
called the parallelogram law. This is appropriate, since
the sum of the two vectors aand bin the plane, a+ b,
is given as the
DIAGONAL
of the parallelogram defined
by the two vectors. Note that the second diagonal is
given by the vector difference a– b. The geometric
properties of a parallelogram establish that the lengths
of these vectors satisfy the relation:
a+ b
2+
a– b
2= 2
a
2+ 2
b
2
This equation is also called the parallelogram law.
See also
COMMUTATIVE PROPERTY
.
376 parallelepiped
Parallel lines