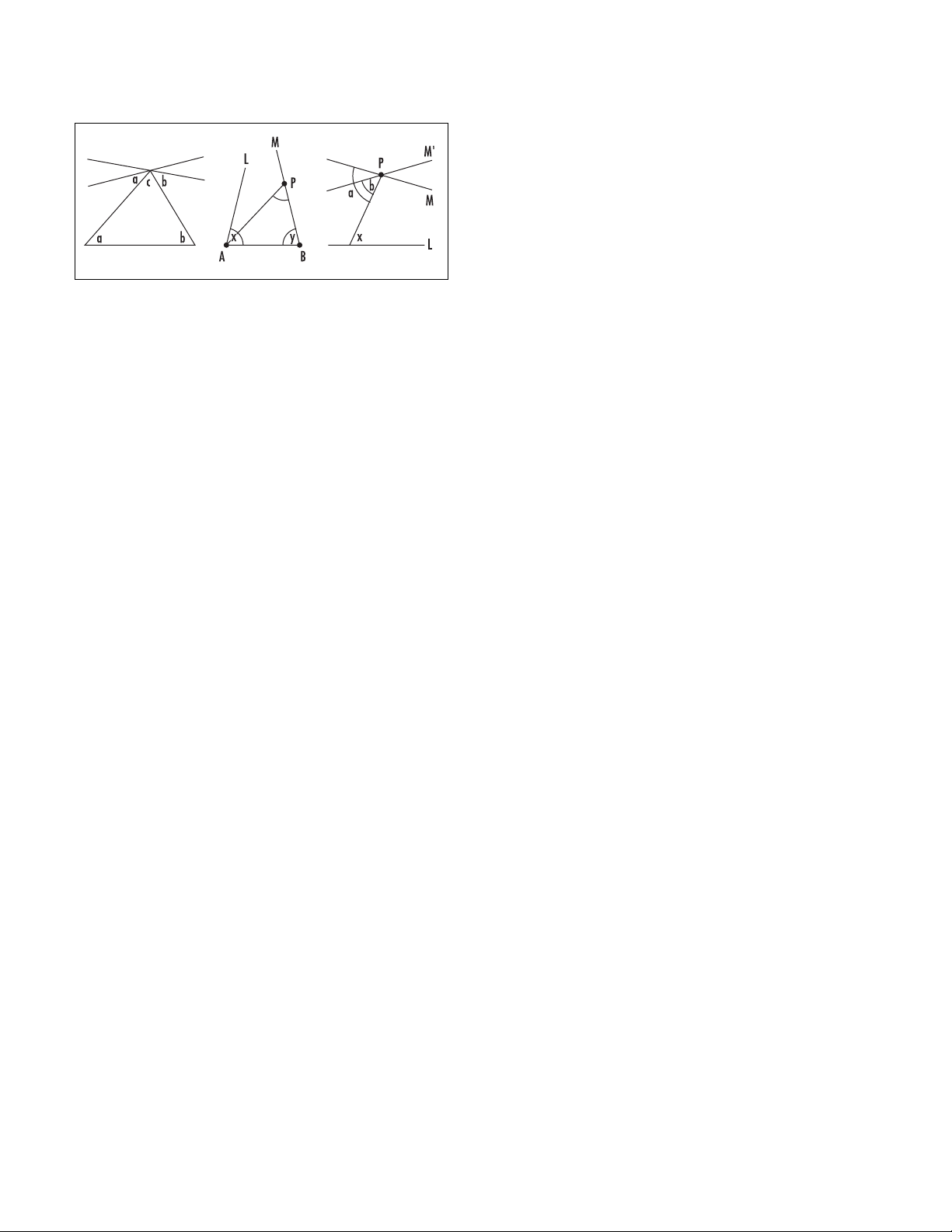
be that angles xand ysum to less that 180°? Draw a
point Pon line M, and slide it along Msufficiently far
so that the angle of the triangle APB at Pis less than
180 – x– y. Clearly the angle in this triangle at Ais less
than x. We now have a triangle with three angles that
sum to something less than x+ (180 – x– y) + y= 180°.
This is impossible by our assumption that (2) is true. It
cannot be the case that Land Mare parallel after all.
(1) implies (3):
Discussion on the exterior-angle theorem shows that
there certainly exists at least one line through a point P
parallel to a given line L. Suppose there are two such
lines. Call them Mand M′as shown in the diagram
above. Draw any line from Pto L. Since, by (1), we are
assuming that alternate interior angles are equal for par-
allel lines, both angles aand bshown are equal to x.
Consequently Mand M′must be the same line.
See also
HISTORY OF GEOMETRY
(essay).
parameter See
PARAMETRIC EQUATIONS
.
parametric equations (freedom equations) When
we think of a curve as the path traced by a moving
point, it is convenient to represent the curve as two
equations, one for each coordinate xand y, in terms of
a third variable usually denoted t: x = f(t) and y= g(t).
The variable tis called a parameter (from the Greek
para, meaning “together,” and meter, meaning “mea-
sure”). Equations with parameters are called paramet-
ric equations. In physical problems, tis usually thought
of as time.
Any curve y= f(x) can be expressed in terms of para-
metric equations: simply set x= tand consequently y= f(t).
Parametric representations, however, are not unique.
The curve y= 4x2, for example, can be represented as
x=t, y = 4t2and also x= , y= t6. Infinitely many other
representations are possible.
A
CIRCLE
of radius rand center (m,n) has a para-
metric representation: x= m+ rcos tand y= n+ rsin t.
(Notice that (x– m)2+ (y– n)2= (rcost)2+ (rsint)2=
r2(cos2t+ sin2t) = r2, the equation of a circle.) Similarly,
an
ELLIPSE
+ = 1 has parameterization: x= acos t
and y= bsin t.
There is no general method for transforming an
equation of the form F(x,y) = 0 into parametric equa-
tions. Each individual example needs to be examined
carefully, and much ingenuity is often required. (For
example, it is not immediately clear what the paramet-
ric equations for the curve given by x5+ xy + y5= 0
could be.) For the reverse direction, one can attempt to
convert a pair of parametric equations into a single
equation of the form F(x,y) = 0 by solving for t. To
illustrate, consider the equations: x= 3t+ 1 and y= t–
1. The first equation yields t= (x– 1)/3, and the sec-
ond, t= y+ 1. Consequently: y+ 1 = (x– 1)/3, or y=
x/3 – 4/3. This shows that the parametric equations
under study represent a straight-line path.
The
SLOPE
of a curve given via parametric equations
is = provided the
DERIVATIVE
x′(t) is not zero.
This follows from the
CHAIN RULE
for differentiation: if
yis a function of x, which itself is a function of t, then
= ·.
Parametric equations are also used to describe the
motion of particles in three-dimensional space. One is
given three equations in a parameter t, one for each of
the three coordinates x, y, and z.
See also
ARC LENGTH
;
CONSTANT WIDTH
.
parity Two integers that are either both even or both
odd are said to have the same parity. For instance, 17
and 53 have the same parity (both are odd), and 9 and
14 have opposite parity. The study of
EVEN AND ODD
NUMBERS
often makes use of parity to establish a num-
ber of sophisticated results.
Sometimes the term parity is used in a more general
setting as to mean “being in one of two possible states.”
For example, three hockey pucks labeled A, B, and C
lying on a playing field, not in a straight line, can be in
dy
––
dt
dy
––
dx
dy
––
dt
y′(t)
––
x′(t)
dy
––
dx
y2
–
b2
x2
–
a2
t3
–
2
378 parameter
Equivalent versions of the parallel postulate