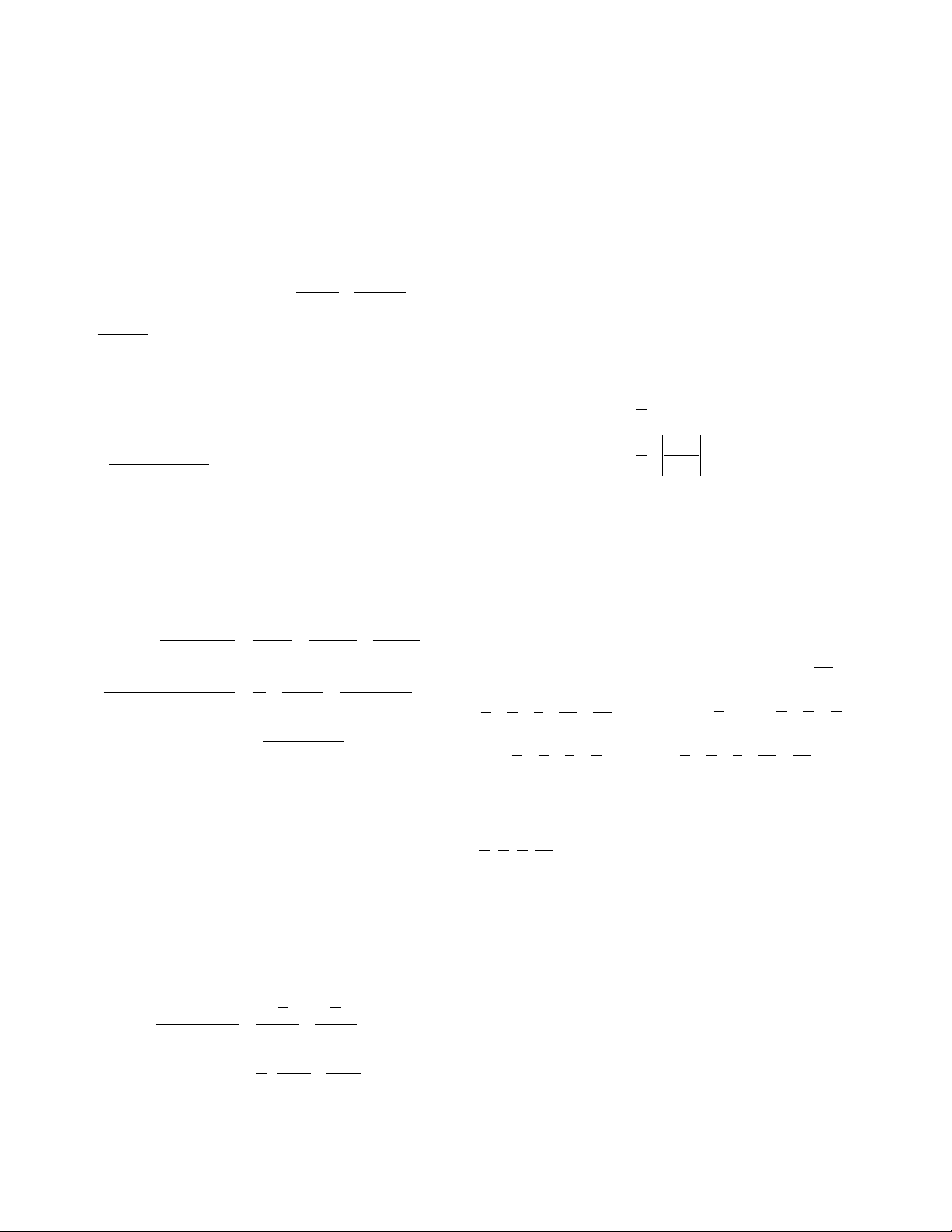
p(x)

––

q(x)

380 partial order

+

p(x)

––

q(x)

p(x) less than the degree of q(x),can be split into partial

fractions for easier handling. This first requires splitting

the denominator q(x) into linear and irreducible

quadratic factors, each to some index, and then writing

as a sum of terms of each of the following type:

1. Corresponding to each (repeated) linear term (x– k)n

in q(x),there is a sum of terms

.

2. Corresponding to each (repeated) irreducible

quadratic terms (ax2+ bx + c)min q(x),there is a

sum of terms

.

Here the numbers Ai, Bi, and Ciare constants. Some

examples illustrate the process:

The values of the constants A, B, C, … are found by

multiplying through by the denominator and then

EQUATING COEFFICIENTS

. Alternatively, one can substi-

tute appropriate values for the variable xto determine

the values of some of these unknowns more quickly.

For example, in the first example, after multiplying

through, we have: 4 = A(x– 3) + B(x+ 2). Setting x= 3

yields 4 = 0 + 5B, establishing that Bis 4/5, and setting

x= –2 gives A= –4/5. We thus have:

Mathematicians have proved that every rational

function , with the degree of p(x) less than the

degree of q(x),can indeed be written as a sum of partial

fractions, and that the constant terms appearing, Ai, Bi,

and Ci, are unique for that rational function. (That is,

no rational function can be expressed as a sum of par-

tial fractions in two different ways.) Partial fractions are

generally used for solving

INTEGRAL

s and in solving

DIFFERENTIAL EQUATION

s. As an example, we have:

partial order See

ORDERED SET

.

partial sum The nth partial sum Snof an infinite

series a1+ a2+ a3+… is the sum of just the first n

terms of the series: Sn= a1+ a2+…+ an. For example,

the first four partial sums of the series

are , ,

, and .

A series is said to converge to a value Lif the

partial sums Sntend to Lin the

LIMIT

as n→∞. In

the above example, the sequence of partial sums

approaches the value 1. Thus we

write: .

See also

CONVERGENT SERIES

.

partition In

NUMBER THEORY

a partition of a natural

number nis a representation of nas a sum of positive

integers. For example, 20 + 15 + 5 and 10 + 10 + 10 +

10 are partitions of the number 40, as is the representa-

tion 40 itself. A partition is considered ordered if the

order of the terms in the sum is considered important.