(431–404
B
.
C
.
E
.) between Athens and Sparta, and he
traveled through Egypt and Italy once democracy was
restored to Athens. In Egypt, Plato learned of the
water clock and later brought the idea back to Greece.
In Italy he encountered the followers of P
YTHAGORAS
and was greatly influenced by the mathematics they
studied. Although Plato made no direct contributions
to mathematics, his respect for the subject was pro-
found, and he wrote about the philosophy of the sub-
ject at great lengths in his works.
Around 387
B
.
C
.
E
. Plato returned to Athens to
found a school devoted to instruction and research in
philosophy and the sciences. He secured a piece of land
from the Greek hero Academos, and the school became
known as the Academy. Plato presided over the insti-
tute for the remainder of his life.
Plato wrote a total of 35 dialogues, fictitious con-
versations between characters, cleverly structured so as
to reveal a philosophical line of thought and investiga-
tion. In his dialogue Phaedo, Plato described mathe-
matical objects as perfect forms (a line, for instance,
really is an object possessing no breadth), and pro-
fessed that the objects we see and can construct in the
physical universe are imperfect imitations of these ideal
forms. He also discussed these ideas in his work the
Republic. It is clear from his dialogues that Plato
regarded mathematics as a source of universal truth
and that the study of mathematics offered the best tool
for fostering intellectual development.
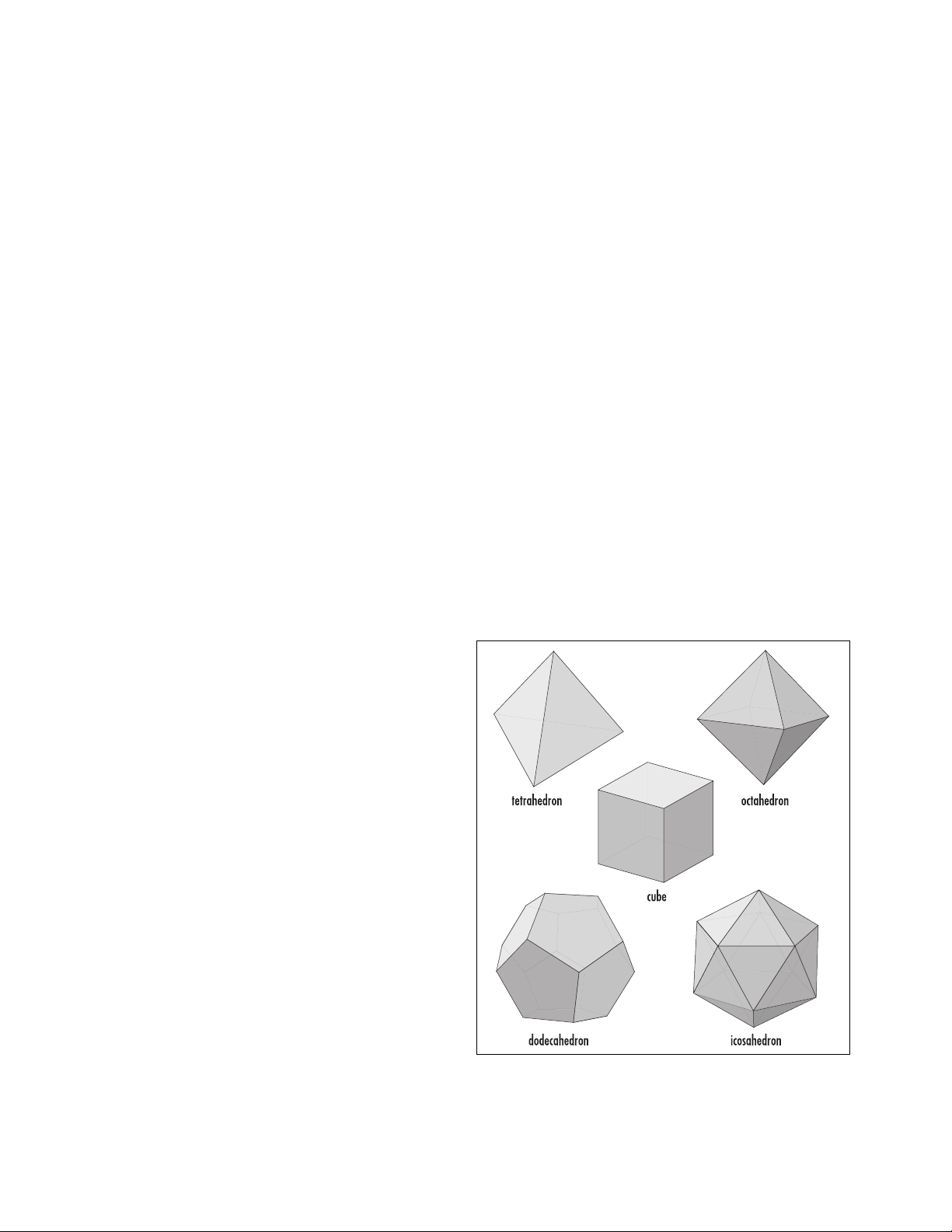
In his work Timaeus, Plato described the five Pla-
tonic solids as fundamental units of the universe. He
suggested, for instance, that each of the four “ele-
ments”—earth, fire, air, and water—is appropriately
modeled by the shape of a Platonic solid, namely, the
CUBE
, the
TETRAHEDRON
, the octahedron, and the
icosahedron, respectively. The fifth Platonic solid, the
dodecahedron, with its 12 faces matching the 12 signs
of the zodiac, represented the universe itself. German
astronomer J
OHANNES
K
EPLER
(1571–1630) was so
smitten with the proposed harmony of the Platonic
solids that he later developed a surprisingly accurate
model of the solar system based upon them.
Plato’s attitude toward, and regard for, the very
nature of mathematical thinking had a lasting influence
on the entire course of scientific progress in the West.
Apart from promoting the value of pure mathematics,
Plato also emphasized the need for rigorous proof,
thereby laying down a philosophical foundation for oth-
ers, most notably E
UCLID
, on which to pursue research.
Plato is also credited for limiting the use of geometrical
tools to the compass and straightedge alone.
Platonic solid (regular polyhedron) Any convex
POLY
-
HEDRON
, all of whose faces are congruent regular poly-
gons, is called a Platonic solid. For example, a
CUBE
,
composed of six identical square faces, is a Platonic solid,
as is a
TETRAHEDRON
composed of four identical equilat-
eral triangular faces. Platonic solids have the property
that not only are all of its faces identical, but all of its
vertices are also alike in the sense that the same number
of edges meet at each vertex of the polyhedron.
Regular polygons and regular polyhedra were stud-
ied extensively by the classical Greek scholars. In two-
dimensional space, regular polygons with any desired
number of sides exist. In three-dimensional space, how-
ever, there are only a small number of regular polyhe-
dra. As his final proposition in his revered work T
HE
E
LEMENTS
, the geometer E
UCLID
(ca. 300–260
B
.
C
.
E
.)
showed that there are only five Platonic solids, namely:
396 Platonic solid
The Platonic solids