428

Q

QED The abbreviation QED is short for quod erat

demonstrandum, Latin for “which was to be demon-

strated.” E

UCLID

of the third century

B

.

C

.

E

. wrote the

Greek equivalent of this phrase at the end of each of his

proofs to indicate that its conclusion had been reached.

Many mathematicians still follow this tradition.

The initials QEF, for quod erat faciendum (which

was to be done), are sometimes added after the comple-

tion of a geometrical construction, and QEI, for quod

erat inveniendum (which was to be found), after the

completion of a calculation.

quadrant In a system of C

ARTESIAN COORDINATES

,

the two coordinate axes divide the plane into four

regions called quadrants. By convention, the quadrants

are numbered, with the first quadrant being the one

above the x-axis and to the right of the y-axis (this is

the region in which both xand yare positive), and the

second, third, and fourth quadrants arranged counter-

clockwise about the origin.

Many popular science fiction texts describe three-

dimensional space as being divided into four quad-

rants. This is incorrect usage of the term.

One-quarter of the circumference of a circle is some-

times called a quadrant, as is one quarter of the interior

of a circle (bounded by two perpendicular radii).

See also

OCTANT

.

quadratic (quadric) Any expression, function, or

equation containing variables raised to the second

power, but no higher power, is described as quadratic.

Thus a quadratic polynomial, for instance, is a

POLY

-

NOMIAL

of second degree, and a quadratic equation is

an equation formed by setting a quadratic polynomial

equal to zero. Such an equation has the form ax2+ bx

+ c= 0 with a, b, and ceither

REAL

or

COMPLEX NUM

-

BERS

and anonzero.

Quadratic equations often arise when computing

the dimensions of rectangles. (This explains the use of

the prefix “quad” in their name, derived from the Latin

name quattuor for “four.”) For instance, told that a

quadrangle of area 55 ft2has length 6 units longer than

its width leads to the equation (given in terms of the

unknown width x) x(x+ 6) = 55 which is the quadratic

equation x2+ 6x– 55 = 0. Ancient Egyptian and Baby-

lonian scholars of ca. 2000

B

.

C

.

E

. were the first to con-

tend with problems of this type.

The technique of

COMPLETING THE SQUARE

pro-

vides a simple method for solving all quadratic equa-

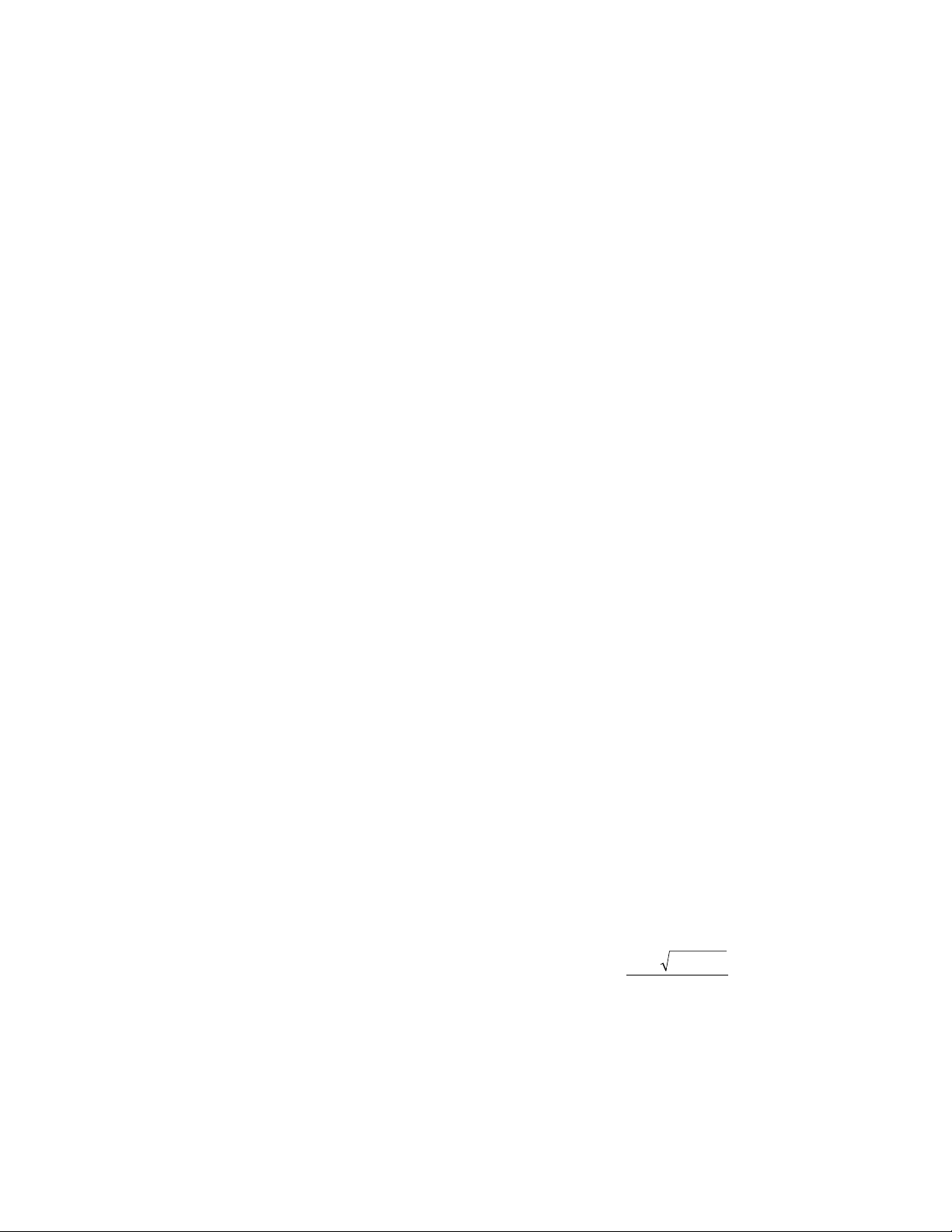
tions. It also leads to the famous quadratic formula:

A quadratic equation of the form ax2+ bx + c

= 0 with a≠0 has solutions given by:

For example, the quadratic formula shows that x2+ 6x

– 55 = 0 has solutions x= 5 and x= –11. (The positive

solution provides an acceptable answer to our quad-

rangle problem.)