circumvent the issues raised by the paradox and to find
a logical base for all of mathematics. He introduced a
notion of “type,” demanding that sets and sentences in
mathematics belong to a hierarchical structure. At the
lowest level there are statements about individual ele-
ments. At the next level there are sentences about sets
of individual elements, and next, sentences about sets
of sets of individual elements, and so forth. Moreover,
no sentence is permitted to be at the same level as its
subject. Hence any statement that refers to itself (such
as the definition of the set N) is simply not allowed in
this hierarchical structure. This approach certainly cir-
cumvents self-referential paradoxes, but many members
of the mathematics community felt, however, that this
construct was not particularly satisfying.
Observe that the barber’s paradox is easily
resolved by noting that the barber could be outside the
set of men, namely, the barber could be a woman. Rus-
sell’s theory of type attempted to mimic this solution
by bringing sets “outside of themselves” through the
notion of a hierarchy. Mathematicians today are
attempting instead to develop a mathematical theory
of “context” where the setting in which statements are
made is considered important.
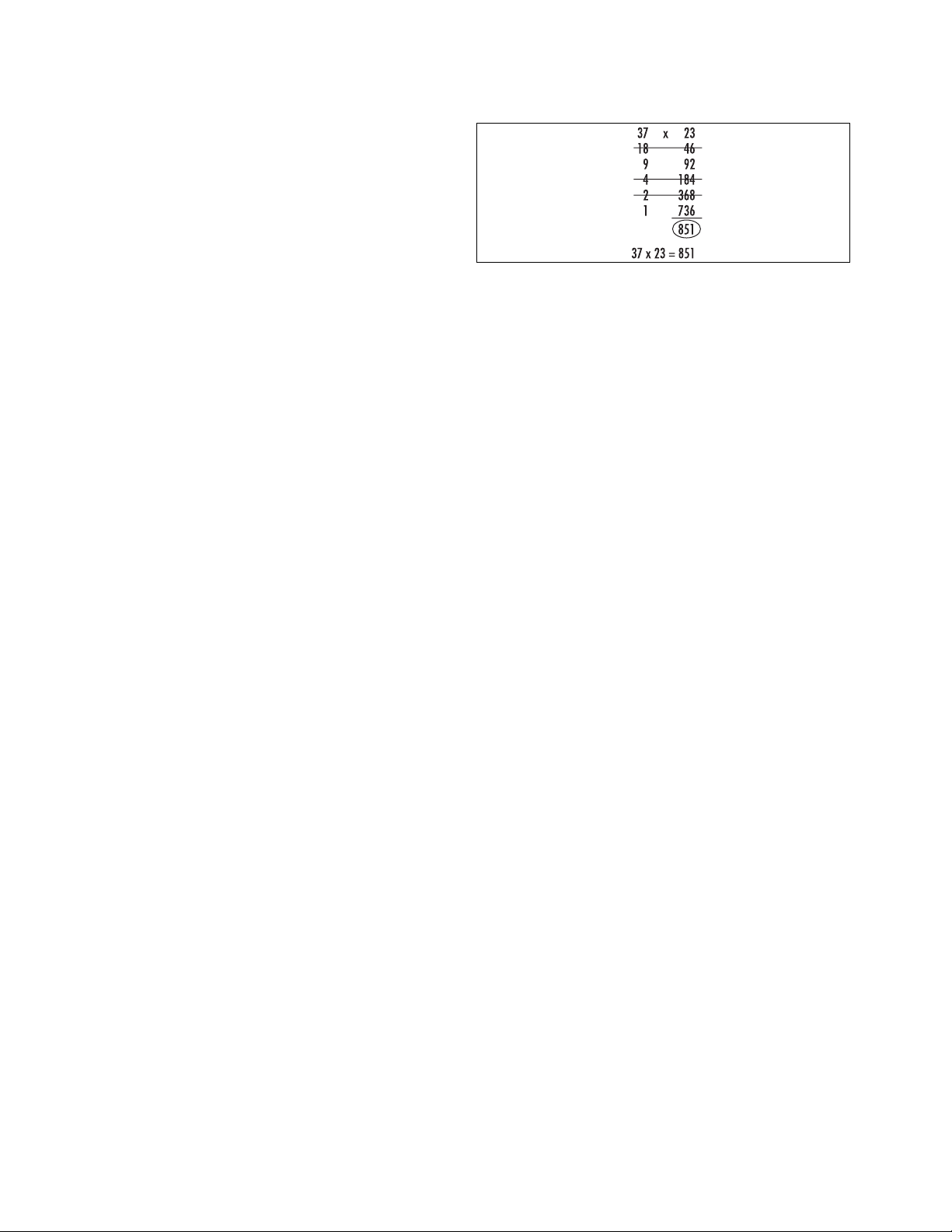
Russian multiplication Also known as peasant mul-
tiplication, the following multiplication method is
believed to have originated in Russia.
1. Head two columns with the numbers you wish to
multiply.
2. Progressively halve the numbers in the left column
(ignoring remainders) while doubling the figures in
the right column. Reduce the left column to one.
3. Delete all rows with an even number in the left
column.
4. Add all the surviving numbers in the right column.
This sum is the desired product.
The key to understanding why this method works
is to note that every number can be written as a sum of
the powers of 2: 1,2,4,8,16,32,… For example, 37 = 32
+ 4 + 1. That 37 is odd shows that the number 1 is pre-
sent in this expression. Halving the number twice
(ignoring remainders) produces:
37 = 32 + 4 + 1 →16 + 2 →8 + 1 = 9
That the answer is odd indicates that 4 is present in the
expression for 37 as a sum of powers of two. That
halving three more times produces the next odd answer
(corresponding to 37 halved five times in all) indicates
that 32 is also present. Thus, the appearance of odd
numbers in the left column indicates which powers of
two are used to build the number 37. For the multipli-
cation, note that:
37 ×23 = (32 + 4 + 1) ×23
= 32 ×23 + 4 ×23 + 1 ×23
= 25×23 + 22×23 + 20×23
Thus the desired product is obtained by summing
the effect of doubling the number 23 zero, two,
and five times. This is accomplished in step 4 of the
procedure.
This method of course works for any pair of whole
numbers you wish to multiply. It is an efficient method
of multiplication, and many computers are programmed
to compute products this way.
See also E
GYPTIAN MULTIPLICATION
; E
LIZABETHAN
MULTIPLICATION
;
FINGER MULTIPLICATION
;
MULTIPLICA
-
TION
; N
APIER
’
S BONES
.
454 Russian multiplication
Russian multiplication