Thales of Miletus 497

Tessellations

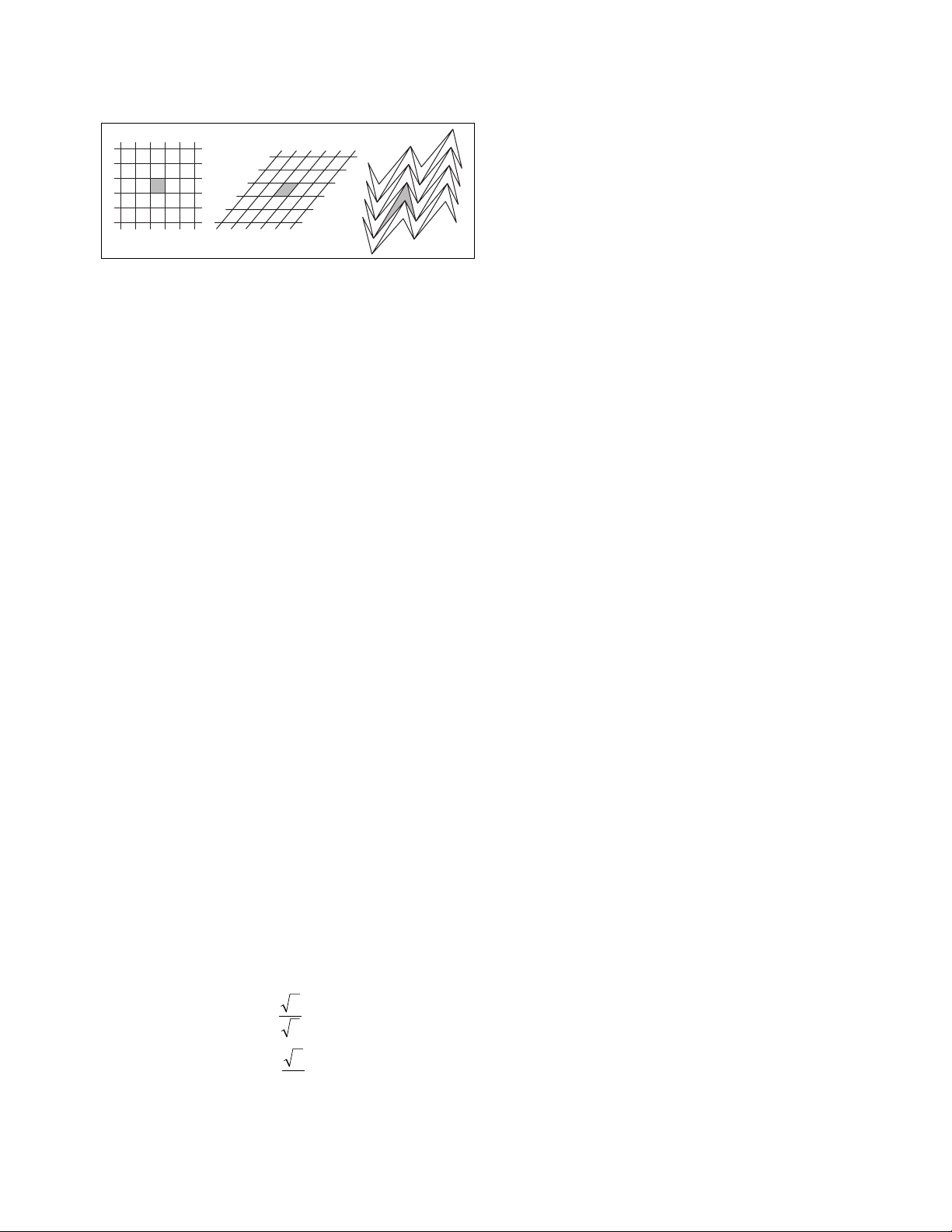
a regular polygon). The diagram above shows that

any

PARALLELOGRAM

tiles the plane. As two copies of

the same triangle fit together to form a parallelogram,

we have:

Any triangle provides a monohedral tessella-

tion of the plane.

By distorting the monohedral tessellation with parallel-

ograms, one can show:

Any quadrilateral, concave or convex, provides

a monohedral tessellation of the plane.

Not every pentagon or every hexagon will tile the

plane. In his 1918 doctoral thesis, mathematician Karl

Reinhardt (1895–1941) classified those hexagons that

do tile and found that they fall into three basic types. To

this day no one knows how many classes of convex

pentagons tessellate the plane. (Fourteen types have cur-

rently been identified.) Reinhardt proved that no con-

vex polygon with seven or more sides will tile the plane.

A tessellation of the plane using two

SQUARE

s of

different sizes provides a surprisingly elegant visual

proof of P

YTHAGORAS

’

S THEOREM

.

tetrahedron (triangular pyramid) Any solid figure

(

POLYHEDRON

) with four triangular faces is called a

tetrahedron. If the four faces are congruent equilateral

triangles, then the figure is called regular.

The height hand volume Vof a regular tetrahe-

dron with edge length aare given by:

and such a regular tetrahedron fits snugly inside a cube

of side-length . (Choose a vertex of the cube and

draw diagonals on the three faces surrounding that

vertex. These coincide with the three edges of an

entrapped tetrahedron.)

Although it is possible to stack a finite number of

small cubes together to form a larger cube, it is impos-

sible to complete a similar feat for regular tetrahedra:

no regular tetrahedron is a union of smaller regular

tetrahedra.

The sum of the first n

TRIANGULAR NUMBERS

is

called the nth tetrahedral number. For example, the

fifth tetrahedral number is 1 + 3 + 6 + 10 + 15 = 35.

If we place triangular arrays of 1, 3, 6, 10, and 15

dots above one another making use of the third

dimension in space, the array of dots produced is a

tetrahedron, explaining the name of these

FIGURATE

NUMBERS

. The general formula for the nth tetrahedral

number is n(n+ 1)(n+ 2). The tetrahedral numbers

appear as one of the diagonals of P

ASCAL

’

S TRIANGLE

.

See also

NET

; P

LATONIC SOLID

.

Thales of Miletus (ca. 625–547

B

.

C

.

E

.) Greek Geom-

etry, Philosophy Born in the region of Miletus, Asia

Minor (now Turkey), Thales is considered the first sci-

entist and philosopher of Western history, at least in the

sense of being the first scholar to whom particular

scientific and mathematical discoveries have been

attributed. Rather than relying on mythology and reli-

gion to explain the natural world, Thales searched for

rational principles in science. This work led him to also

look for unifying principles in geometry and, as such, he

was the first scholar to attempt to derive geometric facts

by processes of deduction and logical reasoning. He

established, for example, fundamental geometrical

propositions such as “the base angles of any isosceles

triangle are equal” and “the angle in a semicircle is a

right angle.”

Little is known of Thales’s life, and all his written

texts have been lost. Nonetheless, scholars that fol-

lowed Thales made numerous references to his achieve-

ments and to his approach to the study of the world.

The Greek philosopher P

ROCLUS

(ca. 450

C

.

E

.) claimed

that Thales acquired his mathematical knowledge from

Egyptian scholars, and centuries later, Hieronymus, a

student of Aristotle, wrote that Thales measured the

1

–

6

a

––

√

–

2