torus can be considered the C

ARTESIAN PRODUCT

of

two circles: each point on a torus corresponds to a pair

of points, one on one circle (the generating circle) and a

second on a second circle (the circle traced out by that

point on the generating circle). The set of all possible

positions of an hour hand and minute hand on a clock,

each corresponding to a full circle of values, describes a

set of outcomes topologically equivalent to a torus.

(Physicists would say that the “phase space” of the two

hands of a clock is a torus.)

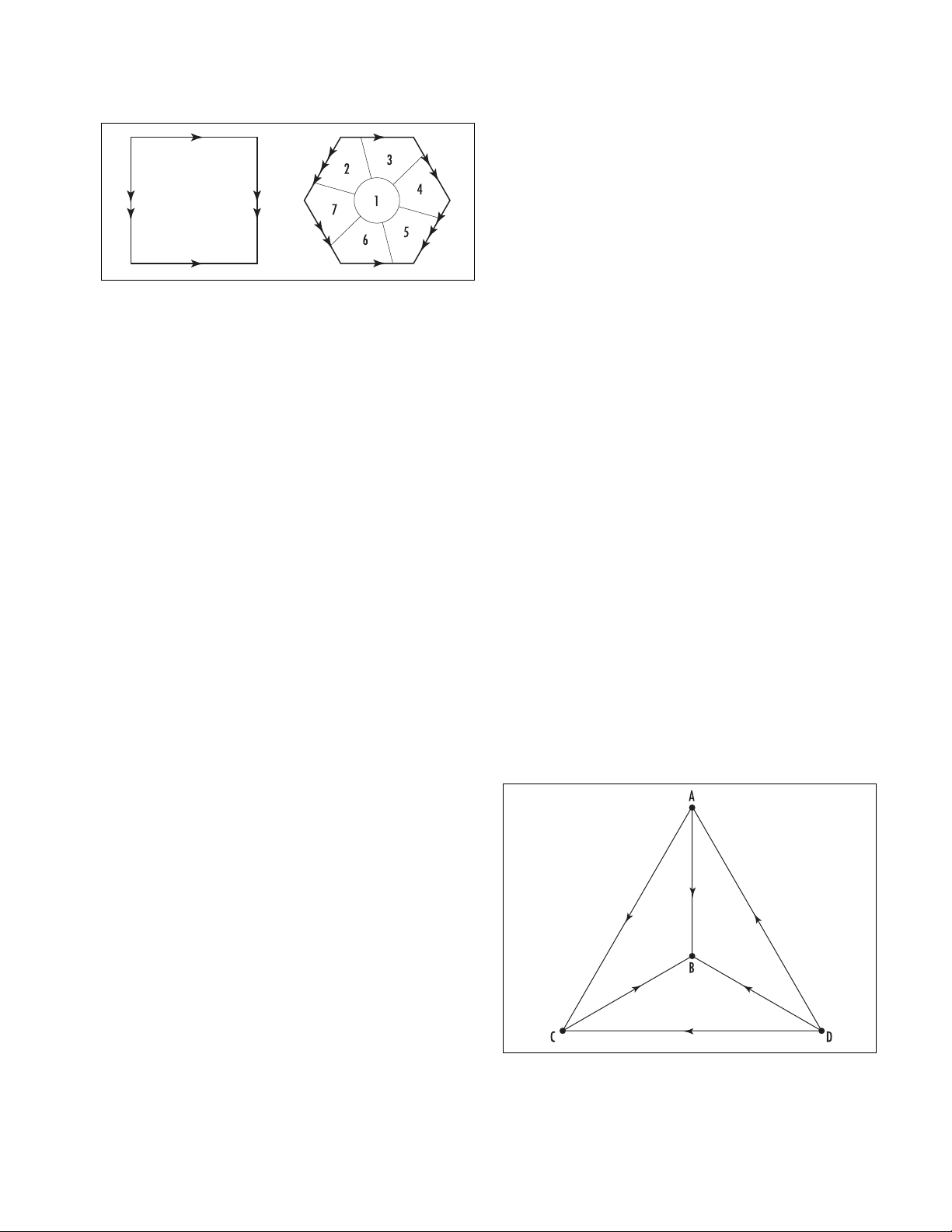
One can physically construct a torus by sewing

together the opposite sides of a square rubber sheet as

shown. (One must be sure to align the directions of the

arrows indicated.) Sewing together the opposite sides

of a hexagon also produces a torus.

This latter construct shows that it is possible to

produce a design of seven regions on a torus so that

each regions shares a portion of boundary with each of

the six remaining regions. Thus some maps on a torus

require seven colors to paint if one wishes to ensure

that neighboring regions are always of distinct colors.

Mathematicians have proved that the situation is never

worse than this: seven colors will always suffice to

color a toroidal map, no matter how complicated that

map may be.

Given a punctured torus made of rubber, such as

an inner tube with a small hole, it is physically possible

to stretch open the hole and turn the surface inside out

through that hole. Surprisingly, the resulting surface is

another torus. A double torus is a closed surface with

two holes. Everting a double torus through a puncture

in its surface again yields the same surface back again,

another double torus, and the same result is true of

triple tori and multiple tori with any number of holes.

See also

FOUR

-

COLOR THEOREM

;

SPHERE

.

tournament (round-robin tournament, complete di-

graph) A competition among a collection of teams is

called a tournament if each possible pair of teams

plays exactly one match, and each game played results

in a win for one team and a loss for the other. (That is,

no ties are permitted.) Mathematically, a tournament

among nplayers is a

GRAPH

with nvertices, with edges

drawn between every possible pair of points, directed

with arrows pointing from one vertex to another. Here

each vertex represents a team, and an edge pointing

from vertex Ato vertex Bindicates a win for team A

over team B. The diagram below, for instance, repre-

sents a tournament among four teams, A, B, C, and D.

We see that team Alost to team Dbut beat teams B

and C.

A graph on nvertices with an edge between every

possible pair of vertices directed by an arrow is called a

complete directed graph or simply a complete digraph

on nvertices.

Structurally, there is essentially only one possible

complete digraph on two vertices (although, in terms

of team-playing, there are two possible interpreta-

tions: either a team Abeat a team B, or team Bbeat

team A). For nequal to 3, 4, 5, 6, and 7 there are,

respectively, 2, 4, 12, 56, and 456 different complete

digraphs on nvertices.

Any team that beat every other team in a tourna-

ment is called a source or a transmitter, and any team

that lost to all other teams is called a sink or a receiver.

(These names reflect the alternative interpretation of a

tournament 501

Constructing a torus

Representing a tournament