单词

linear differential equation with constant coefficients

释义

linear differential equation with constant coefficients

For simplicity, consider such an equation of second‐order,

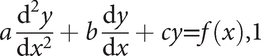
where a, b, and c are given constants and f is a given function. (Higher‐order equations can be treated similarly.) Suppose that f is not the zero function. Then the equation

is the homogeneous equation that corresponds to the inhomogeneous equation 1. The two are connected by the following result:

Theorem

If y = G(x) is the general solution of 2 and y = y1(x) is a particular solution of 1, then y = G(x) + y1(x) is the general solution of 1.

Thus the problem of solving 1 is reduced to the problem of finding the complementary function (C.F.) G(x), which is the general solution of 2, and a particular solution y1(x) of 1, usually known in this context as a particular integral (P.I.).

The complementary function is found by looking for solutions of 2 of the form y = e^mx and obtaining the auxiliary equation am2 + bm + c = 0. If this equation has distinct real roots m1 and m2, the C.F. is $inline$ if it has one (repeated) root m, the C.F. is y = (A + Bx)e^mx; if it has non‐real roots α ± βi, the C.F. is y = e^αx(Acosβx + Bsinβx).

The most elementary way of obtaining a particular integral is to try something similar in form to f(x). Thus, if f(x) = e^kx, try as the P.I. y1(x) = pe^kx. If f(x) is a polynomial in x, try a polynomial of the same degree. If f(x) = coskx or sinkx, try y1(x) = pcoskx + qsinkx. In each case, the values of the unknown coefficients are found by substituting the possible P.I. into the equation 1. If f(x) is the sum of two terms, a P.I. corresponding to each may be found and the two added together.

For example, the general solution of y″−3y′+2y = 4x + e^3x, is found to be $inline$

随便看

数学辞典收录了4151条数学词条，基本涵盖了常用数学知识及数学英语单词词组的翻译及用法，是数学学习的有利工具。