“hyperbola”的概念、定义、习题解题方法及翻译-数学辞典

单词

hyperbola

释义

hyperbola

A conic with eccentricity greater than 1. Thus it is the locus of all points P such that the distance from P to a fixed point F1 (the focus) is equal to e (>1) times the distance from P to a fixed line l1 (the directrix). It turns out that there is another point F2 and another line l2 such that the same set of points would be obtained with these as focus and directrix. The hyperbola is also the conic section that results when a plane cuts a (double) cone in such a way that a section in two separate parts is obtained.

The line through F1 and F2 is the transverse axis, and the points V1 and V2 where it cuts the hyperbola are the vertices. The length |V1V2| is the length of the transverse axis and is usually taken to be 2a. The midpoint of V1V2 is the centre of the hyperbola. The line through the centre perpendicular to the transverse axis is the conjugate axis. It is usual to introduce b (>0) defined by b2 = a2(e2−1), so that e2=1 + b2/a2. The two separate parts of the hyperbola are the two branches.

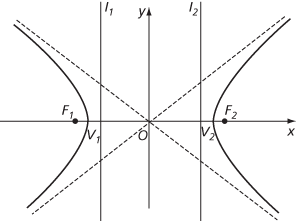
A hyperbola in normal form

If a coordinate system is taken with origin at the centre of the hyperbola, and x‐axis along the transverse axis, the foci have coordinates (ae, 0) and (−ae, 0), the directrices have equations x = a/e and x = −a/e, and the hyperbola's equation has the normal form

The hyperbola can be parametrized by x = asecθ, y = btanθ (0 ≤ θ<2π, θ ≠ π/2, 3π/2) or alternatively by x = acosh t, y = bsinht (tϵR) (see hyperbolic function), but this gives only one branch of the hyperbola.

The hyperbola, in normal form, has two asymptotes, y = (b/a)x and y = (−b/a)x. The shape of the hyperbola is determined by the eccentricity. The particular value 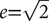$inline$ is important for this gives b = a. Then the asymptotes are perpendicular, and the hyperbola is called a rectangular hyperbola.

随便看

数学辞典收录了4151条数学词条，基本涵盖了常用数学知识及数学英语单词词组的翻译及用法，是数学学习的有利工具。