hypergeometric distribution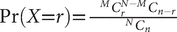 where r runs from 0 up to the smaller of M and n. This is called a hypergeometric distribution. The mean is
where r runs from 0 up to the smaller of M and n. This is called a hypergeometric distribution. The mean is 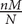 , and the variance is
, and the variance is
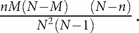
- Chebyshev, Pafnuty Lvovich
- Chebyshev polynomials
- Chebyshev's inequality
- Chebyshev's Theorem
- check digit
- Chen's theorem
- Chinese postman problem
- Chinese remainder theorem
- chirality
- chi-squared contingency table test
- chi-squared distribution
- chi-squared test
- Cholesky's decomposition
- chord
- Christoffel symbol
- chromatic number
- Church, Alonzo
- Church's thesis
- Chu Shih-chieh
- cipher
- cipher
- ciphertext
- circle
- circle of convergence
- circle of curvature