Stirling Number of the Second Kind
The number of ways of partitioning a set of 
elements into 
nonempty Sets (i.e., Blocks), also called a Stirling Set Number. For example, the Set 
can be partitioned intothree Subsets in one way: 
; into two Subsets in three ways:
, 
, and 
; and into one Subset in one way: 
.
The Stirling numbers of the second kind are denoted 
, 
, 
, or 
, so the Stirling numbers of the second kind for three elements are
 |  |  | (1) |
 | |  | (2) |
 | |  | (3) |
Since a set of
elements can only be partitioned in a single way into 1 or Subsets, | (4) |
 | |
 | |
 | |
 | |
 | |
 |
The Stirling numbers of the second kind can be computed from the sum
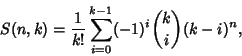 | (5) |
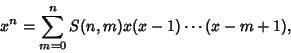a Binomial Coefficient, or the Generating Functions | (6) |
 | (7) |
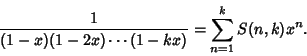 | (8) |
The following diagrams (Dickau) illustrate the definition of the Stirling numbers of the second kind for 
and 4.
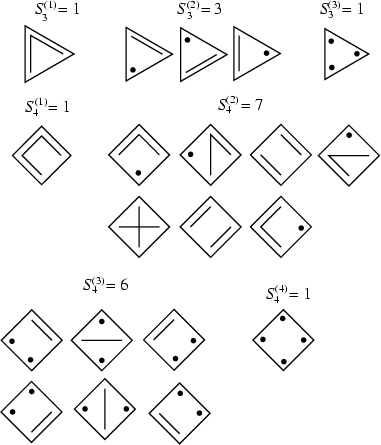
Stirling numbers of the second kind obey the Recurrence Relations
 | (9) |
An identity involving Stirling numbers of the second kind is
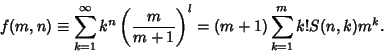 | (10) |
can have only 0, 2, or 6 as a last Digit (Riskin 1995).See also Bell Number, Combination Lock, Lengyel's Constant, Minimal Cover, Stirling Number ofthe First Kind
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Stirling Numbers of the Second Kind.'' §24.1.4 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 824-825, 1972. Comtet, L. Advanced Combinatorics: The Art of Finite and Infinite Expansions, rev. enl. ed. Boston, MA: Reidel, 1974. Conway, J. H. and Guy, R. K. In The Book of Numbers. New York: Springer-Verlag, pp. 91-92, 1996. Dickau, R. M. ``Stirling Numbers of the Second Kind.'' Graham, R. L.; Knuth, D. E.; and Patashnik, O. Concrete Mathematics: A Foundation for Computer Science, 2nd ed. Reading, MA: Addison-Wesley, 1994. Knuth, D. E. ``Two Notes on Notation.'' Amer. Math. Monthly 99, 403-422, 1992. Riordan, J. An Introduction to Combinatorial Analysis. New York: Wiley, 1980. Riordan, J. Combinatorial Identities. New York: Wiley, 1968. Riskin, A. ``Problem 10231.'' Amer. Math. Monthly 102, 175-176, 1995. Sloane, N. J. A. Sequence A008277in ``The On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html. Stanley, R. P. Enumerative Combinatorics, Vol. 1. Cambridge, England: Cambridge University Press, 1997.
http://forum.swarthmore.edu/advanced/robertd/stirling2.html
- Cauchy Distribution
- Cauchy Equation
- Cauchy Functional Equation
- Cauchy-Hadamard Theorem
- Cauchy Inequality
- Cauchy Integral Formula
- Cauchy Integral Test
- Cauchy Integral Theorem
- Cauchy-Kovalevskaya Theorem
- Cauchy-Lagrange Identity
- Cauchy-Maclaurin Theorem
- Cauchy Mean Theorem
- Cauchy Principal Value
- Cauchy Problem
- Cauchy Ratio Test
- Cauchy Remainder Form
- Cauchy-Riemann Equations
- Cauchy Root Test
- Cauchy-Schwarz Integral Inequality
- Cauchy-Schwarz Sum Inequality
- Cauchy's Cosine Integral Formula
- Cauchy Sequence
- Cauchy's Formula
- Cauchy's Rigidity Theorem
- Cauchy Test