Platonic Solid

A solid with equivalent faces composed of congruent regular convex Polygons. There are exactly five suchsolids: the Cube, Dodecahedron, Icosahedron, Octahedron, and Tetrahedron, as was proved byEuclid in the last proposition of the Elements">Elements.

The Platonic solids were known to the ancient Greeks, and were described by Plato in his Timaeus ca. 350BC . In this work, Plato equated the Tetrahedron with the ``element'' fire, the Cube with earth, theIcosahedron with water, the Octahedron with air, and the Dodecahedron with the stuff of which theconstellations and heavens were made (Cromwell 1997).

The Platonic solids are sometimes also known as the Regular Polyhedra of CosmicFigures (Cromwell 1997), although the former term is sometimes used to refer collectively to both the Platonicsolids and Kepler-Poinsot Solids (Coxeter 1973).

If is a Polyhedron with congruent (convex) regular polygonal faces, then Cromwell (1997, pp. 77-78) shows thatthe following statements are equivalent.

1. The vertices of 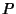all lie on a Sphere.

2. All the Dihedral Angles are equal.

3. All the Vertex Figures are Regular Polygons.

4. All the Solid Angles are equivalent.

5. All the vertices are surrounded by the same number of Faces.

Let (sometimes denoted ) be the number of Vertices, (or ) the number ofEdges, and (or ) the number of Faces. The following table gives theSchläfli Symbol, Wythoff Symbol, and C&R symbol, the number of vertices , edges ,and faces , and the Point Groups for the Platonic solids (Wenninger 1989).

Solid	Schläfli Symbol	Wythoff Symbol	C&R Symbol				Group
Cube		3 2 4		8	12	6
Dodecahedron		3 2 5		20	30	12
Icosahedron		5 2 3		12	30	20
Octahedron		4 2 3		6	12	8
Tetrahedron		3 2 3		4	6	4

Let be the Inradius, the Midradius, and the Circumradius. The following two tables givethe analytic and numerical values of these distances for Platonic solids with unit side length.

Solid
Cube
Dodecahedron
Icosahedron
Octahedron
Tetrahedron

Solid
Cube	0.5	0.70711	0.86603
Dodecahedron	1.11352	1.30902	1.40126
Icosahedron	0.75576	0.80902	0.95106
Octahedron	0.40825	0.5	0.70711
Tetrahedron	0.20412	0.35355	0.61237

Finally, let be the Area of a single Face, be the Volume of the solid, the Edges be of unit length on a side, and be the Dihedral Angle. The following table summarizes thesequantities for the Platonic solids.

Solid
Cube	1	1
Dodecahedron
Icosahedron
Octahedron
Tetrahedron

The number of Edges meeting at a Vertex is . TheSchläfli Symbol can be used to specify a Platonic solid. For the solid whose faces are-gons (denoted ), with touching at each Vertex, the symbol is . Given and , the number of Vertices, Edges, and faces are given by

Minimal Surfaces for Platonic solid frames are illustrated in Isenberg (1992, pp. 82-83).

References

Artmann, B. ``Symmetry Through the Ages: Highlights from the History of Regular Polyhedra.'' In In Eves' Circles (Ed. J. M. Anthony). Washington, DC: Math. Assoc. Amer., pp. 139-148, 1994.

Ball, W. W. R. and Coxeter, H. S. M. ``Polyhedra.'' Ch. 5 in Mathematical Recreations and Essays, 13th ed. New York: Dover, pp. 131-136, 1987.

Behnke, H.; Bachman, F.; Fladt, K.; and Kunle, H. (Eds.). Fundamentals of Mathematics, Vol. 2: Geometry. Cambridge, MA: MIT Press, p. 272, 1974.

Beyer, W. H. (Ed.) CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, pp. 128-129, 1987.

Bogomolny, A. ``Regular Polyhedra.'' http://www.cut-the-knot.com/do_you_know/polyhedra.html.

Coxeter, H. S. M. Regular Polytopes, 3rd ed. New York: Dover, pp. 1-17, 93, and 107-112, 1973.

Critchlow, K. Order in Space: A Design Source Book. New York: Viking Press, 1970.

Cromwell, P. R. Polyhedra. New York: Cambridge University Press, pp. 51-57, 66-70, and 77-78, 1997.

Dunham, W. Journey Through Genius: The Great Theorems of Mathematics. New York: Wiley, pp. 78-81, 1990.

Gardner, M. ``The Five Platonic Solids.'' Ch. 1 in The Second Scientific American Book of Mathematical Puzzles & Diversions: A New Selection. New York: Simon and Schuster, pp. 13-23, 1961.

Heath, T. A History of Greek Mathematics, Vol. 1: From Thales to Euclid. New York: Dover, p. 162, 1981.

Isenberg, C. The Science of Soap Films and Soap Bubbles. New York: Dover, 1992.

Kepler, J. Opera Omnia, Vol. 5. Frankfort, p. 121, 1864.

Ogilvy, C. S. Excursions in Geometry. New York: Dover, pp. 129-131, 1990.

Pappas, T. ``The Five Platonic Solids.'' The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, pp. 39 and 110-111, 1989.

Rawles, B. A. ``Platonic and Archimedean Solids--Faces, Edges, Areas, Vertices, Angles, Volumes, Sphere Ratios.'' http://www.intent.com/sg/polyhedra.html.

Steinhaus, H. ``Platonic Solids, Crystals, Bees' Heads, and Soap.'' Ch. 8 in Mathematical Snapshots, 3rd American ed. New York: Oxford University Press, 1960.

Waterhouse, W. ``The Discovery of the Regular Solids.'' Arch. Hist. Exact Sci. 9, 212-221, 1972-1973.

Wenninger, M. J. Polyhedron Models. Cambridge, England: Cambridge University Press, 1971.

• Elliptic Fixed Point (Map)
• Elliptic Function
• Elliptic Functional
• Elliptic Geometry
• Elliptic Group Modulo p
• Elliptic Helicoid
• Elliptic Hyperboloid
• Elliptic Integral
• Elliptic Integral of the First Kind
• Elliptic Integral of the Second Kind
• Elliptic Integral of the Third Kind
• Elliptic Integral Singular Value
• Elliptic Integral Singular Value k1
• Elliptic Integral Singular Value k2
• Elliptic Integral Singular Value k3
• Ellipticity
• Elliptic Lambda Function
• Elliptic Logarithm
• Elliptic Modular Function
• Elliptic Paraboloid
• Elliptic Partial Differential Equation
• Elliptic Plane
• Elliptic Point
• Elliptic Pseudoprime
• Elliptic Rotation