Complete Functions
A set of Orthonormal Functions 
is termed complete in the Closed Interval 
if, forevery piecewise Continuous Function 
in the interval, the minimum square error
(where
denotes the Norm) converges to zero as 
becomes infinite. Symbolically, a set of functions iscomplete if
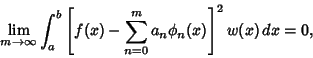
where
is a Weighting Function and the above is a Lebesgue Integral.See also Bessel's Inequality, Hilbert Space
References
Arfken, G. ``Completeness of Eigenfunctions.'' §9.4 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 523-538, 1985.
- Brouwer Fixed Point Theorem
- Browkin's Theorem
- Brown Function
- Brown Numbers
- Brown's Criterion
- Broyden's Method
- Bruck-Ryser-Chowla Theorem
- Bruck-Ryser Theorem
- Brunnian Link
- Brunn-Minkowski Inequality
- Brun's Constant
- Brun's Sum
- Brun's Theorem
- Brute Force Factorization
- B-Spline
- B-Tree
- Bubble
- Buchberger's Algorithm
- Buckminster Fuller Dome
- Buffon-Laplace Needle Problem
- Buffon's Needle Problem
- Bulirsch-Stoer Algorithm
- Bullet Nose
- Bumping Algorithm
- Bundle