One-Way Function

Consider straight-line algorithms over a Finite Field with elements. Then the -straight linecomplexity of a function is defined as the length of the shortest straight-line algorithm which computesa function such that is satisfied for at least elements of . A function isstraight-line ``one way'' of range if satisfies the properties:

1. There exists an infinite set 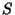of finite fields such that is defined in every and isOne-to-One in every .

2. For every such that , tends to infinity as the cardinality of approaches infinity.

3. For every such that , the ``work function'' satisfies

References

Ziv, J. ``In Search of a One-Way Function'' §4.1 in Open Problems in Communication and Computation (Ed. T. M. Cover and B. Gopinath). New York: Springer-Verlag, pp. 104-105, 1987.

• Barth Decic
• Barth Sextic
• Bartlett Function
• Barycentric Coordinates
• Baseball
• Baseball Cover
• Base Curve
• Base (Logarithm)
• Base (Neighborhood System)
• Base (Number)
• Base Space
• Basin of Attraction
• Basis
• Basis Theorem
• Basler Problem
• Basset Function
• Batch
• Bateman Function
• Batrachion
• Bauer's Identical Congruence
• Bauer's Theorem
• Bauspiel
• Bayes' Formula
• Bayesian Analysis
• Bayes' Theorem