Norm
Given a ![]() -D Vector
-D Vector
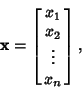
a Vector Norm
- 1.
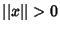 when
when 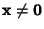 and
and 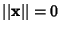 Iff
Iff 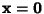 ,
, - 2.
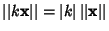 for any Scalar
for any Scalar  ,
, - 3.
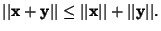
Given a Square Matrix ![]() , a Matrix Norm
, a Matrix Norm ![]() is a Nonnegative number associated with
is a Nonnegative number associated with ![]() having the properties
having the properties
- 1.
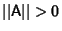 when
when 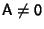 and
and 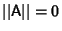 Iff
Iff  ,
, - 2.
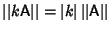 for any Scalar
for any Scalar  ,
, - 3.
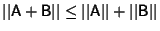 ,
, - 4.
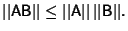
References
Gradshteyn, I. S. and Ryzhik, I. M. Tables of Integrals, Series, and Products, 5th ed. San Diego, CA: Academic Press, pp. 1114-1125, 1979.
- Conformal Solution
- Conformal Tensor
- Conformal Transformation
- Congruence
- Congruence Axioms
- Congruence (Geometric)
- Congruence Transformation
- Congruent
- Congruent Incircles Point
- Congruent Isoscelizers Point
- Congruent Numbers
- Congruum
- Congruum Problem
- Conic
- Conical Coordinates
- Conical Frustum
- Conical Function
- Conical Spiral
- Conical Wedge
- Conic Constant
- Conic Double Point
- Conic Equidistant Projection
- Conic Projection
- Conic Section
- Conic Section Tangent