Concentric Circles
The region between two Concentric circles of different Radii is called an Annulus.
Given two concentric circles with Radii 
and 
, what is the probability that a chord chosen atrandom from the outer circle will cut across the inner circle? Depending on how the ``random'' Chord is chosen,1/2, 1/3, or 1/4 could all be correct answers.
- 1. Picking any two points on the outer circle and connecting them gives 1/3.
- 2. Picking any random point on a diagonal and then picking the Chord that perpendicularly bisects it gives 1/2.
- 3. Picking any point on the large circle, drawing a line to the center, and then drawing the perpendicularly bisected Chord gives 1/4.
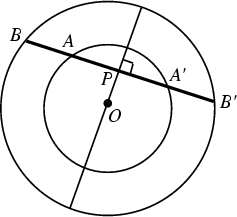
Given an arbitrary Chord 
to the larger of two concentric Circles centered on 
, thedistance between inner and outer intersections is equal on both sides 
. To prove this, take thePerpendicular to passing through and crossing at 
. By symmetry, it must be true that 
and 
are equal. Similarly, 
and 
must be equal. Therefore, 
equals 
. Incidentally, thisis also true for Homeoids, but the proof is nontrivial.
- Spencer's 15-Point Moving Average
- Spence's Function
- Spence's Integral
- Sperner's Theorem
- Sphenocorona
- Sphenoid
- Sphenomegacorona
- Sphere
- Sphere-Cylinder Intersection
- Sphere Embedding
- Sphere Eversion
- Sphere Geodesic
- Sphere Packing
- Sphere Point Picking
- Sphere-Sphere Intersection
- Sphere with Tunnel
- Spherical Bessel Differential Equation
- Spherical Bessel Function
- Spherical Bessel Function of the First Kind
- Spherical Bessel Function of the Second Kind
- Spherical Bessel Function of the Third Kind
- Spherical Cap
- Spherical Coordinates
- Spherical Design
- Spherical Excess