| 释义 |
Cyclotomic PolynomialA polynomial given by
 | (1) |
 are the primitive are the primitive 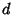 th Roots of Unity in th Roots of Unity in  given by given by 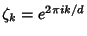 . The numbers . The numbers 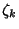 are sometimes called de Moivre Numbers. are sometimes called de Moivre Numbers. 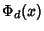 is anirreducible Polynomial in is anirreducible Polynomial in  with degree with degree  , where , where 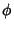 is the Totient Function. For is the Totient Function. For 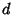 Prime, Prime,
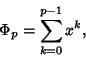 | (2) |
 has coefficients of has coefficients of  for for 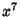 and and  , making it the firstcyclotomic polynomial to have a coefficient other than , making it the firstcyclotomic polynomial to have a coefficient other than 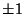 and 0. This is true because 105 is the first number to havethree distinct Odd Prime factors, i.e., and 0. This is true because 105 is the first number to havethree distinct Odd Prime factors, i.e.,  (McClellan and Rader 1979, Schroeder 1997). Migotti (1883)showed that Coefficients of (McClellan and Rader 1979, Schroeder 1997). Migotti (1883)showed that Coefficients of  for for 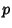 and and 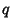 distinct Primes can be only 0, distinct Primes can be only 0, 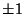 . Lam and Leung (1996) considered . Lam and Leung (1996) considered
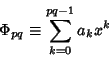 | (3) |
 Prime. Write the Totient Function as Prime. Write the Totient Function as
 | (4) |
 | (5) |
- 1.
 Iff Iff 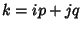 for some for some 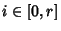 and and  , , - 2.
 Iff Iff  for for  and and  , , - 3. otherwise
 . .
The number of terms having  is is  , and the number of terms having , and the number of terms having  is is . Furthermore, assume . Furthermore, assume  , then the middle Coefficient of , then the middle Coefficient of  is is  . .
The Logarithm of the cyclotomic polynomial
 | (6) |
The first few cyclotomic Polynomials are
The smallest values of 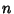 for which for which  has one or more coefficients has one or more coefficients 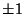 , ,  , ,  , ... are 0, 105, 385, 1365, 1785, 2805, 3135, 6545, 6545, 10465, 10465, 10465, 10465, 10465, 11305, ... (Sloane's A013594). , ... are 0, 105, 385, 1365, 1785, 2805, 3135, 6545, 6545, 10465, 10465, 10465, 10465, 10465, 11305, ... (Sloane's A013594).
The Polynomial 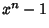 can be factored as can be factored as
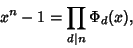 | (7) |
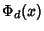 is a Cyclotomic Polynomial. Furthermore, is a Cyclotomic Polynomial. Furthermore,
 | (8) |
can also be computed from
 | (10) |
 is the Floor Function.See also Aurifeuillean Factorization, Möbius Inversion Formula is the Floor Function.See also Aurifeuillean Factorization, Möbius Inversion Formula
References
Beiter, M. ``The Midterm Coefficient of the Cyclotomic Polynomial 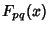 .'' Amer. Math. Monthly 71, 769-770, 1964. .'' Amer. Math. Monthly 71, 769-770, 1964.Beiter, M. ``Magnitude of the Coefficients of the Cyclotomic Polynomial 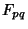 .'' Amer. Math. Monthly 75, 370-372, 1968. .'' Amer. Math. Monthly 75, 370-372, 1968. Bloom, D. M. ``On the Coefficients of the Cyclotomic Polynomials.'' Amer. Math. Monthly 75, 372-377, 1968. Carlitz, L. ``The Number of Terms in the Cyclotomic Polynomial 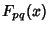 .'' Amer. Math. Monthly 73, 979-981, 1966. .'' Amer. Math. Monthly 73, 979-981, 1966. Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, 1996. de Bruijn, N. G. ``On the Factorization of Cyclic Groups.'' Indag. Math. 15, 370-377, 1953. Lam, T. Y. and Leung, K. H. ``On the Cyclotomic Polynomial  .'' Amer. Math. Monthly 103, 562-564, 1996. .'' Amer. Math. Monthly 103, 562-564, 1996. Lehmer, E. ``On the Magnitude of Coefficients of the Cyclotomic Polynomials.'' Bull. Amer. Math. Soc. 42, 389-392, 1936. McClellan, J. H. and Rader, C. Number Theory in Digital Signal Processing. Englewood Cliffs, NJ: Prentice-Hall, 1979. Migotti, A. ``Zur Theorie der Kreisteilungsgleichung.'' Sitzber. Math.-Naturwiss. Classe der Kaiser. Akad. der Wiss., Wien 87, 7-14, 1883. Schroeder, M. R. Number Theory in Science and Communication, with Applications in Cryptography, Physics, Digital Information, Computing, and Self-Similarity, 3rd ed. New York: Springer-Verlag, p. 245, 1997. Sloane, N. J. A. Sequence A013594in ``The On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html. Vardi, I. Computational Recreations in Mathematica. Redwood City, CA: Addison-Wesley, pp. 8 and 224-225, 1991. |

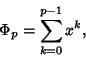
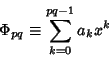
 Iff
Iff 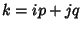 for some
for some 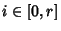 and
and  ,
, Iff
Iff  for
for  and
and  ,
, .
.![]() can be factored as
can be factored as

![]() .'' Amer. Math. Monthly 71, 769-770, 1964.
.'' Amer. Math. Monthly 71, 769-770, 1964.![]() .'' Amer. Math. Monthly 75, 370-372, 1968.
.'' Amer. Math. Monthly 75, 370-372, 1968.![]() .'' Amer. Math. Monthly 73, 979-981, 1966.
.'' Amer. Math. Monthly 73, 979-981, 1966.![]() .'' Amer. Math. Monthly 103, 562-564, 1996.
.'' Amer. Math. Monthly 103, 562-564, 1996.