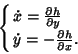| 释义 |
Duffing Differential EquationThe most general forced form of the Duffing equation is
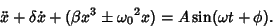 | (1) |
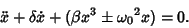 | (2) |
 and we take the plus sign, and we take the plus sign,
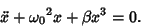 | (3) |
 , the equation represents a ``hard spring,'' and for , the equation represents a ``hard spring,'' and for 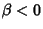 , it represents a ``soft spring.'' If , it represents a ``soft spring.'' If 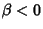 , the phase portrait curves are closed.Returning to (1),take , the phase portrait curves are closed.Returning to (1),take  , ,  , , 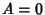 , and use the minus sign. Then the equation is , and use the minus sign. Then the equation is
 | (4) |
The fixed points of these differential equations
so 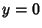 , and , and
giving 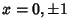 . Differentiating, . Differentiating,
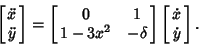 | (11) |
 | (12) |
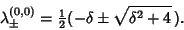 | (13) |
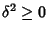 , so , so  is real. Since is real. Since 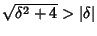 , there will always be onePositive Root, so this fixed point is unstable. Now look at ( , there will always be onePositive Root, so this fixed point is unstable. Now look at (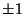 , 0). , 0).
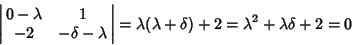 | (14) |
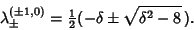 | (15) |
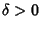 , , 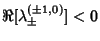 , so the point is asymptotically stable. If , so the point is asymptotically stable. If  , ,  , so the point is linearly stable. If , so the point is linearly stable. If  , the radical gives anImaginary Part and the Real Part is , the radical gives anImaginary Part and the Real Part is  , so the point is unstable. If , so the point is unstable. If 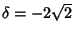 , , 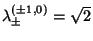 , which has a Positive Real Root, so the point is unstable. If , which has a Positive Real Root, so the point is unstable. If 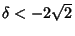 , then , then 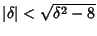 , so both Roots are Positive and the point is unstable. The following table summarizes these results. , so both Roots are Positive and the point is unstable. The following table summarizes these results.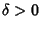 | asymptotically stable |  | linearly stable (superstable) | 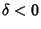 | unstable |
Now specialize to the case  , which can be integrated by quadratures.In this case, the equations become , which can be integrated by quadratures.In this case, the equations become
Differentiating (16) and plugging in (17) gives
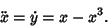 | (18) |
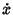 gives gives
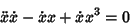 | (19) |
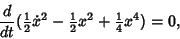 | (20) |
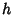 , ,
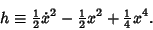 | (21) |
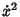 gives gives
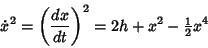 | (22) |
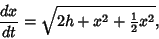 | (23) |
 | (24) |
Note that the invariant of motion 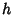 satisfies satisfies
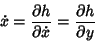 | (25) |
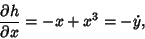 | (26) |
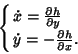 | (27) |
References
Ott, E. Chaos in Dynamical Systems. New York: Cambridge University Press, 1993.
|
![]() , which can be integrated by quadratures.In this case, the equations become
, which can be integrated by quadratures.In this case, the equations become
![]() satisfies
satisfies