Zero
The Integer denoted 0 which, when used as a counting number, means that no objects are present. It is the onlyInteger (and, in fact, the only Real Number) which is neither Negative nor Positive. A number which is not zero is said to be Nonzero.
Because the number of Permutations of 0 elements is 1, 0! (zero Factorial) is often defined as1. This definition is useful in expressing many mathematical identities in simple form. A number other than 0 takento the Power 0 is defined to be 1. ![]() is undefined, but defining
is undefined, but defining ![]() allows concise statement of the beautifulanalytical formula for the integral of the generalized Sinc Function
allows concise statement of the beautifulanalytical formula for the integral of the generalized Sinc Function
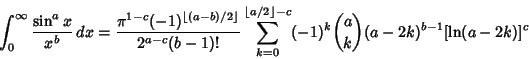
given by Kogan, where
The following table gives the first few numbers ![]() such that teh decimal expansion of
such that teh decimal expansion of ![]() contains no zeros, for small
contains no zeros, for small ![]() . The largest known
. The largest known ![]() for which
for which ![]() contain no zeros is 86 (Madachy 1979), with no other
contain no zeros is 86 (Madachy 1979), with no other ![]() (M. Cook),improving the
(M. Cook),improving the ![]() limit obtained by Beeler et al. (1972). The values
limit obtained by Beeler et al. (1972). The values ![]() such that the positions of theright-most zero in
such that the positions of theright-most zero in ![]() increases are 10, 20, 30, 40, 46, 68, 93, 95, 129, 176, 229, 700, 1757, 1958, 7931, 57356,269518, ... (Sloane's A031140). The positions in which the right-most zeros occur are 2, 5, 8, 11, 12, 13, 14, 23, 36, 38,54, 57, 59, 93, 115, 119, 120, 121, 136, 138, 164, ... (Sloane's A031141). The right-most zero of
increases are 10, 20, 30, 40, 46, 68, 93, 95, 129, 176, 229, 700, 1757, 1958, 7931, 57356,269518, ... (Sloane's A031140). The positions in which the right-most zeros occur are 2, 5, 8, 11, 12, 13, 14, 23, 36, 38,54, 57, 59, 93, 115, 119, 120, 121, 136, 138, 164, ... (Sloane's A031141). The right-most zero of ![]() occurs atthe 217th decimal place, the farthest over for powers up to
occurs atthe 217th decimal place, the farthest over for powers up to ![]() .
.
| Sloane | ||
| 2 | Sloane's A007377 | 1, 2, 3, 4, 5, 6, 7, 8, 9, 13, 14, 15, 16, 18, 19, 24, 25, 27, 28, ... |
| 3 | Sloane's A030700 | 1, 2, 3, 4, 5, 6, 7, 8, 9, 11, 12, 13, 14, 19, 23, 24, 26, 27, 28, ... |
| 4 | Sloane's A030701 | 1, 2, 3, 4, 7, 8, 9, 12, 14, 16, 17, 18, 36, 38, 43, ... |
| 5 | Sloane's A008839 | 1, 2, 3, 4, 5, 6, 7, 9, 10, 11, 17, 18, 30, 33, 58, ... |
| 6 | Sloane's A030702 | 1, 2, 3, 4, 5, 6, 7, 8, 12, 17, 24, 29, 44, ... |
| 7 | Sloane's A030703 | 1, 2, 3, 6, 7, 10, 11, 19, 35 |
| 8 | Sloane's A030704 | 1, 2, 3, 5, 6, 8, 9, 11, 12, 13, 17, 24, 27 |
| 9 | Sloane's A030705 | 1, 2, 3, 4, 6, 7, 12, 13, 14, 17, 34 |
| 11 | Sloane's A030706 | 1, 2, 3, 4, 6, 7, 8, 9, 12, 13, 14, 15, 16, 18, 41, ... |
While it has not been proven that the numbers listed above are the only ones without zeros for a given base, the probabilitythat any additional ones exist is vanishingly small. Under this assumption, the sequence of largest ![]() such that
such that ![]() contains no zeros for
contains no zeros for ![]() , 3, ... is then given by 86, 68, 43, 58, 44, 35, 27, 34, 0, 41, ... (Sloane's A020665).
, 3, ... is then given by 86, 68, 43, 58, 44, 35, 27, 34, 0, 41, ... (Sloane's A020665).
References
Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, Item 57, Feb. 1972. Kogan, S. ``A Note on Definite Integrals Involving Trigonometric Functions.'' http://www.mathsoft.com/asolve/constant/pi/sin/sin.html. Madachy, J. S. Madachy's Mathematical Recreations. New York: Dover, pp. 127-128, 1979. Pappas, T. ``Zero-Where & When.'' The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, p. 162, 1989. Sloane, N. J. A. SequenceA007377/M0485in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
- Bhargava's Theorem
- Bhaskara-Brouckner Algorithm
- Bianchi Identities
- Bianchi Identities (Contracted)
- Biased
- Bias (Estimator)
- Bias (Series)
- Biaugmented Pentagonal Prism
- Biaugmented Triangular Prism
- Biaugmented Truncated Cube
- BIBD
- Bicentric Polygon
- Bicentric Quadrilateral
- Bichromatic Graph
- Bicollared
- Bi-Connected Component
- Bicorn
- Bicubic Spline
- Bicupola
- Bicuspid Curve
- Bicylinder
- Bidiakis Cube
- Bieberbach Conjecture
- Bienaymé-Chebyshev Inequality
- Bifoliate