Epitrochoid
 |
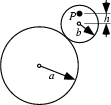The Roulette traced by a point 
attached to a Circle of radius 
rolling around the outside of a fixedCircle of radius 
. These curves were studied by Dürer (1525), Desargues (1640), Huygens 
(1679),Leibniz, Newton (1686), L'Hospital (1690), Jakob Bernoulli (1690), la Hire (1694), Johann Bernoulli (1695), Daniel Bernoulli (1725), Euler (1745, 1781). An epitrochoid appears in Dürer's work Instruction in Measurement with Compassesand Straight Edge (1525). He called epitrochoids Spider Lines because the lines he used to construct the curves lookedlike a spider.
The parametric equations for an epitrochoid are
 |  |  | |
 | |  |
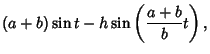where
is the distance from to the center of the rolling Circle. Special cases include the Limaçon with 
, the Circle with 
, and the Epicycloid with 
.See also Epicycloid, Hypotrochoid, Spirograph, Trochoid
References
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 168-170, 1972. Lee, X. ``Epitrochoid.''http://www.best.com/~xah/SpecialPlaneCurves_dir/Epitrochoid_dir/epitrochoid.html. Lee, X. ``Epitrochoid and Hypotrochoid Movie Gallery.''http://www.best.com/~xah/SpecialPlaneCurves_dir/EpiHypoTMovieGallery_dir/epiHypoTMovieGallery.html.
- Thue-Siegel-Roth Theorem
- Thue-Siegel-Schneider-Roth Theorem
- Thue's Theorem
- Thurston's Geometrization Conjecture
- Thwaites Conjecture
- Ticktacktoe
- Tic-Tac-Toe
- Tight Closure
- Tightly Embedded
- Tiling
- Tiling Theorem
- Times
- Titanic Prime
- Titchmarsh Theorem
- Tit-for-Tat
- Tits Group
- Toeplitz Matrix
- Togliatti Surface
- Tomography
- Tooth Surface
- Topological Basis
- Topological Completion
- Topological Dimension
- Topological Entropy
- Topological Groupoid