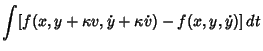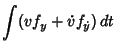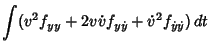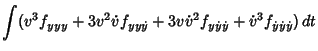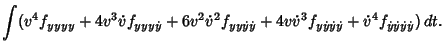| 释义 |
Euler-Lagrange Differential EquationA fundamental equation of Calculus of Variations which states that if 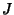 is defined by an Integral of the form is defined by an Integral of the form
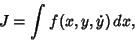 | (1) |
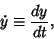 | (2) |
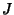 has a Stationary Value if the Euler-Lagrange differential equation has a Stationary Value if the Euler-Lagrange differential equation
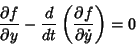 | (3) |
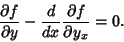 | (4) |
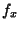 (the Partial Derivative of (the Partial Derivative of  with respect to with respect to  ) turns out to be 0, in which case a manipulation of the Euler-Lagrange differential equation reduces to the greatly simplified andpartially integrated form known as the Beltrami Identity, ) turns out to be 0, in which case a manipulation of the Euler-Lagrange differential equation reduces to the greatly simplified andpartially integrated form known as the Beltrami Identity,
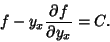 | (5) |
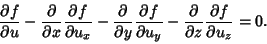 | (6) |
To derive the Euler-Lagrange differential equation, examine
since 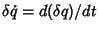 . Now, integrate the second term by Parts using . Now, integrate the second term by Parts using
so
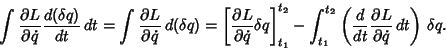 | (10) |
 | (11) |
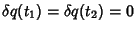 and (11) becomes and (11) becomes
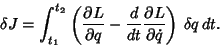 | (12) |
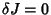 . These must vanish for any smallchange . These must vanish for any smallchange 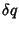 , which gives from (12), , which gives from (12),
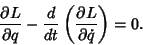 | (13) |
The variation in 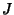 can also be written in terms of the parameter can also be written in terms of the parameter 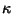 as as
where
and the first, second, etc., variations are
The second variation can be re-expressed using
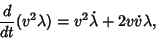 | (21) |
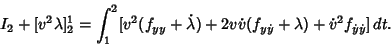 | (22) |
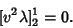 | (23) |
 such that such that
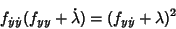 | (24) |
 such that such that
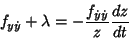 | (25) |
 satisfies satisfies
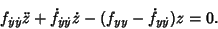 | (26) |
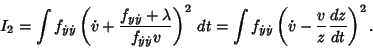 | (27) |
References
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, 1985.Forsyth, A. R. Calculus of Variations. New York: Dover, pp. 17-20 and 29, 1960. Morse, P. M. and Feshbach, H. ``The Variational Integral and the Euler Equations.'' §3.1 in Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 276-280, 1953. |
![]() is defined by an Integral of the form
is defined by an Integral of the form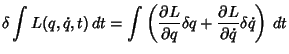
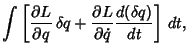
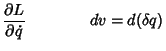
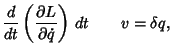
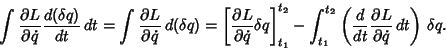

![]() can also be written in terms of the parameter
can also be written in terms of the parameter ![]() as
as