Fontené Theorems
- 1. If the sides of the Pedal Triangle of a point
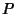 meet the corresponding sides of a Triangle
meet the corresponding sides of a Triangle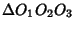 at
at 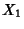 ,
, 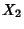 , and
, and  , respectively, then
, respectively, then  ,
, 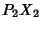 ,
, 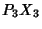 meet at a point
meet at a point  common to the Circles
common to the Circles  and
and  . In other words,
. In other words,  is one of the intersectionsof the Nine-Point Circle of
is one of the intersectionsof the Nine-Point Circle of  and the Pedal Circle of
and the Pedal Circle of 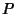 .
. - 2. If a point moves on a fixed line through the Circumcenter, then its Pedal Circle passesthrough a fixed point on the Nine-Point Circle.
- 3. The Pedal Circle of a point is tangent to the Nine-Point Circle Iff the point and itsIsogonal Conjugate lie on a Line through the Orthocenter. Feuerbach's Theorem is a special case of this theorem.
References
Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, pp. 245-247, 1929.
- Mercator Projection
- Mercator Series
- Mercer's Theorem
- Mergelyan-Wesler Theorem
- Meridian
- Meromorphic
- Mersenne Number
- Mersenne Prime
- Mertens Conjecture
- Mertens Constant
- Mertens Function
- Mertens Theorem
- Mertz Apodization Function
- Mesh Size
- Mesokurtic
- M-Estimate
- Metabiaugmented Dodecahedron
- Metabiaugmented Hexagonal Prism
- Metabiaugmented Truncated Dodecahedron
- Metabidiminished Icosahedron
- Metabidiminished Rhombicosidodecahedron
- Metabigyrate Rhombicosidodecahedron
- Metadrome
- Metagyrate Diminished Rhombicosidodecahedron
- Metalogic