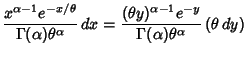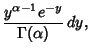| 释义 |
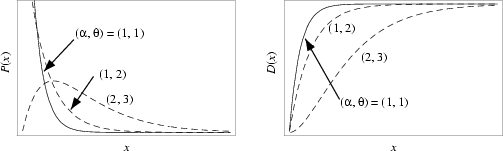
Gamma DistributionA general type of statistical Distribution which is related to the Beta Distribution and arises naturally inprocesses for which the waiting times between Poisson Distributed events are relevant. Gammadistributions have two free parameters, labeled 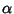 and and  , a few of which are illustrated above. , a few of which are illustrated above.
Given a Poisson Distribution with a rate of change  , the Distribution Function , the Distribution Function  giving thewaiting times until the giving thewaiting times until the 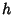 th change is th change is
for 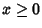 . The probability function . The probability function 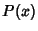 is then obtained by differentiating is then obtained by differentiating 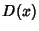 , ,
Now let 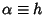 and define and define 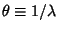 to be the time between changes. Then the above equationcan be written to be the time between changes. Then the above equationcan be written
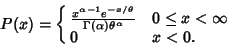 | (3) |
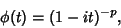 | (4) |
In order to find the Moments of the distribution, let
so
and the logarithmic Moment-Generating function is
The Mean, Variance, Skewness, and Kurtosis are then
The gamma distribution is closely related to other statistical distributions.If 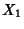 , , 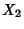 , ..., , ..., 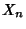 are independent random variates with a gamma distribution having parameters are independent random variates with a gamma distribution having parameters  , , 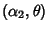 , ..., , ..., 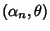 , then , then 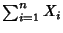 is distributed as gamma withparameters is distributed as gamma withparameters
Also, if 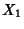 and and 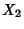 are independent random variates with a gamma distribution having parameters are independent random variates with a gamma distribution having parameters  and and 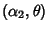 , then , then 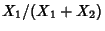 is a Beta Distribution variate with parameters is a Beta Distribution variate with parameters 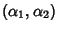 . Both can be derived as follows. . Both can be derived as follows.
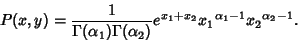 | (18) |
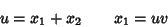 | (19) |
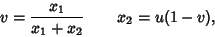 | (20) |
 | (21) |
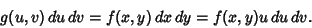 | (22) |
The sum 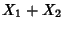 therefore has the distribution therefore has the distribution
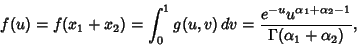 | (24) |
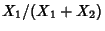 has the distribution has the distribution
where  is the Beta Function, which is a Beta Distribution. is the Beta Function, which is a Beta Distribution.
If  and and  are gamma variates with parameters are gamma variates with parameters 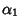 and and 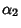 , the , the  is a variate with a BetaPrime Distribution with parameters is a variate with a BetaPrime Distribution with parameters 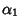 and and 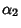 . Let . Let
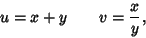 | (26) |
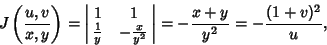 | (27) |
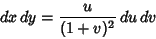 | (28) |
The ratio  therefore has the distribution therefore has the distribution
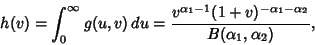 | (30) |
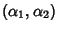 . .
The ``standard form'' of the gamma distribution is given by letting 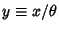 , so , so 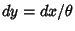 and and
so the Moments about 0 are
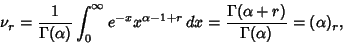 | (32) |
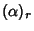 is the Pochhammer Symbol. The Moments about is the Pochhammer Symbol. The Moments about 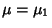 are then are then
The Moment-Generating Function is
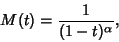 | (37) |
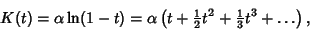 | (38) |
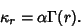 | (39) |
 is a Normal variate with Mean is a Normal variate with Mean 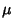 and Standard Deviation and Standard Deviation 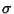 ,then ,then
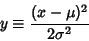 | (40) |
 .See also Beta Distribution, Chi-Squared Distribution .See also Beta Distribution, Chi-Squared Distribution
References
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 534, 1987. |
![]() and
and ![]() , a few of which are illustrated above.
, a few of which are illustrated above.![]() , the Distribution Function
, the Distribution Function ![]() giving thewaiting times until the
giving thewaiting times until the ![]() th change is
th change is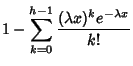

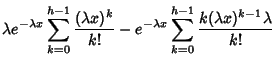
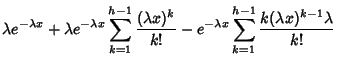
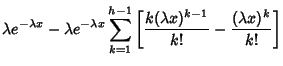
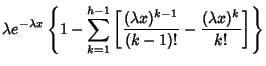
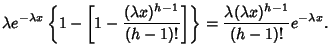
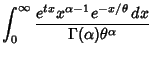
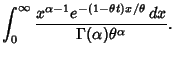
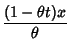
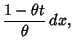
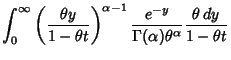
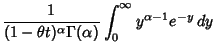
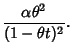
![]() ,
, ![]() , ...,
, ..., ![]() are independent random variates with a gamma distribution having parameters
are independent random variates with a gamma distribution having parameters ![]() ,
, ![]() , ...,
, ..., ![]() , then
, then ![]() is distributed as gamma withparameters
is distributed as gamma withparameters 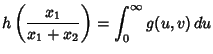
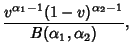
![]() and
and ![]() are gamma variates with parameters
are gamma variates with parameters ![]() and
and ![]() , the
, the ![]() is a variate with a BetaPrime Distribution with parameters
is a variate with a BetaPrime Distribution with parameters ![]() and
and ![]() . Let
. Let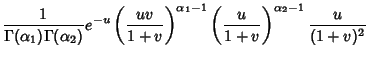
![]() , so
, so ![]() and
and