Hadamard Matrix
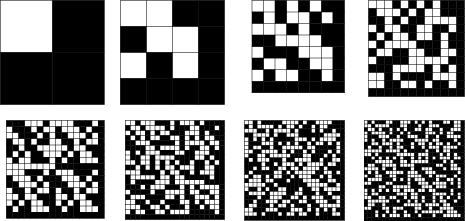
A class of Sylvester ![]() (1867) under the name of Anallagmatic Pavement. AHadamard matrix is a Square Matrix containing only 1s and
(1867) under the name of Anallagmatic Pavement. AHadamard matrix is a Square Matrix containing only 1s and ![]() s such that when any two columns or rows are placed sideby side, Half the adjacent cells are the same Sign and half the other (excepting from the count an
s such that when any two columns or rows are placed sideby side, Half the adjacent cells are the same Sign and half the other (excepting from the count an ![]() -shaped``half-frame'' bordering the matrix on two sides which is composed entirely of 1s). When viewed as pavements, cells with 1sare colored black and those with
-shaped``half-frame'' bordering the matrix on two sides which is composed entirely of 1s). When viewed as pavements, cells with 1sare colored black and those with ![]() s are colored white. Therefore, the
s are colored white. Therefore, the ![]() Hadamard matrix
Hadamard matrix ![]() must have
must have![]() white squares (
white squares (![]() s) and
s) and ![]() black squares (1s).
black squares (1s).
This is equivalent to the definition
| (1) |
Paley's Theorem guarantees that there always exists a Hadamard matrix ![]() when
when ![]() is divisible by 4 and of theform
is divisible by 4 and of theform ![]() , where
, where ![]() is an Odd Prime. In such cases, the Matrices can be constructed using aPaley Construction. The Paley Class
is an Odd Prime. In such cases, the Matrices can be constructed using aPaley Construction. The Paley Class ![]() is undefined for the following values of
is undefined for the following values of ![]() : 92, 116, 156, 172,184, 188, 232, 236, 260, 268, 292, 324, 356, 372, 376, 404, 412, 428, 436, 452, 472, 476, 508, 520, 532, 536, 584, 596, 604,612, 652, 668, 712, 716, 732, 756, 764, 772, 808, 836, 852, 856, 872, 876, 892, 904, 932, 940, 944, 952, 956, 964, 980, 988,996.
: 92, 116, 156, 172,184, 188, 232, 236, 260, 268, 292, 324, 356, 372, 376, 404, 412, 428, 436, 452, 472, 476, 508, 520, 532, 536, 584, 596, 604,612, 652, 668, 712, 716, 732, 756, 764, 772, 808, 836, 852, 856, 872, 876, 892, 904, 932, 940, 944, 952, 956, 964, 980, 988,996.
Sawade (1985) constructed ![]() . It is conjectured (and verified up to
. It is conjectured (and verified up to ![]() ) that
) that ![]() exists forall
exists forall ![]() Divisible by 4 (van Lint and Wilson 1993). However, the proof of this Conjecture remains an importantproblem in Coding Theory. The number of Hadamard matrices of order
Divisible by 4 (van Lint and Wilson 1993). However, the proof of this Conjecture remains an importantproblem in Coding Theory. The number of Hadamard matrices of order ![]() are 1, 1, 1, 5, 3, 60, 487, ...(Sloane's A007299).
are 1, 1, 1, 5, 3, 60, 487, ...(Sloane's A007299).
If ![]() and
and ![]() are known, then
are known, then ![]() can be obtained by replacing all 1s in
can be obtained by replacing all 1s in ![]() by
by![]() and all
and all ![]() s by
s by ![]() . For
. For ![]() , Hadamard matrices with
, Hadamard matrices with ![]() , 20, 28, 36, 44, 52, 60, 68, 76,84, 92, and 100 cannot be built up from lower order Hadamard matrices.
, 20, 28, 36, 44, 52, 60, 68, 76,84, 92, and 100 cannot be built up from lower order Hadamard matrices.
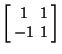 | (2) | ||
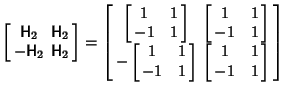 | |||
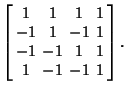 | (3) |
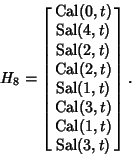 | (4) |
Hadamard matrices can be used to make Error-Correcting Codes.
See also Hadamard Design, Paley Construction, Paley's Theorem, Walsh Function
References
Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, pp. 107-109 and 274, 1987. Beth, T.; Jungnickel, D.; and Lenz, H. Design Theory. New York: Cambridge University Press, 1986. Colbourn, C. J. and Dinitz, J. H. (Eds.) ``Hadamard Matrices and Designs.'' Ch. 24 in CRC Handbook of Combinatorial Designs. Boca Raton, FL: CRC Press, pp. 370-377, 1996. Geramita, A. V. Orthogonal Designs: Quadratic Forms and Hadamard Matrices. New York: Marcel Dekker, 1979. Golomb, S. W. and Baumert, L. D. ``The Search for Hadamard Matrices.'' Amer. Math. Monthly 70, 12-17, 1963. Hall, M. Jr. Combinatorial Theory, 2nd ed. New York: Wiley, p. 207, 1986. Hedayat, A. and Wallis, W. D. ``Hadamard Matrices and Their Applications.'' Ann. Stat. 6, 1184-1238, 1978. Kimura, H. ``Classification of Hadamard Matrices of Order 28.'' Disc. Math. 133, 171-180, 1994. Kimura, H. ``Classification of Hadamard Matrices of Order 28 with Hall Sets.'' Disc. Math. 128, 257-269, 1994. Ogilvie, G. A. ``Solution to Problem 2511.'' Math. Questions and Solutions 10, 74-76, 1868. Paley, R. E. A. C. ``On Orthogonal Matrices.'' J. Math. Phys. 12, 311-320, 1933. Ryser, H. J. Combinatorial Mathematics. Buffalo, NY: Math. Assoc. Amer., pp. 104-122, 1963. Sawade, K. ``A Hadamard Matrix of Order-268.'' Graphs Combinatorics 1, 185-187, 1985. Seberry, J. and Yamada, M. ``Hadamard Matrices, Sequences, and Block Designs.'' Ch. 11 in Contemporary Design Theory: A Collection of Surveys (Eds. J. H. Dinitz and D. R. Stinson). New York: Wiley, pp. 431-560, 1992. Sloane, N. J. A. SequenceA007299/M3736in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995. Spence, E. ``Classification of Hadamard Matrices of Order 24 and 28.'' Disc. Math 140, 185-243, 1995. Sylvester, J. J. ``Thoughts on Orthogonal Matrices, Simultaneous Sign-Successions, and Tessellated Pavements in Two or More Colours, with Applications to Newton's Rule, Ornamental Tile-Work, and the Theory of Numbers.'' Phil. Mag. 34, 461-475, 1867. Sylvester, J. J. ``Problem 2511.'' Math. Questions and Solutions 10, 74, 1868. van Lint, J. H. and Wilson, R. M. A Course in Combinatorics. New York: Cambridge University Press, 1993.![]() Kitis, L. ``Paley's Construction of Hadamard Matrices.''http://www.mathsource.com/cgi-bin/MathSource/Applications/Mathematics/0205-760.
Kitis, L. ``Paley's Construction of Hadamard Matrices.''http://www.mathsource.com/cgi-bin/MathSource/Applications/Mathematics/0205-760.
- Wilson's Theorem
- Wilson's Theorem Corollary
- Wilson's Theorem (Gauss's Generalization)
- Winding Number (Contour)
- Winding Number (Map)
- Windmill
- Window Function
- Winkler Conditions
- Winograd Transform
- Wirtinger's Inequality
- Wirtinger-Sobolev Isoperimetric Constants
- Witch of Agnesi
- Witness
- Wittenbauer's Parallelogram
- Wittenbauer's Theorem
- Witten's Equations
- Wolstenholme's Theorem
- Woodall Number
- Woodbury Formula
- Word
- World Line
- Worm
- Worpitzky's Identity
- Writhe
- Wronskian