| 释义 |
Hurwitz's Irrational Number TheoremAs Lagrange  showed, any Irrational Number showed, any Irrational Number 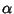 has an infinity of rational approximations has an infinity of rational approximations  which satisfy which satisfy
 | (1) |
 , ,
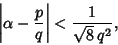 | (2) |
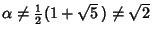 , ,
 | (3) |
 | (4) |
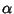 , where the , where the 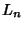 are called Lagrange Numbers and get steadily larger for each ``bad'' set of irrational numbers which is excluded.See also Hurwitz's Irrational Number Theorem, Liouville's Rational ApproximationTheorem, Liouville-Roth Constant, Markov Number, Roth's Theorem, Segre's Theorem,Thue-Siegel-Roth Theorem are called Lagrange Numbers and get steadily larger for each ``bad'' set of irrational numbers which is excluded.See also Hurwitz's Irrational Number Theorem, Liouville's Rational ApproximationTheorem, Liouville-Roth Constant, Markov Number, Roth's Theorem, Segre's Theorem,Thue-Siegel-Roth Theorem
References
Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, p. 40, 1987.Chandrasekharan, K. An Introduction to Analytic Number Theory. Berlin: Springer-Verlag, p. 23, 1968. Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 187-189, 1996.
|
![]() showed, any Irrational Number
showed, any Irrational Number ![]() has an infinity of rational approximations
has an infinity of rational approximations ![]() which satisfy
which satisfy