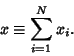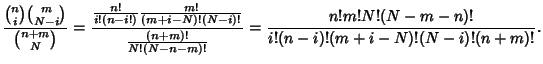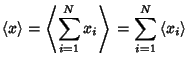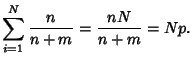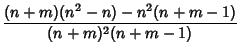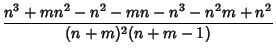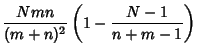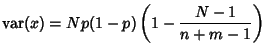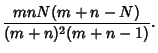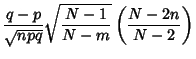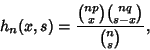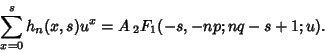| 释义 |
Hypergeometric DistributionLet there be  ways for a successful and ways for a successful and  ways for an unsuccessful trial out of a total of ways for an unsuccessful trial out of a total of  possibilities.Take possibilities.Take 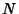 samples and let samples and let 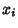 equal 1 if selection equal 1 if selection  is successful and 0 if it is not. Let is successful and 0 if it is not. Let  be the total number ofsuccessful selections, be the total number ofsuccessful selections,
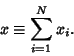 | (1) |
 successful selections is then successful selections is then
The  th selection has an equal likelihood of being in any trial, so the fraction of acceptable selections th selection has an equal likelihood of being in any trial, so the fraction of acceptable selections  is is
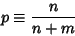 | (3) |
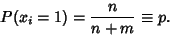 | (4) |
 is is
The Variance is
 | (6) |
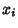 is a Bernoulli variable, is a Bernoulli variable,
so
 | (8) |
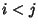 , the Covariance is , the Covariance is
 | (9) |
 and and  are successful for are successful for  is is
But since 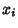 and and  are random Bernoulli variables (each 0 or 1), their product isalso a Bernoulli variable. In order for are random Bernoulli variables (each 0 or 1), their product isalso a Bernoulli variable. In order for 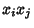 to be 1, both to be 1, both 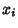 and and  must be1, must be1,
Combining (11) with
 | (12) |
There are a total of 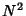 terms in a double summation over terms in a double summation over 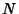 . However, . However,  for for 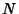 of these, so there are a totalof of these, so there are a totalof 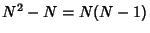 terms in the Covariance summation terms in the Covariance summation
 | (14) |
so the final result is
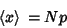 | (16) |
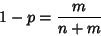 | (17) |
 | (18) |
The Skewness is
and the Kurtosis
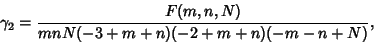 | (21) |
The Generating Function is
 | (23) |
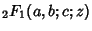 is the Hypergeometric Function. is the Hypergeometric Function.
If the hypergeometric distribution is written
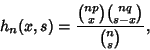 | (24) |
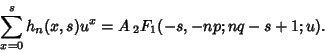 | (25) |
References
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, pp. 532-533, 1987.Spiegel, M. R. Theory and Problems of Probability and Statistics. New York: McGraw-Hill, pp. 113-114, 1992. |
![]() ways for a successful and
ways for a successful and ![]() ways for an unsuccessful trial out of a total of
ways for an unsuccessful trial out of a total of ![]() possibilities.Take
possibilities.Take ![]() samples and let
samples and let ![]() equal 1 if selection
equal 1 if selection ![]() is successful and 0 if it is not. Let
is successful and 0 if it is not. Let ![]() be the total number ofsuccessful selections,
be the total number ofsuccessful selections,