dy

––

dx

df

––

dx

differential calculus 133

h

f(x)f′(x)

k(constant) 0

mx+b(straight line of slope m)m

x1

xrrxr–1

sin xcos x

cos x–sin x

1

tan xsec2x= ——

–

cos2x

sec xsec xtan x

cosec x–cosec xcot x

cot x–cosec 2x

exex

ekx kekx

axaxln a

sinh xcosh x

cosh xsinh x

1

ln x

–

x

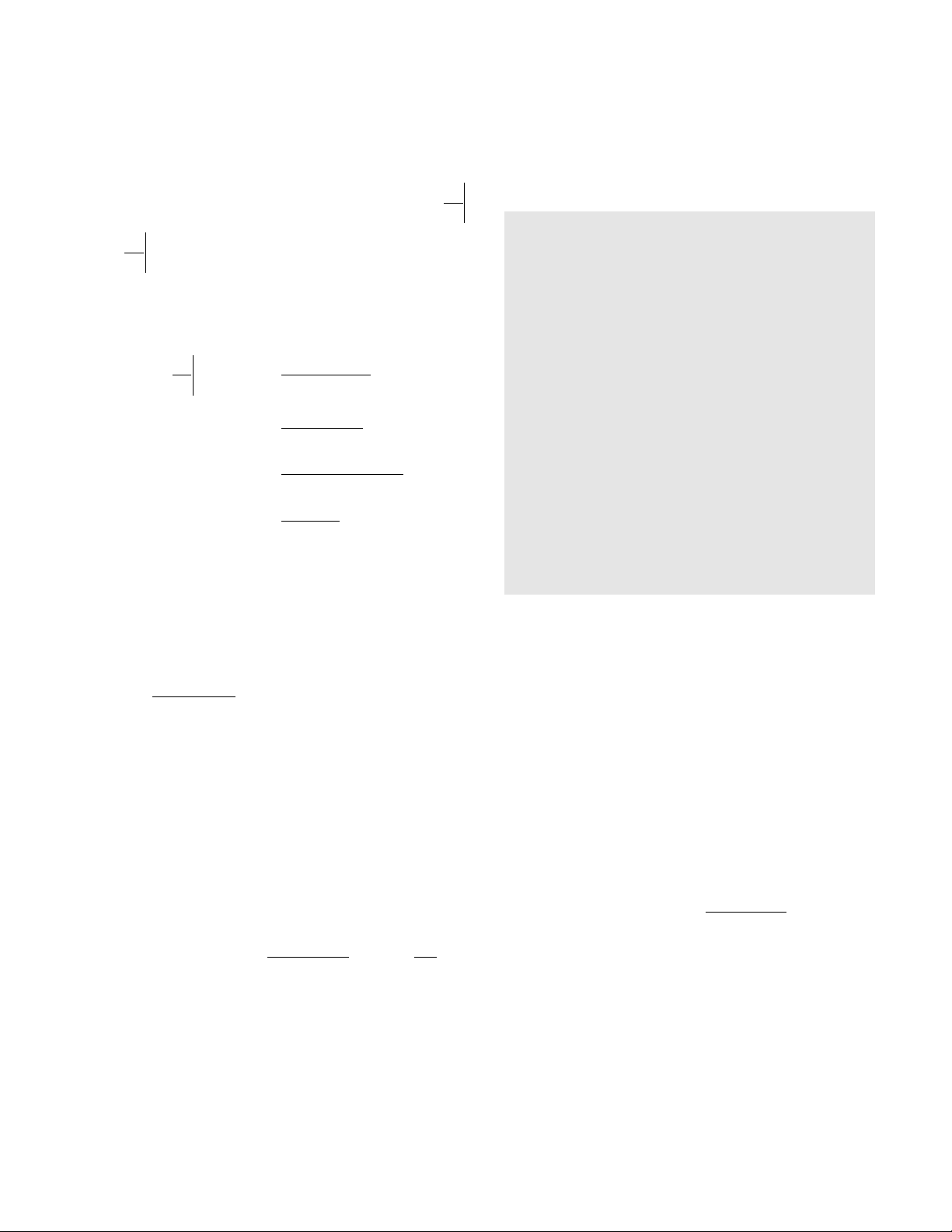
The derivative of a function y = f(x) at position xis

denoted either or (or, to make the point at

which the derivative is being computed explicit,

or ). The derivative is also written f′(x), which is

read as “fprime of x.”

As an example, the derivative of the function

y=f(x)=x2at position x= 7 is given by:

That is, the slope of the tangent line to the curve

y=x2at x=7 is 14. In general, the derivative of

f(x)=x2at an arbitrary point xis given by:

.A consider-

able amount of algebra is usually needed to compute

these limits. The aim is to cancel hin the denomina-

tor so as to avoid division by zero.

The process of finding the derivative of a function

is called differentiation. A function y = f(x) is called dif-

ferentiable at a point xif the derivative of the function

f′(x) exists at that position. A function is differentiable

if its derivative can be computed at every point under

consideration. Not every function is differentiable.

For example, the

ABSOLUTE VALUE

function y= |x|

has no well-defined tangent line at its vertex at position

x= 0, and limit does

not exist. (Consider the cases hpositive and negative sep-

arately.) It can be shown that every differentiable func-

tion is continuous, but, as the absolute-value function

shows, a continuous function need not be differentiable.

The thrust of differential calculus is thus the com-

putation of the derivatives of functions. The following

table shows the derivatives of some standard functions.

The

PRODUCT RULE

,

QUOTIENT RULE

, and the

CHAIN

RULE

also assist in the computation of derivatives.

Apart from dealing with issues of rates of change,

differential calculus is also used to solve

OPTIMIZATION

problems, that is, problems of finding the maximum or

minimum values for a given function (which are called

MAXIMUM

/

MINIMUM

problems).

A

NTIDIFFERENTIATION

is intimately connected with

INTEGRAL CALCULUS

, the general problem of computing

areas under curves and volumes under surfaces. The

FUNDAMENTAL THEOREM OF CALCULUS

explains this

connection.

The derivative of a function y = f(x) at the point

x=x1can alternatively be defined as the limit:

Some authors of mathematics textbooks prefer this def-

inition. Of course, setting x1=xand x2=x+h, it is

equivalent to the definition presented above.

See also

CONCAVE UP

/

CONCAVE DOWN

;

DIFFEREN

-

TIAL

;

DIFFERENTIAL EQUATION

;

DIRECTIONAL DERIVA

-

TIVE

;

HIGHER DERIVATIVE

;

HISTORY OF CALCULUS

(essay);

IMPLICIT DIFFERENTIATION

;

INCREASING

/