单词	irreducible
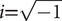释义	irreducible Unable to be factorized. So prime numbers are irreducible integers, and x2 + 1 is irreducible when considered as a real polynomial though it is reducible to (x + i) (x−i) as a complex polynomial where . More generally, an element x of an integral domain R is irreducible if x is non-zero, not a unit, and whenever x = yz where y, z ∈ R, then y or z is a unit. In a unique factorization domain an element is irreducible if and only if it is a prime element, but this is not the case generally in rings.
随便看	NRICH nth derivative nth-order partial derivative nth root nth root of unity n-tuple null hypothesis nullity null matrix null measure null sequence null set null set null space Nullstellensatz null vector number number field number line Numberphile number systems number theory numeral numerator numerical analysis

数学辞典收录了4151条数学词条，基本涵盖了常用数学知识及数学英语单词词组的翻译及用法，是数学学习的有利工具。