left and right derivative
exists, this limit is the left derivative of f at a. Similarly, if
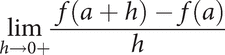
exists, this limit is the right derivative of f at a. (See limit from the left and right.) The derivative f′(a) exists if and only if the left derivative and the right derivative of f at a exist and are equal. An example where the left and right derivatives both exist but are not equal is provided by the function f, where f(x) = |x| for all x. At 0, the left derivative equals−1 and the right derivative equals+1.
- neighbourhood
- neighbourhood
- nested multiplication
- nested sets
- net
- net
- net
- network
- network flow
- Neumann condition
- Neumann, John Von
- neural nets
- neutral element
- neutral geometry
- newton
- Newton, Isaac
- Newton quotient
- Newton's identities
- Newton's interpolating polynomial
- Newton's interpolation formula
- Newton's law of cooling
- Newton's law of gravitation
- Newton's law of restitution
- Newton's laws of motion
- Newton's method